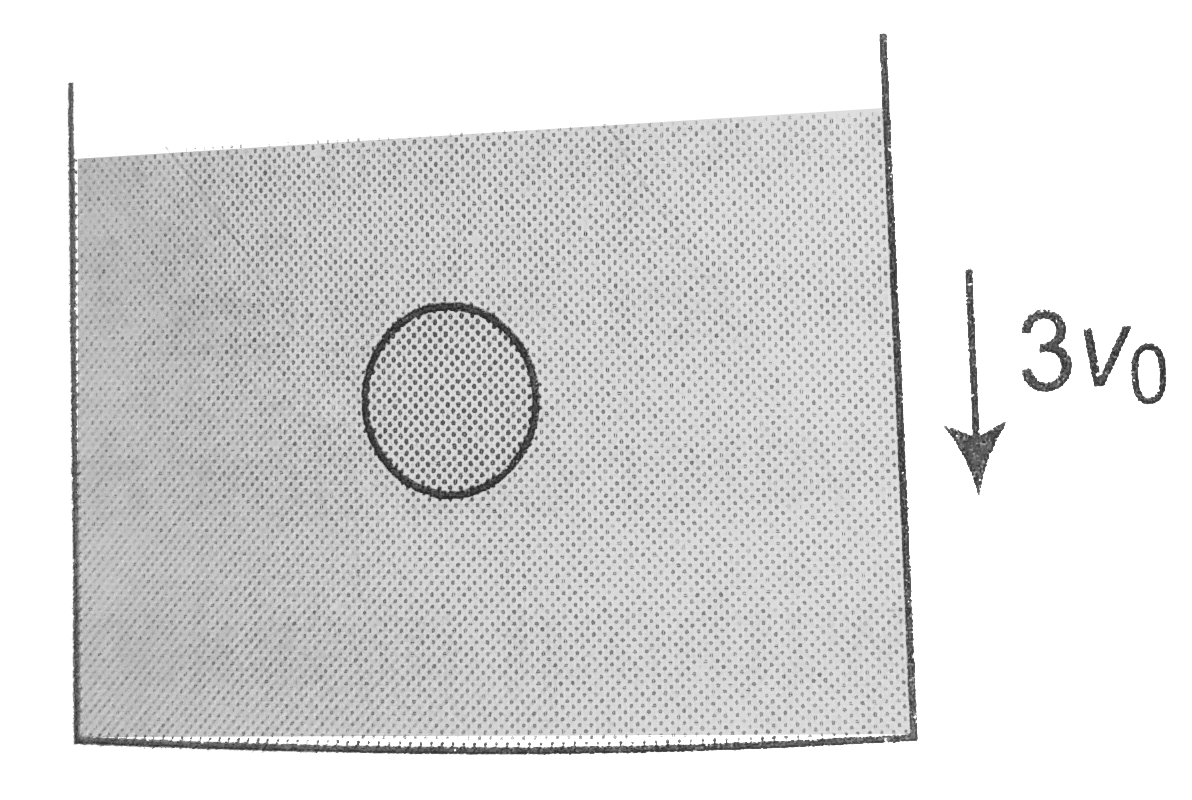
A container filled with viscous liquid is moving vertically downwards with constant speed $3 v_{0}$ . At the instant shown, a sphere of radius $r$ is moving vertically downwards (in liquid) has speed $v_{0}$ . The coefficient of viscosity is $η$ . There is no relative motion between the liquid and the container. Then at the shoen instant, The magnitude of viscous force acting on sphere is

$6 π η r v_{0}$

$12 π η r v_{0}$

$18 π η r v_{0}$

$24 π η r v_{0}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

A rain drop radius $0.3 m m$ falling vertically downwards in air has a terminal velocity of $1 \frac{m}{s}$ The viscosity of air is $18 \times 10^{- 5}$ poise. The viscous force on the drop is
A $101.73 \times 10^{- 4} d e n e$
B $101.73 \times 10^{- 5} d y n e$
C $16.95 \times 10^{- 5} d e n e$
D $16.95 \times 10^{- 4} d y n e$
A sphere of mass m and radius r is projected in a gravity free space with speed v. If coefficient of viscosity of the medium in which it moves is $\frac{1}{6 π}$ , the distance travelled by the body before it stops is
A $\frac{m v}{2 r}$
B $\frac{2 m v}{r}$
C $\frac{m v}{r}$
D $\frac{m v}{4 r}$
A small metal sphere of radius a is falling with a velocity $υ$ through a vertical column of a viscous liquid. If the coefficient of viscosity of the liquid is $η$ , then the sphere encounters an opposing force of
A $6 π η a^{2} υ$
B $\frac{6 η υ}{π a}$
C $6 π η a υ$
D $\frac{π η υ}{6 a^{3}}$