Objective function of a L.P.P. is Solution in Tamil
a constraint
a function to be optimized
a relation between the variables
None of these
The correct Answer is:B
Step by step video, text & image solution for Objective function of a L.P.P. is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LINEAR PROGRAMMING BOOK - NEW JOYTHI PUBLICATIONCHAPTER - LINEAR PROGRAMMING EXERCISE - UNIT TEST
6 Videos
- LINEAR INEQUALITIESBOOK - NEW JOYTHI PUBLICATIONCHAPTER - LINEAR INEQUALITIESEXERCISE - QUESTIONS FROM COMPETITIVE EXAMS
38 Videos
- MATHEMATICAL REASONINGBOOK - NEW JOYTHI PUBLICATIONCHAPTER - MATHEMATICAL REASONINGEXERCISE - QUESTION FROM COMPETITIVE EXAMS
23 Videos
Similar Questions
A company combines two items A and B from gift packs during a festival season. Each pack must weight atleast 5 kg and should contain atelast 2 kg of A and not more than 4 kg of B. The net contribution of the company is Rs. 10 per kg of A and Rs 12 per kg of B. the company wants to determine the optimum mix. By defining suitable variables, write
i. the objective function
ii. The constraints
View SolutionThe set of all x satisying the inequility 4x−13x+1≥1 is
View SolutionThe statement ~(p→q) is equivalent to
View SolutionL.P.P. is a process of finding
View SolutionOptimization of the objective function is a process of
View SolutionL.P.P. has constraints of
View SolutionDefine the term ''objective function'' in an L.P.P.
View SolutionDefine an objective function.
View SolutionThe objective function of a L.P.P is
View SolutionThe feasible region of an L.P.P is shown shaded in the figure. Let Z=3x−4 y be the objective function. The minimum of Z occurs at
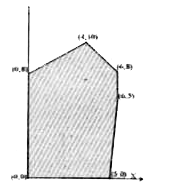
View SolutionObjective function of a LPP is …………
View SolutionIf the objective function for a L.P.P. is Z=5x+7y and the corner points of the bounded feasible region are (0, 0), (7, 0), (3, 4) and (0, 2), then the maximum value of Z occurs at
View SolutionIf the objective function for an L.P.P. is Z=3x−4y and the corner points for the bounded feasible region are (0, 0), (5, 0), (6, 5), (6, 8), (4, 10) and (0, 8), then the maximum value of Z occurs at
View SolutionIf the objective function for an L.P.P. is Z=3x+4y and the corner points for unbounded feasible region are (9, 0), (4, 3), (2, 5), and (0, 8), then the maximum value of Z occurs at
View SolutionIf the corner points of the feasible region for an L.P.P. are (0, 2), (3, 0), (6, 0), (6, 8) and (0, 5), then the minimum value of the objective function F=4x+6y occurs at
View Solution
