In a zoo, the total number of rabbits and peacocks is 50 and the total number of their legs is 140. Find the number of rabbits and number of peacocks.
The correct Answer is:20 and 30
To solve the problem, we need to set up a system of equations based on the information given. Let's denote:
- r = number of rabbits
- p = number of peacocks
Step 1: Set up the equations
From the problem, we have two key pieces of information:
1. The total number of rabbits and peacocks is 50:
r+p=50(Equation 1)
2. The total number of legs is 140. Since each rabbit has 4 legs and each peacock has 2 legs, we can express this as:
4r+2p=140(Equation 2)
Step 2: Simplify Equation 2
To make calculations easier, we can simplify Equation 2 by dividing all terms by 2:
2r+p=70(Equation 3)
Step 3: Solve the system of equations
Now we have two equations to work with:
1. r+p=50 (Equation 1)
2. 2r+p=70 (Equation 3)
We can subtract Equation 1 from Equation 3 to eliminate p:
(2r+p)−(r+p)=70−50
This simplifies to:
2r−r=20
r=20
Step 4: Find the number of peacocks
Now that we have the number of rabbits, we can substitute r=20 back into Equation 1 to find p:
20+p=50
Subtracting 20 from both sides gives:
p=30
Conclusion
Thus, the number of rabbits is 20 and the number of peacocks is 30.
Summary of the solution:
- Number of rabbits r=20
- Number of peacocks p=30
- p = number of peacocks
From the problem, we have two key pieces of information:
r+p=50(Equation 1)
4r+2p=140(Equation 2)
To make calculations easier, we can simplify Equation 2 by dividing all terms by 2:
2r+p=70(Equation 3)
Now we have two equations to work with:
1. r+p=50 (Equation 1)
2. 2r+p=70 (Equation 3)
(2r+p)−(r+p)=70−50
This simplifies to:
2r−r=20
r=20
Now that we have the number of rabbits, we can substitute r=20 back into Equation 1 to find p:
20+p=50
Subtracting 20 from both sides gives:
p=30
Thus, the number of rabbits is 20 and the number of peacocks is 30.
- Number of rabbits r=20
- Number of peacocks p=30
Topper's Solved these Questions
- LINEAR EQUATIONS AND INEQUATIONS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS AND INEQUATIONS EXERCISE - ESSAY TYPE QUESTIONS
5 Videos
- LINEAR EQUATIONS AND INEQUATIONS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS AND INEQUATIONS EXERCISE - LEVEL 1
34 Videos
- LINEAR EQUATIONS AND INEQUATIONS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS AND INEQUATIONS EXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
30 Videos
- INDICESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - INDICESEXERCISE - CONCEPT APPLICATION (Level 3)
7 Videos
- MATRICES BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MATRICES EXERCISE - Concept Application Level -2
15 Videos
Similar Questions
Knowledge Check
Find the total number of factors of 1420 ?
A12B13C14D15Find the total number of factors of 1680.
A40B50C60D30Find the total number of factors of 240 -
A20B25C15D22
In a school, the number of girls is 50 more than the number of boy The total number of students is 1070. Find the number of girls.
View SolutionThere are some parrots and some tigers in a forest. If the total number of animal heads in the forest is 858 and the total number of animal legs is 1846, what is the number of parrots in the forest? (a) 800 (b) 833 (c) 845 (d) Cannot be determined (e) None of these
View SolutionFind the total number of factors of 1680.
View SolutionFind the total number of factors of 540.
View Solution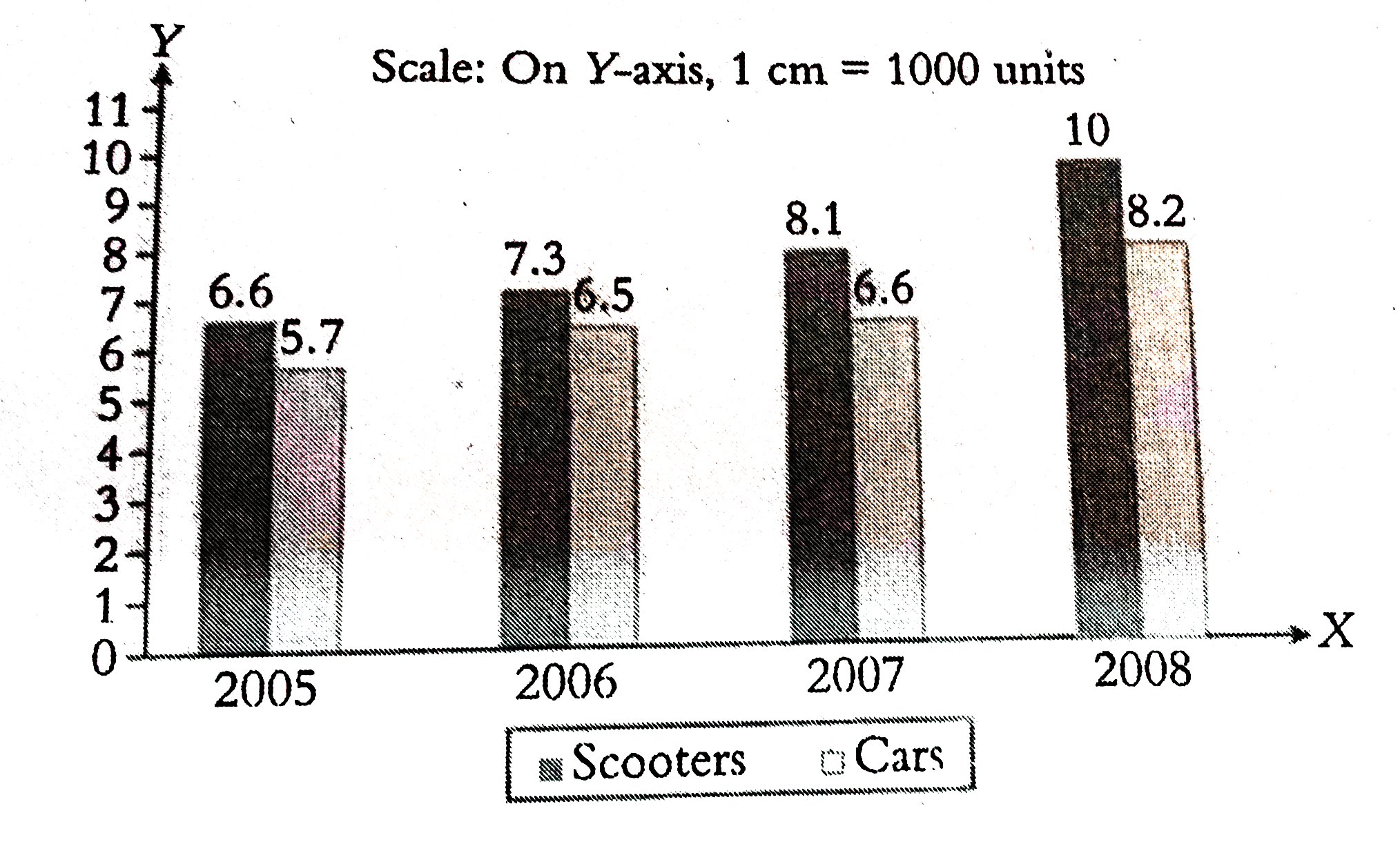
Find the total number of scooters sold in the four years.
View Solution
