If (1001)(ab+cb−cd)=(4−532), then (a-b) + (c-d)=____.
-2
9
2
-1
The correct Answer is:D
To solve the equation given by the matrix multiplication, we need to break it down step by step.
Given:
(1001)(ab+cb−cd)=(4−532)
Step 1: Perform the Matrix Multiplication
The multiplication of the two matrices on the left side is done as follows:
(1⋅a+0⋅(b−c)1⋅(b+c)+0⋅d0⋅a+1⋅(b−c)0⋅(b+c)+1⋅d)=(ab+cb−cd)
So, we have:
(ab+cb−cd)=(4−532)
Step 2: Set Up the Equations
From the equality of the matrices, we can set up the following equations:
1. a=4
2. b+c=−5
3. b−c=3
4. d=2
Step 3: Solve for b and c
We have two equations involving b and c:
1. b+c=−5 (Equation 1)
2. b−c=3 (Equation 2)
To solve these equations, we can add Equation 1 and Equation 2:
(b+c)+(b−c)=−5+3
2b=−2⟹b=−1
Now, substitute b=−1 back into Equation 1 to find c:
−1+c=−5⟹c=−5+1=−4
Step 4: Find a−b+c−d
Now we have:
- a=4
- b=−1
- c=−4
- d=2
Now we calculate (a−b)+(c−d):
a−b+c−d=4−(−1)+(−4)−2
=4+1−4−2
=5−6=−1
Final Answer
Thus, the value of (a−b)+(c−d) is:
−1
(1001)(ab+cb−cd)=(4−532)
(ab+cb−cd)=(4−532)
2. b+c=−5
3. b−c=3
4. d=2
2. b−c=3 (Equation 2)
2b=−2⟹b=−1
- a=4
- b=−1
- c=−4
- d=2
=4+1−4−2
=5−6=−1
−1
Topper's Solved these Questions
- MATRICESBook:PEARSON IIT JEE FOUNDATIONChapter:MATRICESExercise:EXERCISE CONCEPT APPLICATION (LEVEL 2)
Explore 19 Videos
- MATRICESBook:PEARSON IIT JEE FOUNDATIONChapter:MATRICESExercise:EXERCISE CONCEPT APPLICATION (LEVEL 3)
Explore 15 Videos
- MATRICESBook:PEARSON IIT JEE FOUNDATIONChapter:MATRICESExercise:ESSAY TYPE QUESTIONS
Explore 5 Videos
- MATHEMATICAL INDUCTION AND BIONOMIAL THEOREMBook:PEARSON IIT JEE FOUNDATIONChapter:MATHEMATICAL INDUCTION AND BIONOMIAL THEOREMExercise:LEVEL 3
Explore 15 Videos
- MENSURATIONBook:PEARSON IIT JEE FOUNDATIONChapter:MENSURATIONExercise:Level 3
Explore 21 Videos
Similar Questions
Knowledge Check
Match the following:
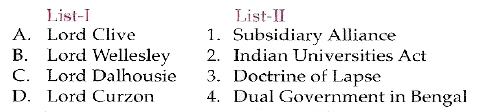
(a) ABCD2341 (b) ABCD4132
(c) ABCD4321 (d) ABCD1423AABCD2341BABCD4132CABCD4321DABCD1423If A20=[abcd] where A=[1102] then a+b+c+d is equal to
A219B220C221D222
If [a−b2a+c2a−b3c+d]=[−15013], then a = __________.
View SolutionFind the values of a,b,c and d, if 3[abcd]=[a6−12d]+[4a+bc+d3].
View SolutionifA=⎡⎢⎣12−35021−11⎤⎥⎦,B=⎡⎢⎣3−12425203⎤⎥⎦andc=⎡⎢⎣4120321−23⎤⎥⎦, then compure (A+B) and (B-C), Also , verify that A+(B-C)=(A+B)-C.
View SolutionIf [2a+ba−2b5c−d4c+3d]=[4−31124] , then value of a + b – c + 2d is:
View SolutionLet A=[1023]andAn=[abcd] then limn→∞b+ca+d =
View SolutionLet A=[01−25−1−4],B=[1−3−10−2 5] and C=[2−51−4 06].
Compute 5A−3B+4C.
View Solution
