Consider the chords of the parabola $y^{2} = 4 x$ which touches the hyperbola $x^{2} - y^{2} = 1$ , the locus of the point of intersection of tangents drawn to the parabola at the extremitites of such chords is a conic section having latursrectum $λ$ , the value of $λ$ , is

$\pm 1$

$\pm 2$

$\pm 3$

$\pm 4$

Video Solution

Text Solution

Verified by Experts

Any tangent to given parabola is
$x . sec θ - y . Tan θ = 1 ... (1)$
Let $P (h, k)$ be the point of intersection of tangents to the parabola $y^{2} = 4 x$ ,
so equation of chord of contact of (h, k) is ky 2(x+h) ...(2)
As, (1) and (2) are identical, so
$\frac{sec θ}{2} = \frac{tan θ}{k} = \frac{- 1}{2 h}$ (on comparing)
$\Rightarrow sec θ = \frac{- 1}{h}$ and $tan θ = \frac{k}{2 h}$
As, ${sec}^{2} θ - {tan}^{2} θ = 1$
$\Rightarrow \frac{1}{h^{2}} - \frac{k^{2}}{4 h^{2}} = 1 \Rightarrow 4 - k^{2} = 4 h^{2}$
$∴$ Locus of P(h,k) is
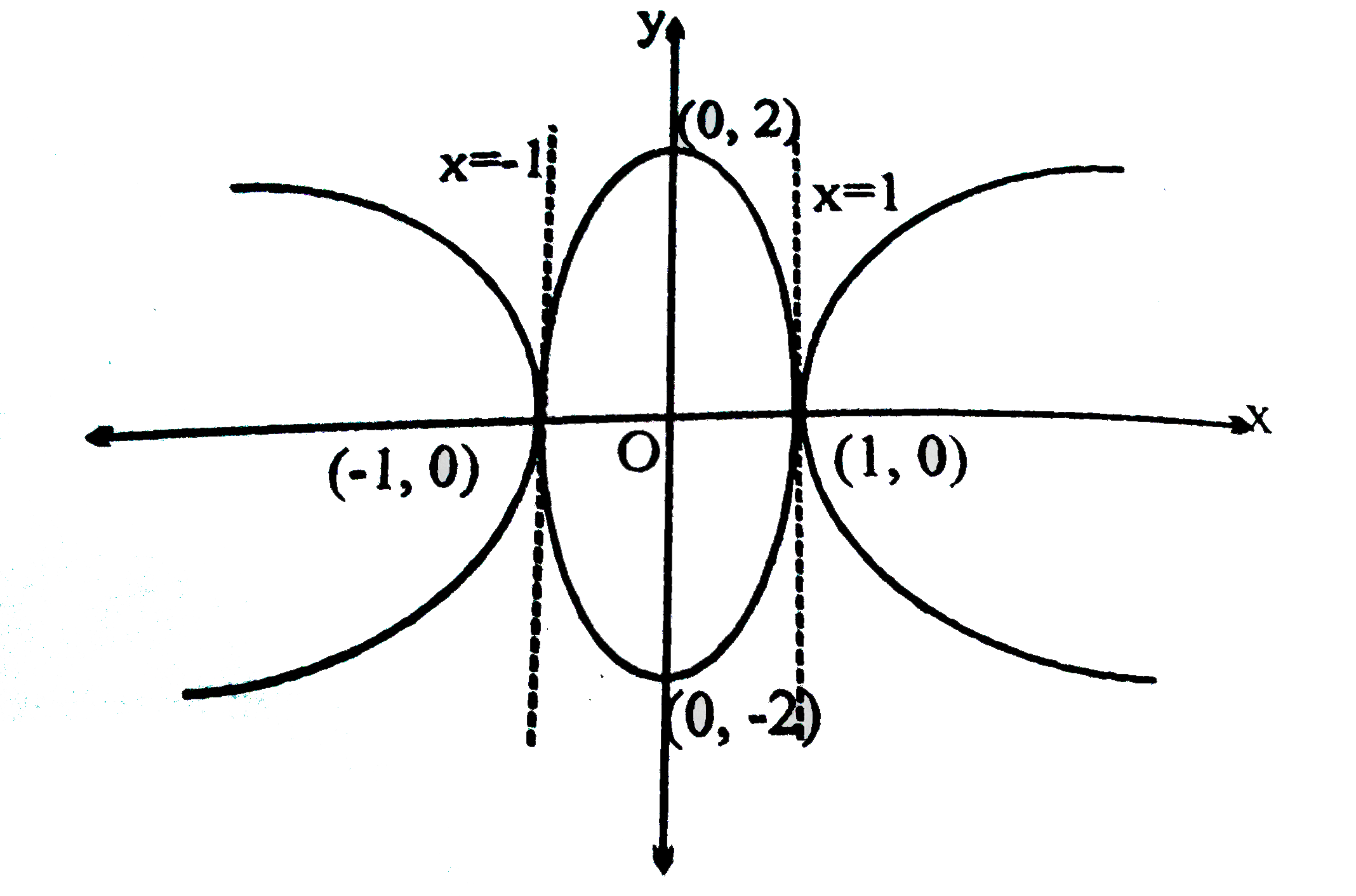
$E : 4 x^{2} + y^{2} = 4$ , where is an ellipse or $\frac{x^{2}}{1} + \frac{y^{2}}{4} = 1 ... (3)$
(i) As , $e^{2} = 1 - \frac{1}{4} = \frac{3}{4} \Rightarrow e = \frac{\sqrt{3}}{2} = {sin 60}^{\circ} \Rightarrow$ (A) is incorrect.
The foci of ellipse are $(0, \pm be) 3 i . e ., (0, \pm \sqrt{3}) \Rightarrow$ (B) is correct.
Also, $l (L . R) = \frac{2 a^{2}}{b} = \frac{2 \times {(1)}^{2}}{2} = 1 \Rightarrow$ (C) is correct.
Note, that distance between vertices of ellipse E = distance between (0,-2) and (0,2)=4 (D) is correct.
(ii) The circle described on vertices of ellipse E as diameter is $x^{2} + y^{2} = 4 ... . (4)$
as, circle in equation (4) intersects orthogonally the circle $x^{2} + y^{2} - 4 x - 2 y + k^{2} = 0$ ,
so using condition of orthogonality, we get $0 = k^{2} - 4 \Rightarrow k = \pm 2$

Updated on:21/07/2023

Chords of the hyperbola $x^{2} - y^{2} = a^{2}$ touch the parabola $y^{2} = 4 a x$ . The locus of their middle point is the curve …………..
A $y^{2} (x - a) = 2 x^{2}$
B $y^{2} (x - a) = x^{3}$
C $y^{2} (x - a) = x^{4}$
D $y^{2} (x + a) = x^{3}$
If the chords of the hyperbola $x^{2} - y^{2} = a^{2}$ touch the parabola $y^{2} = 4 a x$ . Then, the locus of the middle points of these chords is
A $y^{2} = (x - a) x^{3}$
B $y^{2} (x - a) = x^{3}$
C $x^{2} (x - a) = x^{3}$
DNone of these
A chord of the parabola $y^{2} = 4 a x$ subtends a right angle at the vertex. The locus of the point of intersection of tangents at its extremities is
A $x + a = 0$
B $x + 2 a = 0$
C $x + 4 a = 0$
Dnone