Find the minimum distance between the curves $y^{2} = 4 x a n {d x}^{2} + y^{2} - 12 x + 31 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:25

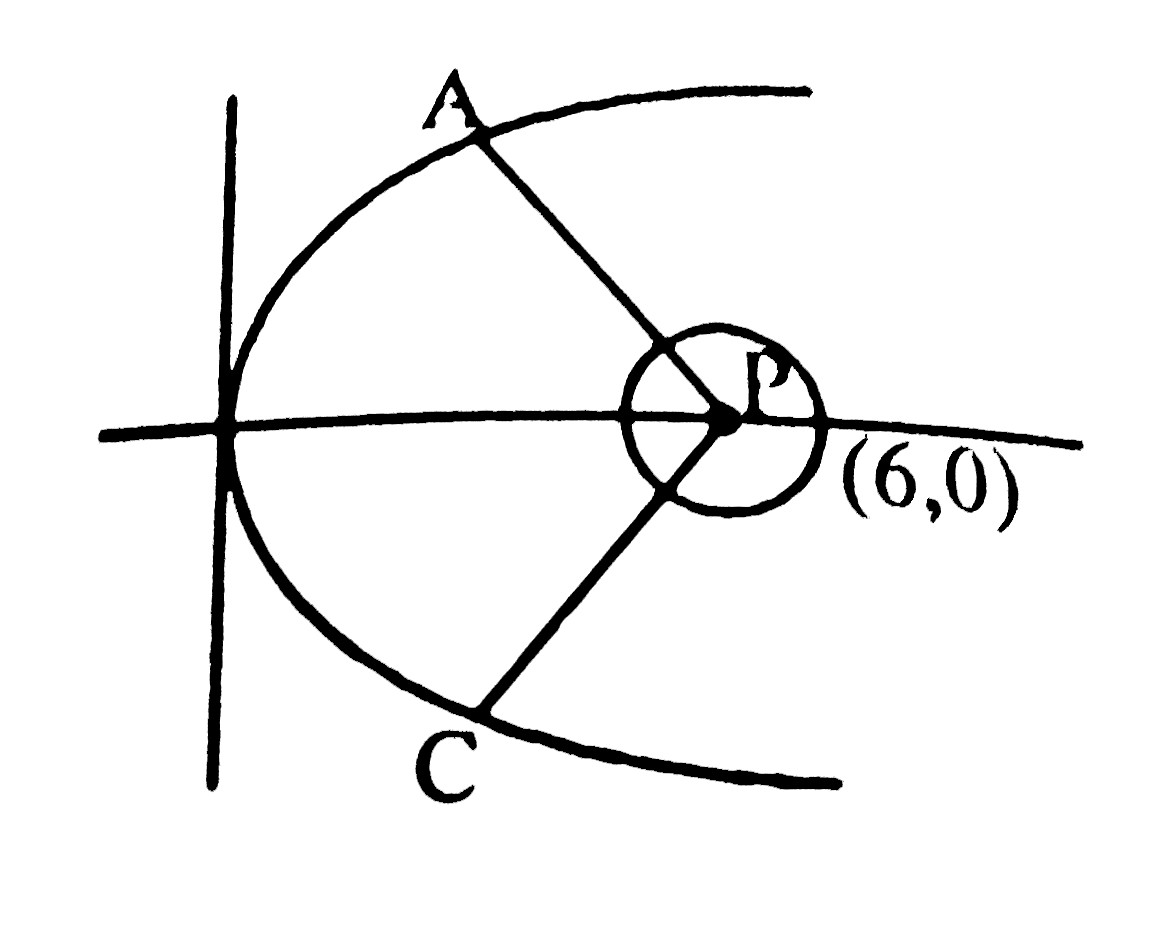
The centre and the radius of the given circle are P(6,0) and $\sqrt{5}$ , respectively. Now, the minimum distance between the two curves always occurs along a line which is normal to both the curves.
The equation of the normal for $y^{2} = 4 x$ at ( $t^{2}, 2 t$ ) is
$y = - t x + 2 t + t^{3}$ .
If it is normal to the circle also, then it must pass though (6,0). So
$0 = t^{3} - 4 t \Rightarrow t = 0$ or $t = \pm 2$
$\Rightarrow A (4, 4)$ and $C (4, 4)$
$\Rightarrow P A = P C = \sqrt{20} = 2 \sqrt{5}$
The required minimum distance is $2 \sqrt{5} - \sqrt{5} = \sqrt{5} = d$
Hence $d^{4} = {(\sqrt{5})}^{4} = 25$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

BANSAL-TEST PAPERS-MATHS

Home
Ask Doubt
Profile