Let 'L' be the point (t,2)and'M' be a point on the y axis such that 'LM' has slope −'t'. Then the locus of the mid point of 'LM',as't' varies over all real values, is a parabola, whose
vertex is (0, 2)
lengths of latus-rectum is 2
focus is (0,178)
equation of directrix is 8y-15=0
The correct Answer is:A, C, D
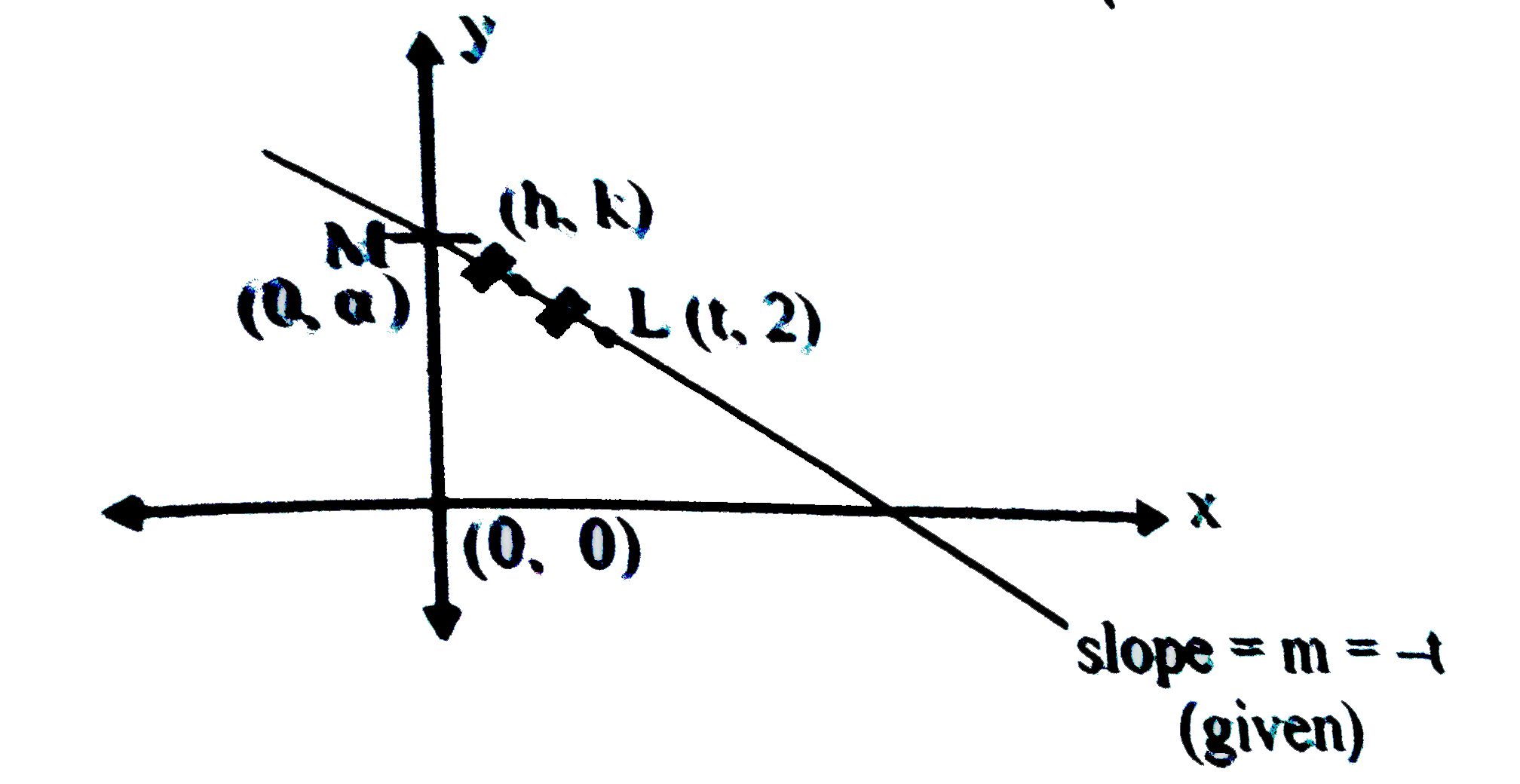
Now, slope of LM=2−αt−0=−t (given)
⇒α−2=t2⇒α=2+t2
Let mid point of LM is (h, k)
Now, 2h=tand2k=α+2=t2+4
∴ On eliminating t, we get
k=2(h2+1)
∴ Locus of (h, k) is y=2(x2+1)⇒x2=12(y−2)
Now, verify alternative.
Topper's Solved these Questions
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:Physics, SECTION-1 (PART-A)
Explore 5 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:Physics, SECTION-1 (PART-A) [COMPREHENSION TYPE]
Explore 2 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:Math
Explore 15 Videos
- PROBABILITYBook:BANSALChapter:PROBABILITYExercise:All Questions
Explore 1 Video
- THERMODYNAMICSBook:BANSALChapter:THERMODYNAMICSExercise:Match the column
Explore 7 Videos
Similar Questions
Knowledge Check
The locus of the mid-point of the chords of the parabola x2=4py having slope m, is a
Acircle with center at origin and radius | 2 pm |Bline parallel to x-axis at a distance | 2 pm | from itCline parallel to y-axis a distance | 2 pm | from itDline parallel to y=mx, m≠0 at a distance | 2 pm | from itThe tangent PT and the normal PN to the parabola y2=4ax at a point P on it meet its axis at points T and N, respectively. The locus of the centroid of the triangle PTN is a parabola whose:
Avertex is (2a3,0)Bdirectrix is x = 0Clatus rectum is 2a3Dfocus is (a,0)Let the tangents to the parabola y2=4ax drawn from point P have slope m1andm2. If m1m2=2, then the locus of point P is
Ax=aBx=a2Cx+a=0Dx=2a
If the mid point of AB = (t, 2t) and the ends A, B move on x and y axes respectively, then the locus of the 1st point of trisection of AB is
View SolutionIf the first point of trisection of AB is (t,2t) and the ends A,B moves on xandy axis respectively, then locus of mid point of AB is
View SolutionIf the first point of trisection of AB is (t,2t) and the ends A, B moves on xandy axis respectively, then locus of mid point of AB is
View SolutionIf the first point of trisection of AB is (t,2t) and the ends A,B moves on xandy axis respectively, then locus of mid point of AB is
View SolutionLet A be a fixed point (0,6) and B be a moving point (2t,0). Let M be the mid-point of AB and the perpendicular bisector of AB meets the y-axis at C. The locus of the mid-point P of MC is :
View Solution