Find the area of the circle $x^{2} + y^{2} = 16 w h i c h i s e x t e r i or \to t h e p a r a b o l a y^{2} = 6 x$ by using integration.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $\frac{4}{3} (8 π - \sqrt{3})$ sq. units

The given curves are
$x^{2} + y^{2} = 16 …(i)$
$y^{2} = 6 x ….(ii)$
$Solving x^{2} + 6 x = 16$
$or (x - 2) (x + 8) = 0$
$∴ x = 2$ (as x=-8 is not possible)
Thus, the point of intersection are $A (2, 2 \sqrt{3}) and A' (2, - 2 \sqrt{3})$ .
The graphs of the curves is as shown in the following figure.

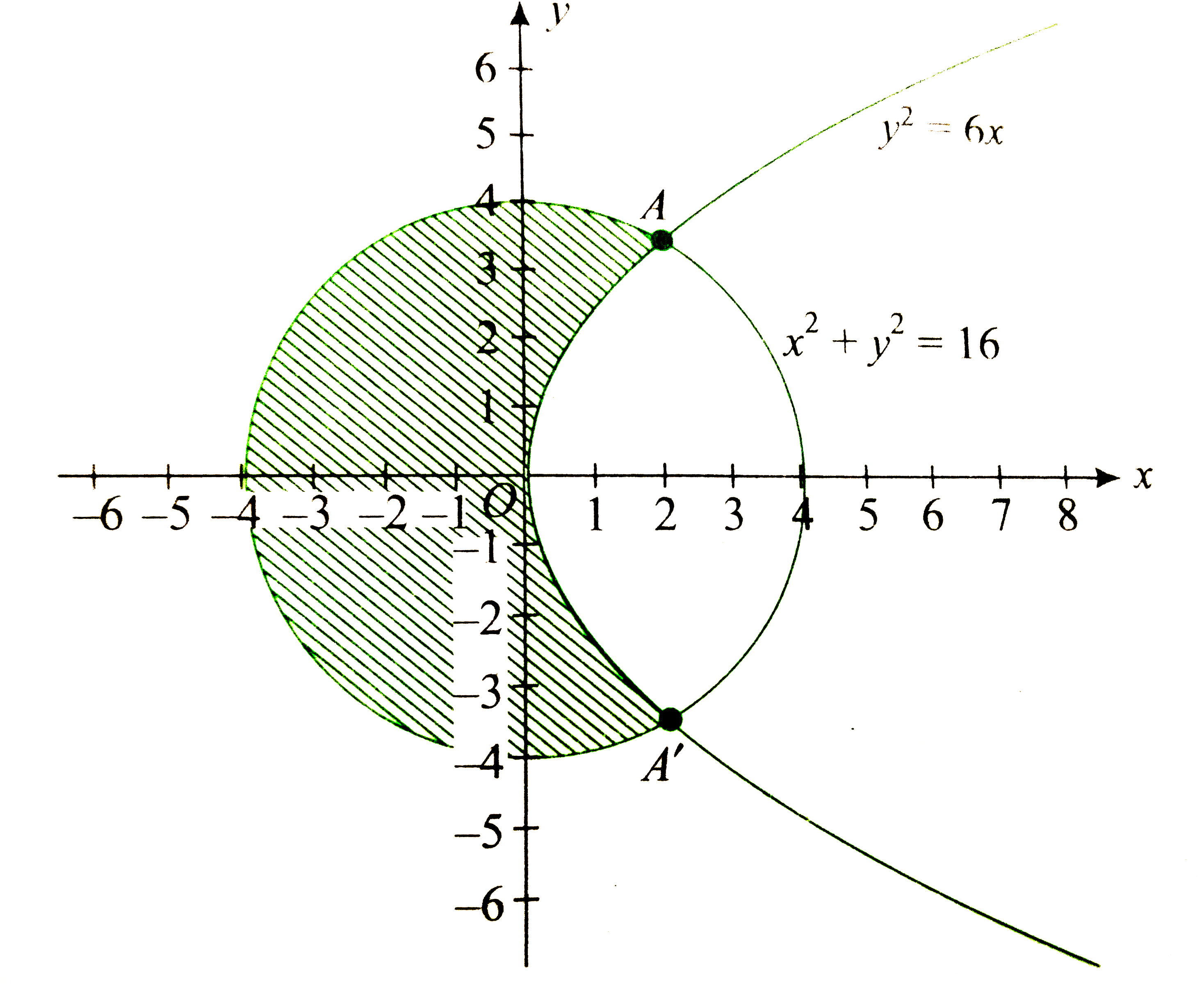
From the figure, required area is
$= Area of semicircle + 2 \overset{2}{\int_{0}} (\sqrt{(16 - x^{2})} - \sqrt{6 x}) d x$
$= 8 π + 2 {[\frac{x}{2} \sqrt{16 - x^{2}} + \frac{16}{2} \sin^{- 1} \frac{x}{4} - \sqrt{6} \frac{2 x^{3 / 2}}{3}]}_{0}^{2}$
$= 8 π + 2 [2 \sqrt{2} + \frac{4 π}{3} - \sqrt{6} \frac{2 \cdot 2^{3 / 2}}{3}]$
$= \frac{4}{3} (8 π - \sqrt{3})$ sq. units

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The area of the portion of the circle $x^{2} + y^{2} = 64$ which is exterior to the parabola $y^{2} = 12 x$ is
A $(8 π - \sqrt{3}) s q u n i t s$
B $\frac{16}{3}) (8 - \sqrt{3}) s q u n i t s$
C $\frac{16}{3}) (8 π - \sqrt{3}) s q u n i t s$
Dnone of the above
Question 2 - Select One
The area of the portion of the circle $x^{2} + y^{2} = 64$ which is exterior to the parabola $y^{2} = 12 x$ is
A $(8 π - \sqrt{3}) s q u n i t s$
B $\frac{16}{3}) (8 - \sqrt{3}) s q u n i t s$
C $\frac{16}{3}) (8 π - \sqrt{3}) s q u n i t s$
Dnone of the above
Question 3 - Select One
Find the axis of the parabola $x^{2} = 16 y$ .
A $x = 0$
B $y = 0$
C $x = 4$
D $x + y = 0$

CENGAGE-AREA-Exercise 9.2

Home
Ask Doubt
Profile