A hot liquid kept in a breaker cools from 80∘C to 70∘C in two minutes. If the surrounding temperature is 30∘C, then the time of cooling of the same liquid from 60∘C to 50∘C is : Solution in Kannada
240 s
480 s
360 s
216 s.
The correct Answer is:D
(d) : According to Newton.s law of cooling
θ1−θ2t=K[θ1+θ22−θ0]
where θ0 is temperature of currounding.
∴80−702=K[80+702−30] . . . (i)
Also 60−50t=K[60+502−30] . . . (ii)
Dividing (i) by (ii) we have :
t2=75−3055−30⇒t2=4525
⇒t=4525×2=9025=185=3⋅6 minutes.
=216 seconds
correct choice is (d).
Topper's Solved these Questions
- TRANSFERENCE OF HEAT BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Multiple Choice Questions (Level - III) Questions From AIEEE/JEE Examination)
5 Videos
- TRANSFERENCE OF HEAT BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Recent Competitive Questions
4 Videos
- TRANSFERENCE OF HEAT BOOK - MODERN PUBLICATIONCHAPTER - TRANSFERENCE OF HEAT EXERCISE - Recent Competitive Questions
4 Videos
- SOLIDS & SEMICONDUCTOR DEVICESBOOK - MODERN PUBLICATIONCHAPTER - SOLIDS & SEMICONDUCTOR DEVICESEXERCISE - Revision Test
28 Videos
- UNIFORM CIRCULAR MOTIONBOOK - MODERN PUBLICATIONCHAPTER - UNIFORM CIRCULAR MOTIONEXERCISE - Revision Test
57 Videos
Similar Questions
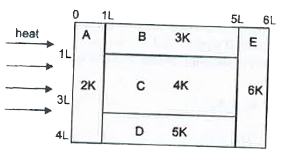
A composite block is made of slabs A,B,C,D and E of different thermal conductivities (given in terms of a constant K) and sizes (given in terms of length, L) as shown in the figure. All slabs are of same width. Heat 'Q' flows only from left to right through the blocks.
Then in steady state
View SolutionTwo slabs are of the thicknesses d1 and d2. Their thermal conductivities are K1 and K2 respectively They are in series. The free ends of the combination of these two slabs are kept at temperatures θ1 and θ2. Assume θ1>θ2. The temperature θ of their common junction is
View SolutionTwo slabs are of the thicknesses d1 and d2. Their thermal conductivities are K1 and K2 respectively They are in series. The free ends of the combination of these two slabs are kept at temperatures θ1 and θ2. Assume θ1>θ2. The temperature θ of their common junction is
View SolutionChoose the forward biased p-n junction :
View SolutionA sphere of mass m is tied to end of a string of length 1 and rotated through the other and along a horizontal circular path with speed . The work done in full horizontal circle is:
View SolutionA liquid cools from 70∘C→60∘C∈5minutes.Calca––tethetimetakenbytheliquc∞lom60^(@)C to 50^(@)C,Ifthetemperatureofthesurround∈gisconstantat30^(@)C .
View SolutionA liquid takes 5 minutes to cool from 80∘C to50∘C . How much time will it take to cool from 60∘C to 30∘C? The temperature of surroundings is 20∘C.
View SolutionA hot liquid kept in a beaker cools from 80∘C to 70∘C in two minutes. If the surrounding temperature is 30∘C, find the time of coolilng of the same liquid from 60∘C to 50∘C.
View SolutionA liquid cools down from 70^(@)C to 60^(@)C in 5 minutes. The time taken to cool it from 60^(@)C to 50^(@)C will be
View SolutionIf a liquid takes 30 seconds in cooling of 80∘C to 70∘C and 70 seconds in cooling 60∘C to 50∘C, then find the room temperature.
View SolutionA hot liquid takes 10 minute to cool from 70^(@)C to 60^(@)C . The time taken by the liquid to cool from 60^(@)C to 50^(@)C is
View SolutionA liquid cools from 70 °C to 60 °C in 5 minutes. If the temperature of the surrounding is constant at 30 °C, then the time taken by the liquid to cool from 60 °C to 50 °C is
View SolutionA hot liquid kept in a breaker cools from 80∘C to 70∘C in two minutes. If the surrounding temperature is 30∘C, then the time of cooling of the same liquid from 60∘C to 50∘C is :
View SolutionA hot liquid in beaker at 80∘C cools down to 70∘C in 2 minutes in an atmosphere of 30∘C temperature, then time taken to cool it down from 60∘C to 50∘C is . . . . . . ..
View SolutionA liquid cools from 70∘C to 60∘C in 5 minutes. Calculate the time taken by the liquid to cool from 60∘C to 50∘C , If the temperature of the surrounding is constant at 30∘C .
View Solution
