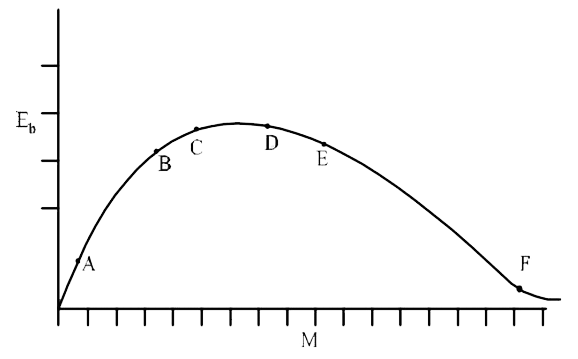
The alongside is a plot of binding energy per nucleon Eb ,against the nuclear mass M,A,B,C,D,E,F correspond to different nuclei. Consider four reactions.
(i) A + B → C +ε (ii) C → A + B + ε
(iii) D + E → F + ε (iv) F → D + E + ε where ε is the energy released. In which reactions , is ε positive?
The alongside is a plot of binding energy per nucleon E(b) ,against the nuclear mass M,A,B,C,D,E,F correspond to different nuclei. Consider four reactions. (i) A + B to C + epsilon (ii) C to A + B + epsilon (iii) D + E to F + epsilon (iv) F to D + E + epsilon where epsilon is the energy released. In which reactions , is epsilon positive?

A

(ii) and (iv)

B

(ii) and (iii)

C

(i) and (iv)

D

(i) and (iii)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Reactions (i) and (iv) are fusion and fission respectively which release energy, so sign of e is positive. The binding energy per nucleon of products is more than that of each reactants.
A+B→C+e fusion reaction.
F→D+E+e fission reaction

Updated on:21/07/2023
|
Class 12PHYSICSNUCLEI

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • ocr_image
    The above is a plot of binding energy per nucleon Eb against the nuclear mass M,A,B,C,D,E,F correspond to different nuclei Consider four reactions:

    AA+B→C+ε
    BC→A+B+ε
    CD+E→F+εand
    DF→D+E+ε,

  • The above is a plot of binding energy per nucleon Eb, against the nuclear mass M, A, B, C, D, E, F correspond to different nuclei. Consider four reactions
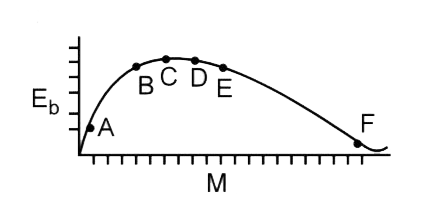
    ocr_image
    (i) A+B→C+ɛ (ii) C→A+B+ɛ
    (iii)D+E→F+ɛ and (iv) F→D+E+ɛ
    Where ɛ is the energy released ? In which reactions is  positive.

    A(i) and (iv)
    B(i) and (iii)
    C(ii) and (iv)
    D(ii) and (iii)

  • The dimensions of 12∈0E2, where ∈0 is permittivity of free space and E is electric field, is :-

    A[M1L1T−2A1]
    B[M1L0T−1]
    C[M1L−1T−2A1]
    D[M1L−1T−2]