An AC source of rms voltage 220 V and variable frequency is connected across a series combination of 5 H inductance 80μF capacitance and 40Ω resistance . Calculate frequency which can drive the circuit to resonance. What will be the impedance of the circuit for this frequency and peak current ?
To solve the problem step by step, we will follow these steps:
Step 1: Identify the given values
- RMS Voltage (V_rms) = 220 V
- Inductance (L) = 5 H
- Capacitance (C) = 80 µF = 80 × 10^(-6) F
- Resistance (R) = 40 Ω
Step 2: Calculate the resonant frequency
At resonance in an RLC circuit, the inductive reactance (X_L) equals the capacitive reactance (X_C). This condition can be expressed mathematically as:
XL=XC
Where:
- XL=ωL
- XC=1ωC
Setting these equal gives:
ωL=1ωC
Multiplying both sides by ω:
ω2=1LC
Thus, the resonant frequency f can be calculated as:
f=12π√1LC
Substituting the values:
f=12π√15×80×10−6
Calculating LC:
LC=5×80×10−6=400×10−6=4×10−4
Now substituting back:
f=12π√14×10−4=12π√2500=12π×50
f≈506.2832≈7.96Hz
Step 3: Calculate the impedance at resonance
At resonance, the impedance Z of the circuit is simply equal to the resistance R:
Z=R=40Ω
Step 4: Calculate the peak current
The RMS current Irms can be calculated using Ohm's law:
Irms=VrmsZ
Substituting the known values:
Irms=22040=5.5A
To find the peak current I0:
I0=√2×Irms
I0=√2×5.5≈1.414×5.5≈7.78A
Summary of Results
- Resonant Frequency f≈7.96Hz
- Impedance at resonance Z=40Ω
- Peak Current I0≈7.78A
- RMS Voltage (V_rms) = 220 V
- Inductance (L) = 5 H
- Capacitance (C) = 80 µF = 80 × 10^(-6) F
- Resistance (R) = 40 Ω
At resonance in an RLC circuit, the inductive reactance (X_L) equals the capacitive reactance (X_C). This condition can be expressed mathematically as:
XL=XC
Where:
- XL=ωL
- XC=1ωC
ωL=1ωC
ω2=1LC
f=12π√1LC
f=12π√15×80×10−6
LC=5×80×10−6=400×10−6=4×10−4
f=12π√14×10−4=12π√2500=12π×50
f≈506.2832≈7.96Hz
At resonance, the impedance Z of the circuit is simply equal to the resistance R:
Z=R=40Ω
The RMS current Irms can be calculated using Ohm's law:
Irms=VrmsZ
Substituting the known values:
Irms=22040=5.5A
I0=√2×Irms
I0=√2×5.5≈1.414×5.5≈7.78A
- Resonant Frequency f≈7.96Hz
- Impedance at resonance Z=40Ω
- Peak Current I0≈7.78A
Topper's Solved these Questions
- ALTERNATING CURRENT Book:MODERN PUBLICATIONChapter:ALTERNATING CURRENT Exercise:PRACTICE PROBLEMS
Explore 67 Videos
- ALTERNATING CURRENT Book:MODERN PUBLICATIONChapter:ALTERNATING CURRENT Exercise:CONCEPTUAL QUESTIONS
Explore 25 Videos
- ATOMS Book:MODERN PUBLICATIONChapter:ATOMS Exercise:Chapter Practice Test
Explore 16 Videos
Similar Questions
A variable frequency 230 V alternating voltage source is connected across a series combination of L=5.0H,C=80μF and R=40Ω
(i) Calculate the angular frequency fo the source which drives the circuit in resonance.
(ii) Obtain r.m.s. the impedance of the circuit and amplitude of current at resonance frequency
(iii) Obtain r.m.s. potential drop across the three elements of the circuit at resonating frequency.
(iv) How do you explain the observation that the algerbraic sum of voltages across the three elements obtained is greater than the supplied voltage.
View SolutionFind the resonant frequency of a series circuit consist of an inductance 200 μH, a capacitance of 0.0005μF and a resistance of 10 Ω.
View Solution
Knowledge Check
A series combination of a 0.2kω resistor and 1.5μF capacitor is given. If, this circuit is connected across a 220 V, 50 Hz AC source what will be the impedance of the circuit
A250ωB268ωC29.15ωD291.5ωIf resistance R =10Ω, inductance L = 2 mH and capacitance C=5μF are connected in series to an AC source of frequency 50 Hz, then at resonance the impedance of circuit is
AZeroB10ΩC1000ΩD10KΩAn alternating current by frequency v is flowing in a circuit containing a resistance R and inductance L in series. The impedance of this circuit is
AR = 2πvLB√R2+4π2v2L2C√R2+L2D√R2+2πvL
A 200 V varialbe frequency a.c. source is connected to a series combination the angular C=80μF and R=40Ω. Calculate the angular frequency of the soucre to get maximum current in the cicuit, the current amplitude at resonance and power dissipated in the circuit.
View Solution(a) An AC source of voltage V=V0sinωt is connected across a series combination of an inductor a capacitor and a resistor . Use the phasor diagram to obtain the expression for the impedance of the circuit and phase angle between the voltage and the current.
(b) A capacitor of unknown capacitance a resistor of 100 Ω and an inductor of self - inductance L =(4/π2) henry are in series connected to an AC source of 200 V and 50 Hz . Calculate the value of the capacitance and the current that flows in the circuit when the current is in phase with the voltage .
View SolutionFigure here, shows a series L-C-R circuit connected to a variable frequency 230 V source. L = 5.0H, C = 80μF and r =40Ω
(a) Determine the source frequency which drives the circuit in resonance.
(b) Obtain the impedance of the circuit and the amplitude of current at the resonating frequency.
(c) Determine the rms potential drops across the three elements of the circuit. show that the potential drop across the L-C combination is zero at the resonating frequency.
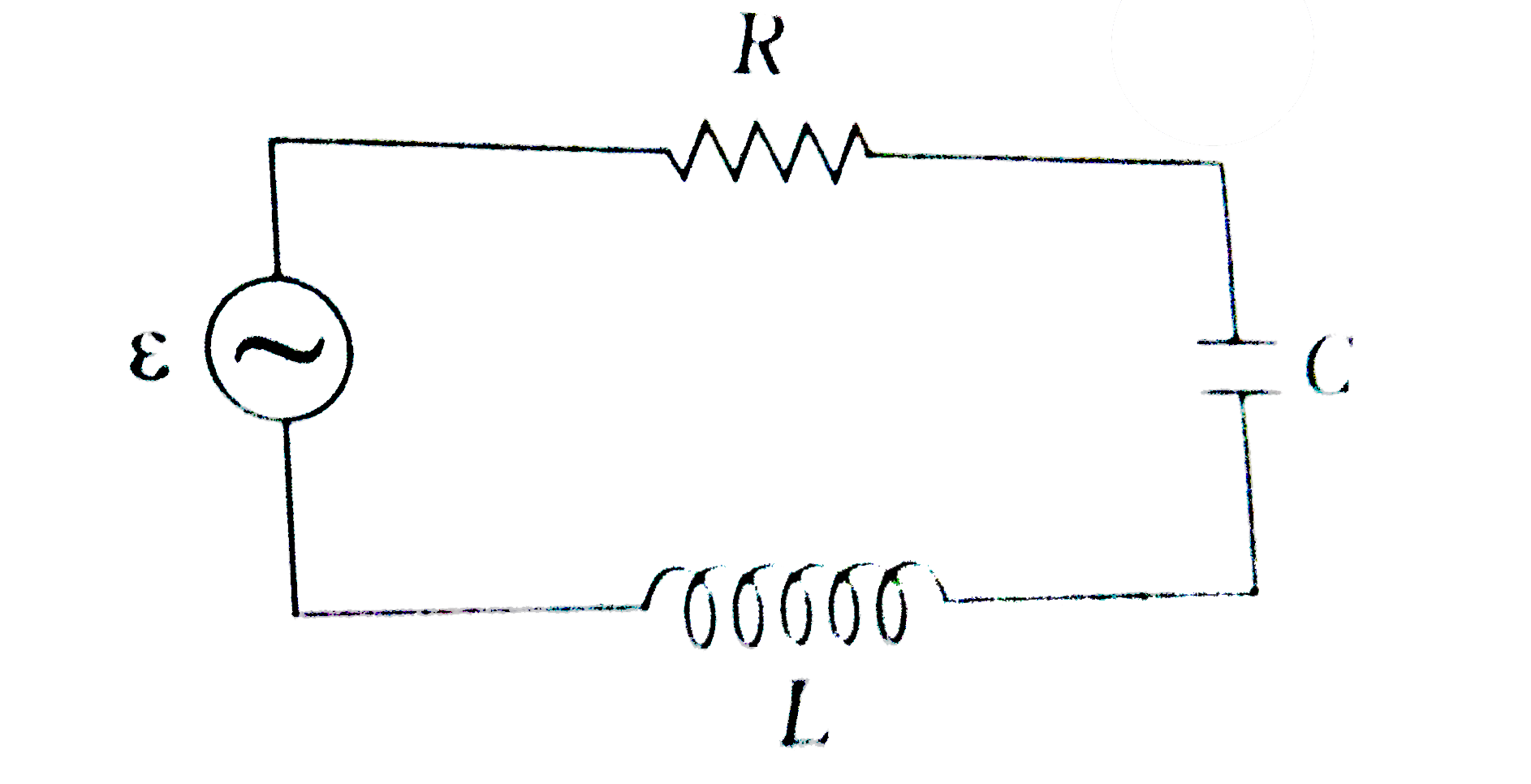
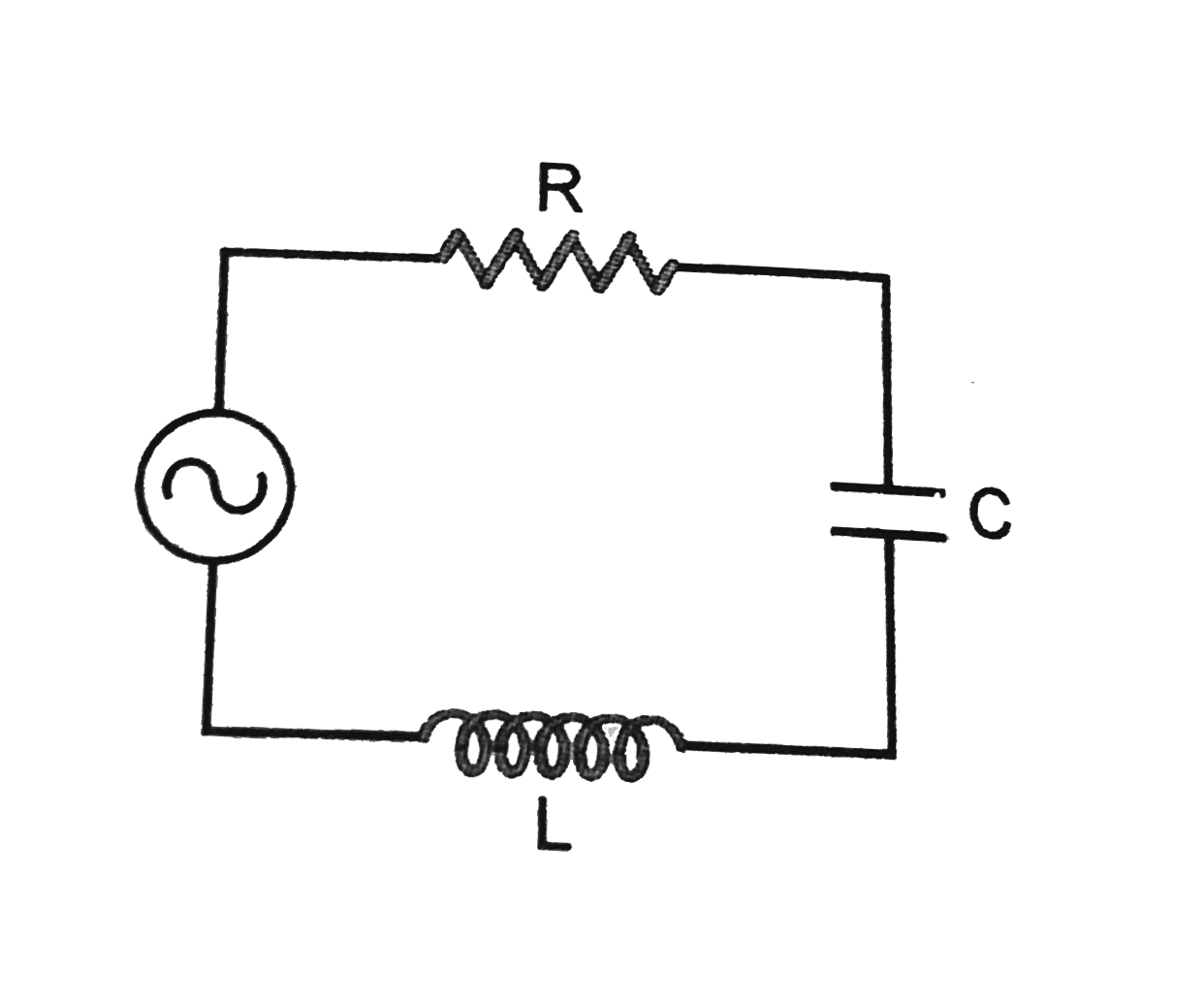
View SolutionA series LCR circuit connected to a variable frequency 230 V source has L = 5.0 H, C=80μF, R=40Ω, Fig.
(a) Determine the source frequency which drives the circuit in resonance.
(b) Obtain the impedance of the circuit and amplitude of current at at resonating frequency.
(c ) Determine the r.m.s. potential drops acorss the three elements the circuits. Show that the potential drop acorss the LC combination is zero at the resonating frequecny.
View SolutionAn inductor of resistance 80 Ω is connected to an AC source of frequency 50 Hz . Calculate the inductance if the impedance of circuit is 150 Ω .
View Solution