Two uniform sheets of charge with surface charge densities +σ and −σ are intersecting at right angles to each other. Electric field intensity due to this system is
σ2ε0
σ2√2ε0
σε0√2
None
The correct Answer is:C
To find the electric field intensity due to two uniform sheets of charge with surface charge densities +σ and −σ that intersect at right angles, we can follow these steps:
Step 1: Understand the Electric Field due to a Single Sheet
The electric field E due to an infinite sheet of charge with surface charge density σ is given by the formula:
E=σ2ϵ0
where ϵ0 is the permittivity of free space.
Step 2: Determine the Direction of Electric Fields
- For the positively charged sheet with surface charge density +σ, the electric field E1 points away from the sheet.
- For the negatively charged sheet with surface charge density −σ, the electric field E2 points towards the sheet.
Step 3: Analyze the Configuration
Since the two sheets intersect at right angles, we can consider the electric fields E1 and E2 to be perpendicular to each other.
Step 4: Calculate the Magnitudes of the Electric Fields
From Step 1, we know:
E1=σ2ϵ0(from the +\sigma sheet)
E2=σ2ϵ0(from the -\sigma sheet)
Step 5: Use the Pythagorean Theorem to Find the Resultant Electric Field
Since E1 and E2 are perpendicular, the net electric field Enet can be calculated using the Pythagorean theorem:
Enet=√E21+E22
Step 6: Substitute the Values
Substituting the values of E1 and E2:
Enet=√(σ2ϵ0)2+(σ2ϵ0)2
Enet=√2(σ2ϵ0)2
Enet=σ2ϵ0√2
Step 7: Final Result
Thus, the electric field intensity due to the system is:
Enet=σ√22ϵ0
The electric field E due to an infinite sheet of charge with surface charge density σ is given by the formula:
E=σ2ϵ0
where ϵ0 is the permittivity of free space.
- For the positively charged sheet with surface charge density +σ, the electric field E1 points away from the sheet.
- For the negatively charged sheet with surface charge density −σ, the electric field E2 points towards the sheet.
Since the two sheets intersect at right angles, we can consider the electric fields E1 and E2 to be perpendicular to each other.
From Step 1, we know:
E1=σ2ϵ0(from the +\sigma sheet)
E2=σ2ϵ0(from the -\sigma sheet)
Since E1 and E2 are perpendicular, the net electric field Enet can be calculated using the Pythagorean theorem:
Enet=√E21+E22
Substituting the values of E1 and E2:
Enet=√(σ2ϵ0)2+(σ2ϵ0)2
Enet=√2(σ2ϵ0)2
Enet=σ2ϵ0√2
Thus, the electric field intensity due to the system is:
Enet=σ√22ϵ0
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS Book:MODERN PUBLICATIONChapter:ELECTRIC CHARGES AND FIELDS Exercise:COMPETITION FILE ( Objective Question (BA.MCQ) )
Explore 26 Videos
- ELECTRIC CHARGES AND FIELDS Book:MODERN PUBLICATIONChapter:ELECTRIC CHARGES AND FIELDS Exercise:COMPETITION FILE ( Objective Question (BA.MCQ))
Explore 3 Videos
- ELECTRIC CHARGES AND FIELDS Book:MODERN PUBLICATIONChapter:ELECTRIC CHARGES AND FIELDS Exercise:Revision Exercises (Numerical Problems )
Explore 7 Videos
- DUAL NATURE OF RADIATION AND MATTER Book:MODERN PUBLICATIONChapter:DUAL NATURE OF RADIATION AND MATTER Exercise:CHAPTER PRACTICE TEST
Explore 15 Videos
- ELECTROMAGNETIC INDUCTIONBook:MODERN PUBLICATIONChapter:ELECTROMAGNETIC INDUCTIONExercise:CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
Explore 14 Videos
Similar Questions
If three infinite charged sheets of uniform surface charge densities σ,2σ and −4σ are placed as shown in figure, then find out electric field intensities at points A, B, C and D.
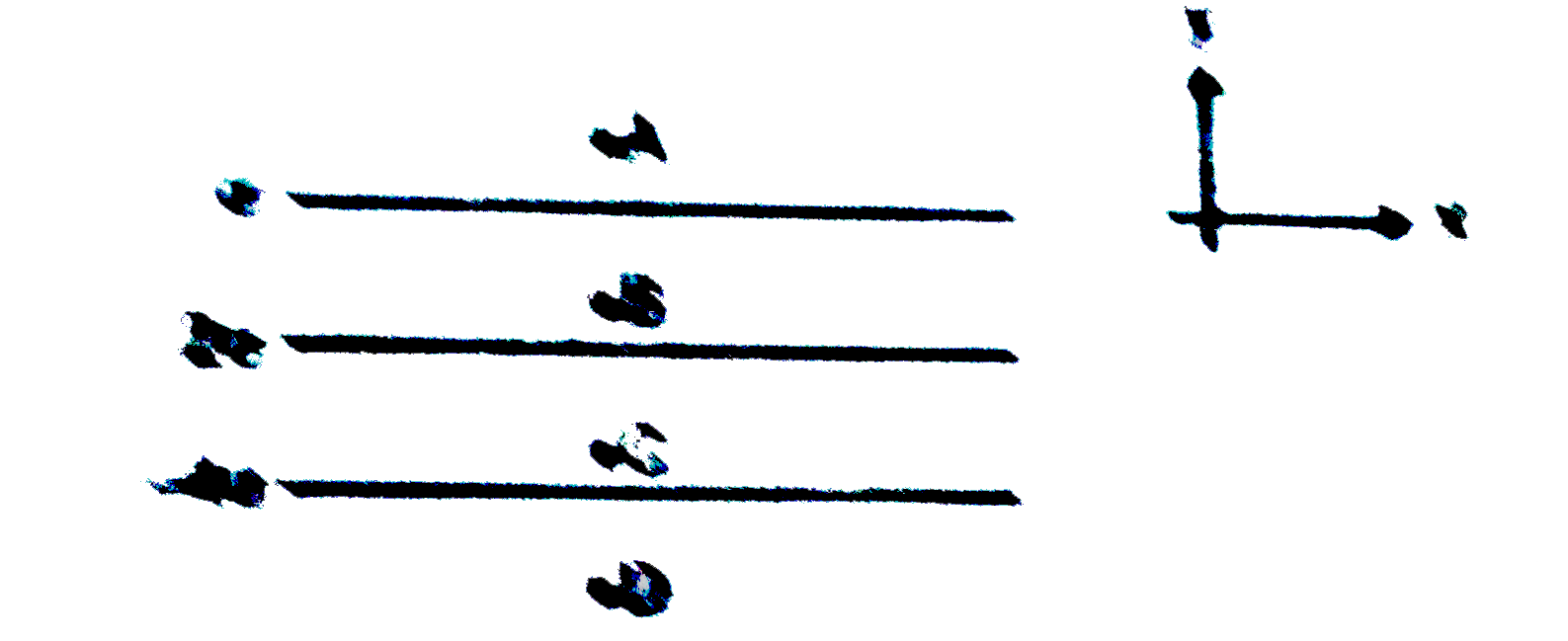
View SolutionA uniformly charged disc of radius R having surface charge density σ is placed in the xy plane with its center at the origin. Find the electric field intensity along the z-axis at a distance Z from origin :
View Solution
Knowledge Check
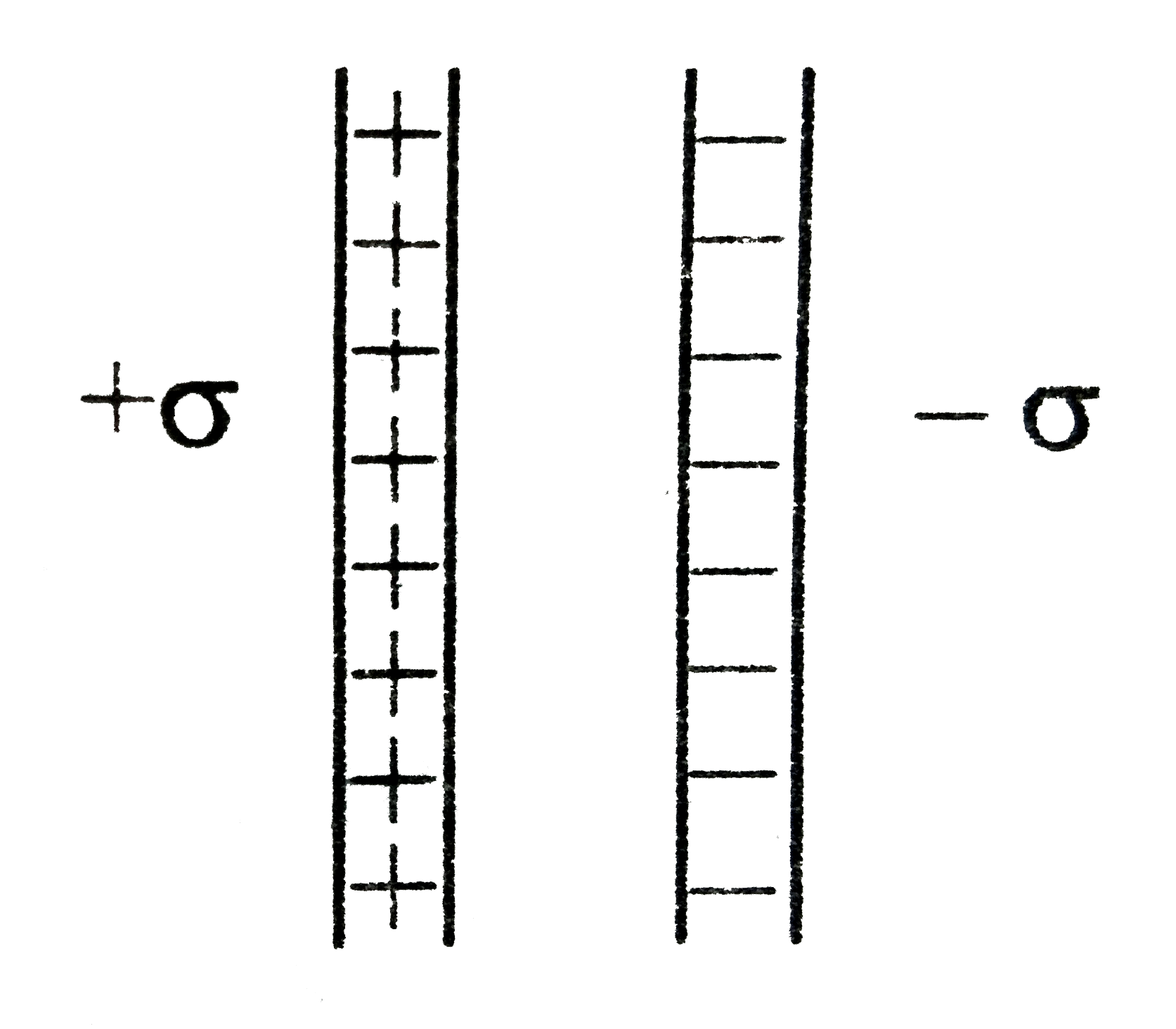
Two plane sheets of charge densities +σand−σ are kept in air as shown in Fig. What are electric field intensities at points A and B ?
 Aσε0,0B0,0Cσε0,σε0D0,σε0
Aσε0,0B0,0Cσε0,σε0D0,σε0Two infinitely long parallel sheets having surface charge densities +σand−σ respectively are separated by a small distance. The electric field in the region between the plates is
A0Bσ2∈0Cσ∈0D2σ∈0Two infinite sheets of uniform charge debsity +σ and −σ are parallel to each other as shown in the gugrue . Elctric field at the
 .Apoints to the left or to the right of the sheets is zero .Bmidpoint between the sheets is zero.Cmidpoint of the sheets is σ/ɛ0 and is directed towards right .Dmidpoint of the sheet is 2σ/ɛ0 and is directed towards right .
.Apoints to the left or to the right of the sheets is zero .Bmidpoint between the sheets is zero.Cmidpoint of the sheets is σ/ɛ0 and is directed towards right .Dmidpoint of the sheet is 2σ/ɛ0 and is directed towards right .
Two thin infinite sheets have uniform surface densities of charge +σ and −σ . Electric field in the space between the two sheets is
View SolutionTwo infinite non-conducting sheets each with uniform surface charge density sigma are kept in such a way that the angle between them is 30^(@). The electric field in the region I shown between them is
View SolutionTwo large parallel conducting plates are placed close to each other ,the inner surface of the two plates have surface charge densities +σ and −σ .The outer surfaces are without charge.The electric field has a magnitude of
View SolutionTwo large parallel sheets charged uniformly with surfasce charge density σ and −σ are located as shown in the figure. Which one of the following graphs shows the variation of electric field along a line perpendicular to the sheets as one moves from A to B ?

View SolutionTwo charged thin infinite plane sheets of uniform surface charge density σ+andσ− where |σ+|>|σ−| , intersect right angle Which of the following best represents the electric field lines for this system:
View Solution