In hydrogen atom , electron excites from ground state of higher energy state and its orbital velocity is reduced to (13)rd of its initial value . The radius of the orbit in the ground state is R . The radius of the orbit in that higher energy state is ... Solution in Kannada
2 R
3 R
27 R
9 R
The correct Answer is:D
As per question ,
vhvg=13 …(i)
where subscripts h and q denotes higher energy state and ground state .
Orbital velocity of electron in the nth orbit is vn=e22ε0nhorvn∝1n
For ground state , n = 1
∴vhvg=1n ...(ii)
Equating (i) and (ii) , we get n = 3
Radius of nth orbit is
rn=n2h2ε0πe2m
or rn∝n2
∴r3r1=(3)2(1)2=9
r3=9r1=9R (∵r1=R (Given))
Topper's Solved these Questions
Similar Questions
All capacitors used in the diagram are identical and each is capacitance C .Then the effective capacitance between the points A and B is
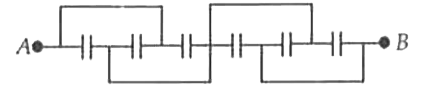
View SolutionWhen an additional charge 2C is given to a capacitor, energy stored in it is increased by 21%. The original charge of the capacitor is
View SolutionThe electron in a hydrogen atom jumps from ground state to the higher energy state where its velcoity is reduced to one-third its initial value. If the radius of the orbit in the ground state is r the radius of new orbit will be
View SolutionThe radius of the Bohr orbit in the ground state of hydrogen atom is 0.5 Å . The radius o fthe orbit of the electron in the third excited state of He^(+) will be
View SolutionIf an orbital electron of the hydrogen atom jumps from the groud state to a higher energy state, its orbital value. If the radius of the electron orbit in the ground state is r , then the radius of the new orbit would be:
View SolutionThe orbital electron of the hydrogen atom jumps from the ground state to a higher energy state and its orbital velocity is reduced to one third of its initial value. If the radius of the orbit in the ground state is r, then what is the radius of the new orbit ?
View SolutionThe orbital velocity of the electron in the ground state of hydrogen atom is v. What will be its orbital velocity when excited to the energy state= −0.544eV?
View SolutionAn orbital electron in the ground state of hydrogen has magnetic moment μ1 .This orbital electron is excited to 3rd excited state by some energy transfer to the hydrogen atom. The new magnetic moment of the electron is μ2
View SolutionIf the radius of electron orbit in the excited state of hydrogen atom is 476.1 pm, the energy of electron in that excited state in J is Radius and energy of electron in the first orbit of hydrogen atom are 52.9 pm and −218×10−18J respectively)
View SolutionCalculate the energy required to excite an electron from ground state to 3rd excited state if the energy of electron in nth orbit is −13.6/n2
View SolutionIn hydrogen atom , electron excites from ground state of higher energy state and its orbital velocity is reduced to (13)rd of its initial value . The radius of the orbit in the ground state is R . The radius of the orbit in that higher energy state is ...
View SolutionThe radius of the Bohr orbit in the ground state of hydrogen atom is 0.5 Å. The radius of the orbit of the electron in the third excited state of He will be
View SolutionThe value of ground state energy of hydrogen atom is - 13.6 eV.
(a) Find the energy required to move an electron from the ground state to the first excited state of the atom.
(b) Determine (i) the kinetic energy, and (ii) the orbital radius in the first excited state of the atom.
(Given the value of Bohr radius =0.53Å)
View SolutionThe radius of the Bohr orbit in the ground state of hydrogen atom is 0.5 the radius of the orbit of the electron in the third excited state of He^(+) will be
View SolutionThe ionisation energy of hydrogen atom (in the ground state) is x kJ. The energy required for an electron to jump from 2^(nd) orbit to the 3^(rd) orbit will be
View Solution