Spheres of iron and lead having same mass are completely immersed in water . Density of lead is more than that of iron . Apparent loss of weight is W1 for iron sphere and W2 for lead sphere . Then W1W2 is Solution in Kannada
=1
between 0 and 1
=0
>1
The correct Answer is:D
Density (ρ)=Mass (M)Volume (V)
p∝1V For the same mass .
∴ρleadρiron=VironVlead
As ρlead>ρiron (Given)
∴VironVlead>1 ...(1)
Using law of floatation ,
For iron sphere
W1=Vironρwaterg ....(ii)
For lead sphere
W2=Vleadρwaterg ....(iii)
Divide (ii) by (iii) , we get
W1W2=VironVlead>1 (Using (i))
Topper's Solved these Questions
Similar Questions
All capacitors used in the diagram are identical and each is capacitance C .Then the effective capacitance between the points A and B is
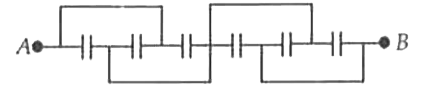
View SolutionWhen an additional charge 2C is given to a capacitor, energy stored in it is increased by 21%. The original charge of the capacitor is
View SolutionA rod AB of weight w_(1) is placed over a sphere of weight w_(2) shown in figure.Ground is rought and there is no friction between rod and sphere and sphere and wall. Draw free body diagrame of sphere and rod separalety.
View SolutionThe relative density of a material of a body is found by weighing it first in air and then in water. If the weight of he body in air is W_(1) = 8.00 +- N and the weight in water is W_(2) = 6.00 +- 0.05 N , then the relative density rho_(r ) = W_(1)//( W_(1) - W_(2)) with the maximum permissible eror is
View SolutionTwo weights W_(1) and W_(2) in equilibrium and at rest, are suspended as shown in figure. Then the ratio (W_(1))/(W_(2)) is :
View SolutionIf the weight of a body in vacuum is w and w1 and w2 are weights when it is immersed in a liquid of specific gravity ρ1 and ρ2 respectively, then find the relation among w,w1andw2
View SolutionA density bottle weighs 120 g and 100 g when filled completely with oil ad water, respectively. If the weight of a empty density bottle is 40g, then arrange the following steps in sequence meant to solve the problem to get the density of oil
(A). The density of the oil
D=Mass of the oilWeight of the water=Mass of the oilMass of the water
=Weighht of oilWeight of the water=W3−W1W2−W1
(B). Let the weight of the bottle +oil=W3=120g
and the weight of the bottle+water =W2
100g, where bottle is completely filled with liquid, i.e., oil or water.
(C). Let the weight of the empty bottle=W1=40g
(D). The the weight of the oil ad water would be equal to (W3−W1)and(W2−W1), respectively.
View Solution[W_(1),W_(2),W_(3)" are apparent "],[" weights of a solid in water at "],[0^(0)C,4^(0)c" and "50^(0)c],[" respectively.If expansion of "],[" solid is negligible,then "],[" A) "W_(1)=W_(2)=W_(3)],[" B) "W_(1)>W_(2)>W_(3)],[" C) "W_(3)>W_(1)>W_(2)],[" D) "W_(3)>W_(2)>W_(1)]
View SolutionChoose the correct answer Two spheres of iron and lead with the same mass were completely submerged in water. The density of lead is higher than that of iron Loss of weight of iron and lead spheres respectively w_1 If he is w_2 w_1//w_2 এর মান
View SolutionA metal sphere immersed in water weighs w1 at 0∘C and w2 at 50∘C . The coefficient of cubical expansion of the metal is less than that of water . Then
View SolutionSphere of iron and lead having same mass are completely immersed in water. Density of lead is more than that of iron. Apparent loss of weight is w_(1) for iron sphere and w_(2) for lead sphere. Then w_(1)//w_(2) is
View SolutionApparent weight of a body immersed in water at 20∘C is W1. When temperature is increased to 40∘C, the apparent weight becomes W2. In this case
View SolutionSpheres of iron and lead having same mass are completely immersed in water. Density of lead is more than that of iron. Apparent loss of weight is W_(1) for iron sphere and W_(2) for lead sphere. Then (W_(1))/(W_(2)) is :
View SolutionIf W1:W2=2:3 and W1:W3=1:2 then W2:W3 is
View SolutionIron and lead shells of the same mass are completely immersed in water. The density of lead is higher than iron. Lack of load for iron shells W_(1) And for lead shells W_(2) Be then (W_(1))/(W_(2)) Will happen.
View Solution
