If 34=k12, then k is ______
3
4
6
9
The correct Answer is:D
To solve the equation 34=k12 for k, we can follow these steps:
Step 1: Set up the equation
We start with the equation:
34=k12
Step 2: Cross-multiply
To eliminate the fractions, we can use cross-multiplication. This means we multiply the numerator of one fraction by the denominator of the other fraction:
3×12=k×4
Step 3: Calculate the left side
Now, calculate 3×12:
3×12=36
So, we have:
36=k×4
Step 4: Solve for k
Next, we need to isolate k. To do this, divide both sides of the equation by 4:
k=364
Step 5: Calculate k
Now, calculate 364:
364=9
Thus, the value of k is:
k=9
Final Answer
The required value of k is 9.
---
We start with the equation:
34=k12
To eliminate the fractions, we can use cross-multiplication. This means we multiply the numerator of one fraction by the denominator of the other fraction:
3×12=k×4
Now, calculate 3×12:
3×12=36
So, we have:
36=k×4
Next, we need to isolate k. To do this, divide both sides of the equation by 4:
k=364
Now, calculate 364:
364=9
k=9
The required value of k is 9.
Topper's Solved these Questions
- FRACTIONS AND DECIMALS Book:PEARSON IIT JEE FOUNDATIONChapter:FRACTIONS AND DECIMALS Exercise:Short Answer type Qns
Explore 26 Videos
- FRACTIONS AND DECIMALS Book:PEARSON IIT JEE FOUNDATIONChapter:FRACTIONS AND DECIMALS Exercise:Concept Application
Explore 5 Videos
- FRACTIONS AND DECIMALS Book:PEARSON IIT JEE FOUNDATIONChapter:FRACTIONS AND DECIMALS Exercise:Exercise very Short Ans Type
Explore 2 Videos
- FACTORS AND MULTIPLES Book:PEARSON IIT JEE FOUNDATIONChapter:FACTORS AND MULTIPLES Exercise:Crossword
Explore 1 Video
- GEOMETRYBook:PEARSON IIT JEE FOUNDATIONChapter:GEOMETRYExercise:CROSSWORD
Explore 1 Video
Similar Questions
If (2,k,−1) is the centroid of the triangle with vertices (2,−1,2),(1,−3,4) and (3,k,−1), then k=_____.
View SolutionIf 3644 k is the term, independent of x in the binomial expansion of (x4−12x2)12 then k is equal to ____________
View SolutionIf four points with position vectors A(−i+2j+3k),B(−i−12j−3k),C(2i−j−4k)andD(2i+λj−k) are coplanar, then λ = _________
View SolutionIf3√a6×b3×c21c9×a12=bckak/2'then k=______ .
View SolutionIf 3k−7 is the arithmetic mean of 3k+4 and k+12 then the value of 2k+5
View SolutionIf A=[1242], then the value of k=_________if |2A|=k|A|.
View SolutionIf A=[1234], then kA (where 'k' is a scalar) = ________.
View SolutionLet (nk) denote nCk and
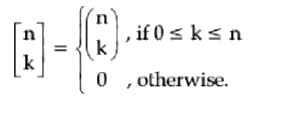
If Ak=9∑i=0(9i)[ 1212−k+i]+8∑i=0(8i)[ 1313−k+i]and A4−A3=190p ,then p is equal to
View SolutionIf x+y=k is normal to y2=12x, then k is 3 (b) 9 (c) −9 (d) −3
View Solutionkx+3y=k−3,12x+ky=k
View Solution
