If $a, b, c$ are in $H . P$ , $b, c, d$ are in $G . P$ and $c, d, e$ are in $A . P .$ , then the value of $e$ is

A

$\frac{a b^{2}}{{(2 a - b)}^{2}}$

B

$\frac{a^{2} b}{{(2 a - b)}^{2}}$

C

$\frac{a^{2} b^{2}}{{(2 a - b)}^{2}}$

D

None of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

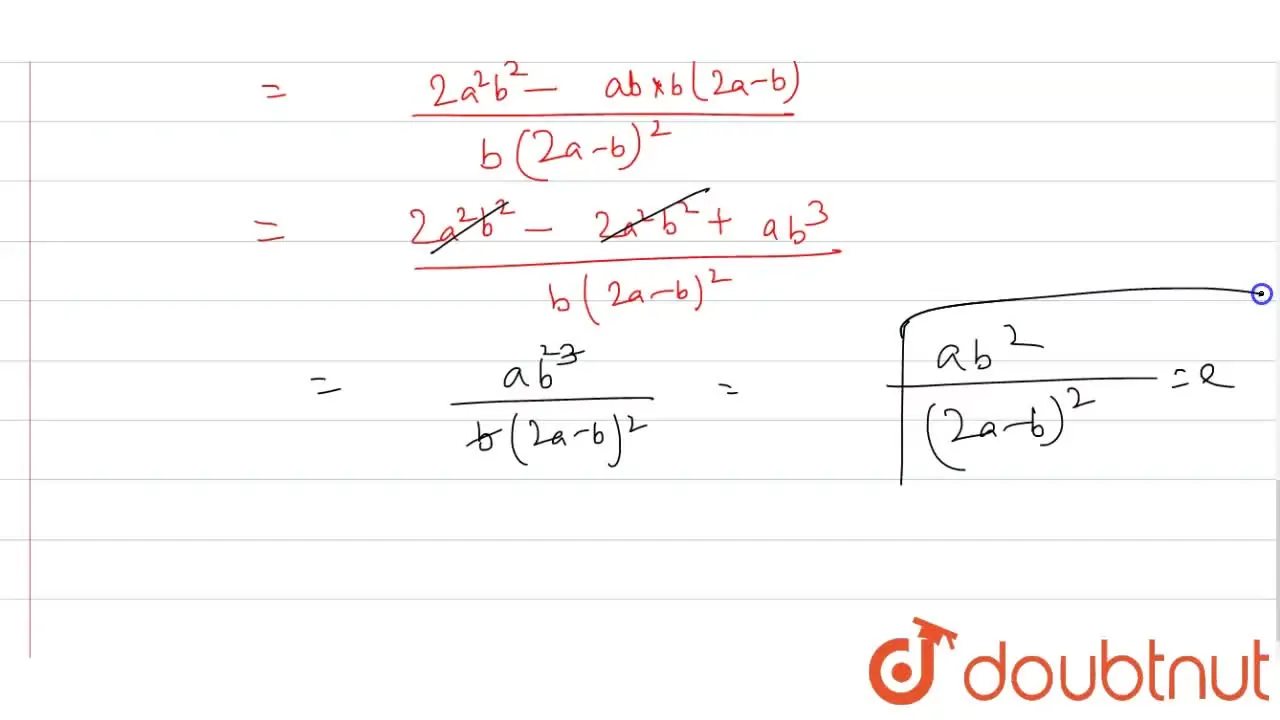
$(a)$ $a, b, c$ are in $H . P . \Rightarrow b = \frac{2 a c}{a + c}$ …. $(i)$
$b, c, d$ are in $G . P . \Rightarrow c^{2} = b d$ .. $(i i)$
$c, d, e$ are in $A . P . \Rightarrow d = \frac{c + e}{2}$ ….. $(i i i)$
From $(i)$ , $a b + b c = 2 a c$
$\Rightarrow c = \frac{a b}{2 a - b}$ …. $(i v)$
From $(i i i)$ and $(i v)$
$d = \frac{1}{2} [\frac{a b}{2 a - b} + e]$ ...... $(v)$
From $(i i)$ , $(i v)$ and $(v)$
$\frac{a^{2} b^{2}}{{(2 a - b)}^{2}} = \frac{b}{2} [\frac{a b}{2 a - b} + e]$
$\Rightarrow e = \frac{a b^{2}}{{(2 a - b)}^{2}}$

|

Updated on:21/07/2023

Class 12 MATHS PROGRESSION AND SERIES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If a,b,c, are in A.P., b,c,d are in G.P. and c,d,e, are in H.P., then a,c,e are in
AA.P.
BG.P.
CH.P.
Dnone of these
Question 2 - Select One
if a,b,c are in A.P. b,c,d are in G.P. and c,d,e are in H.P then a,c,e are in
AA.P
BG.P
CH.P
DNone of these
Question 3 - Select One
If a,b,c, are in A.P., b,c,d are in G.P. and c,d,e, are in H.P., then a,c,e are in
AA.P.
BG.P.
CH.P.
Dnone of these

CENGAGE-PROGRESSION AND SERIES-Single correct Answer

Home
Ask Doubt
Profile