If ∣∣x2−2x−8∣∣+∣∣x2+x−2∣∣=3|x+2|, then the set of all real values of x is
A
[1,4]∪{−2}
B
[1,4]
C
[−2,1]∪[4,∞)
D
(−∞,−2]∪[1,4]
Video Solution
Text Solution
The correct Answer is:A
(a) ∣∣x2−2x−8∣∣+∣∣x2+x−2∣∣=3|x+2|
Now (x2+x−2)−(x2−2x−8)=3x+6=3(x+2)
∴(x2−2x−8)(x2+x−2)≤0
(x−4)(x+2)2(x−1)≤0
The sign scheme is shown below.
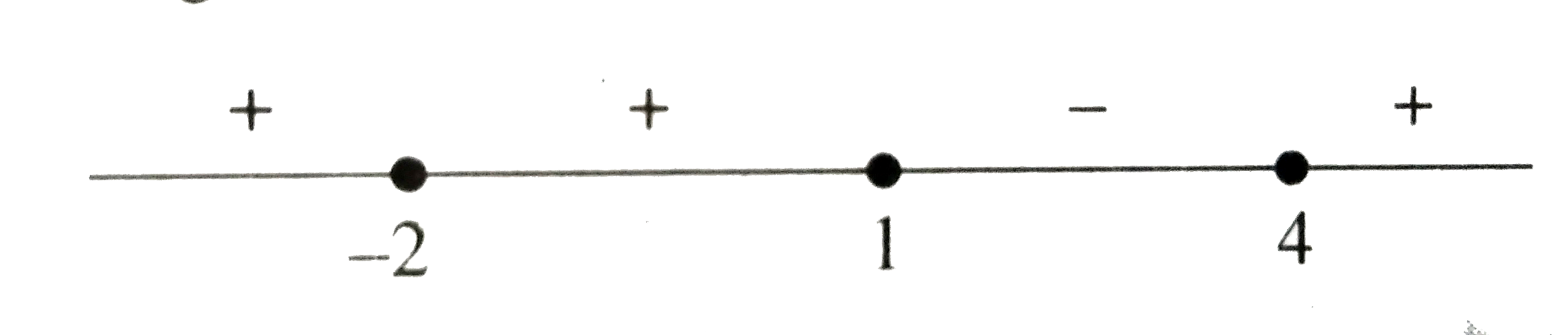
From the sign scheme, x∈[1,4]∪{−2}
|
Topper's Solved these Questions
- INEQUALITIES AND MODULUSBook:CENGAGEChapter:INEQUALITIES AND MODULUSExercise:Single correct Answer
Explore 21 Videos
- INDEFINITE INTEGRATIONBook:CENGAGEChapter:INDEFINITE INTEGRATIONExercise:Question Bank
Explore 25 Videos
- INEQUALITIES INVOLVING MEANS Book:CENGAGEChapter:INEQUALITIES INVOLVING MEANS Exercise:Exercise (Numerical) & JEE Previous Year
Explore 11 Videos
Similar Questions
Knowledge Check
If ∣∣x2−2x+2∣∣−∣∣2x2−5x+2∣∣=∣∣x2−3x∣∣ then the set of values of x is
A(−∞,0]∪[3,00)B[0,12]∪[2,3]C(−∞,0]∪[12,2]∪[3,∞)D[0,2]∪[3,∞)If range of f(x)=x2−3x+2x2−ax+4 is R- (1) then sum of all possible real value(s) of 'a' is
A4B3C5DNone of these
If x∈R such that x2+9x2(x+3)2=27 then sum of squares of all real values of x satisfying given equation is
View SolutionIf x is real, then the maximum value of 3−6x−8x2 is
View SolutionIf ax2−2x+1>0 for atleast one positive real x ,then set of all values of a is
View SolutionIf ax2−2x+1>0 for atleast one positive real x, then set of all values of a is
View SolutionLet f(x)=2x2x2+5x+2andg(x)=1x+1. Find the set of real values of x for which f(x)>g(x).
View SolutionIf (x−2)2(3−x)3(5−x)4(4−x)3<0, then the exhaustive set of real values of x ,is :
View Solution
