The complete set of values of x for which
x3(x−1)2(x+4)(x+1)(x−3)≥0 is
A
(−∞,−4]∪(−1,0]∪(3,∞)∪{1}
B
(−∞,−4]∪(−1,0]∪(3,∞)∪{−1}
C
[−4,−1)∪[0,1)∪(3,∞)
D
[−4,−1)∪[0,1]∪(3,∞)
Video Solution
Text Solution
The correct Answer is:A
(a) We have E=x3(x−1)2(x+4)(x+1)(x−3)
Sign scheme of E is as shown below.
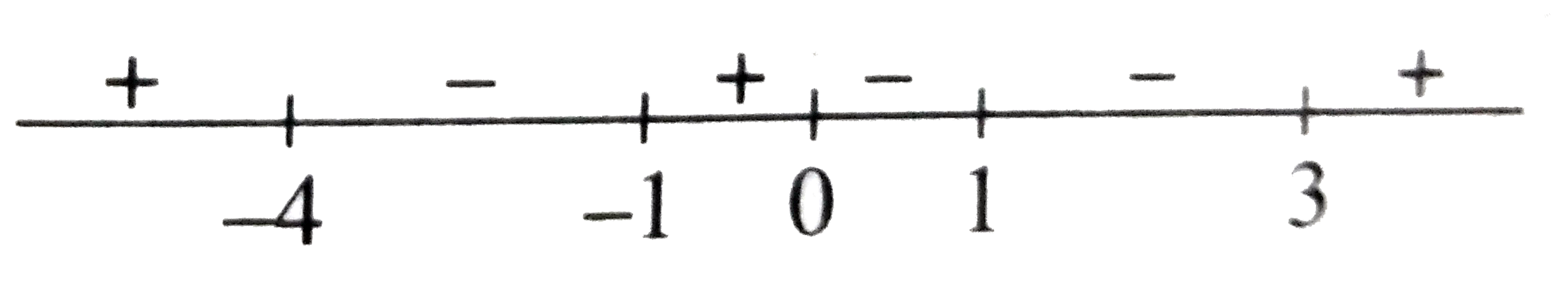
At x=1, sign of the expression does not change as (x−1) has power '2'.
From the sign scheme.
x∈(−∞,−4]∪(−1,0]∪(3,∞)∪{1}
|
Topper's Solved these Questions
- INEQUALITIES AND MODULUSBOOK - CENGAGECHAPTER - INEQUALITIES AND MODULUSEXERCISE - Single correct Answer
21 Videos
- INDEFINITE INTEGRATIONBOOK - CENGAGECHAPTER - INDEFINITE INTEGRATIONEXERCISE - Question Bank
25 Videos
- INEQUALITIES INVOLVING MEANS BOOK - CENGAGECHAPTER - INEQUALITIES INVOLVING MEANS EXERCISE - Exercise (Numerical) & JEE Previous Year
11 Videos
Similar Questions
Knowledge Check
The complete set of values of x for which 2tan−1x+cos−1(1−x21+x2) is independent of x is :
A(−∞,0]B[0,∞)C(−∞,−1]D[1,∞)The set of all values of x for which
(x+1)(x−3)2(x−5)(x−4)3(x−2)x<0A(−∞,−1)∪(0,2)∪(4,5)B(−1,0)∪(2,4)∪(5,∞)C(−1,0)∪(2,3)∪(4,5)D(−∞,−1)∪(0,2)∪[3,5)The set of values for which x3+1≥x2+x is
Ax≤0Bx≥0Cx≥−1D−1≤x≤1
Find the values of x for which x2−4x+3x2+x+1<0
View SolutionFind the values of x for which f(x)=(2x−1)(x−1)2(x−2)3(x−4)4>0
View SolutionLet f(x)=ax−xlna, a>1. Then the complete set of real values of x for which f'(x)>0 is
View SolutionComplete set of values of x for which log1/2x>log1/3x are :
View SolutionComplete set of real values of x for which log(2x−3)(x2−5x−6) is defined is :
View Solution
