If the angle (θ) between velocity vector and the acceleration vector of a moving particle is 90∘<θ<180∘, then the path of the particle is
Straight path with retardation
Straight path with acceleration
Curvilinear path with acceleration
Curvilinear path with retardation
The correct Answer is:D
To solve the question, we need to analyze the motion of a particle when the angle θ between the velocity vector and the acceleration vector is between 90∘ and 180∘.
1. Understanding the Vectors:
- The velocity vector →v indicates the direction of motion of the particle.
- The acceleration vector →a indicates how the velocity is changing over time.
2. Angle Between Vectors:
- Given that 90∘<θ<180∘, this means that the acceleration vector is directed opposite to the velocity vector. The particle is slowing down.
3. Velocity Components:
- We can decompose the velocity vector into components. If we assume the angle ϕ=θ−90∘, then:
- The component of velocity along the x-axis is vcos(ϕ).
- The component of velocity along the y-axis is vsin(ϕ).
4. Equations of Motion:
- Assuming the x-axis is along the direction of the velocity, we can write the equations of motion:
- For the x-direction:
x=vcos(ϕ)t+12axt2
- For the y-direction:
y=vsin(ϕ)t+12ayt2
5. Substituting Time:
- From the x-direction equation, we can express time t in terms of x:
t=xvcos(ϕ)
6. Substituting into the y-equation:
- Substitute t into the y-equation:
y=vsin(ϕ)(xvcos(ϕ))+12ay(xvcos(ϕ))2
- Simplifying this gives:
y=xtan(ϕ)−ayx22v2cos2(ϕ)
7. Identifying the Path:
- The equation derived resembles the standard form of a parabola y=ax2+bx+c.
- Since the coefficient of x2 is negative, it indicates that the parabola opens downwards.
8. Conclusion:
- Therefore, when the angle θ between the velocity and acceleration vectors is between 90∘ and 180∘, the path of the particle is a curvilinear path with retardation.
Final Answer:
The path of the particle is a curvilinear path with retardation.
- The velocity vector →v indicates the direction of motion of the particle.
- The acceleration vector →a indicates how the velocity is changing over time.
- Given that 90∘<θ<180∘, this means that the acceleration vector is directed opposite to the velocity vector. The particle is slowing down.
- We can decompose the velocity vector into components. If we assume the angle ϕ=θ−90∘, then:
- The component of velocity along the x-axis is vcos(ϕ).
- The component of velocity along the y-axis is vsin(ϕ).
- Assuming the x-axis is along the direction of the velocity, we can write the equations of motion:
- For the x-direction:
x=vcos(ϕ)t+12axt2
- For the y-direction:
y=vsin(ϕ)t+12ayt2
- From the x-direction equation, we can express time t in terms of x:
t=xvcos(ϕ)
- Substitute t into the y-equation:
y=vsin(ϕ)(xvcos(ϕ))+12ay(xvcos(ϕ))2
- Simplifying this gives:
y=xtan(ϕ)−ayx22v2cos2(ϕ)
- The equation derived resembles the standard form of a parabola y=ax2+bx+c.
- Since the coefficient of x2 is negative, it indicates that the parabola opens downwards.
- Therefore, when the angle θ between the velocity and acceleration vectors is between 90∘ and 180∘, the path of the particle is a curvilinear path with retardation.
The path of the particle is a curvilinear path with retardation.
Similar Questions
Match the following.
At a certain moment in time the angle between the velocity →v of a particle and the acceleration vector a of that particle is 0. Match the type of motion of the particle at this moment for different θ is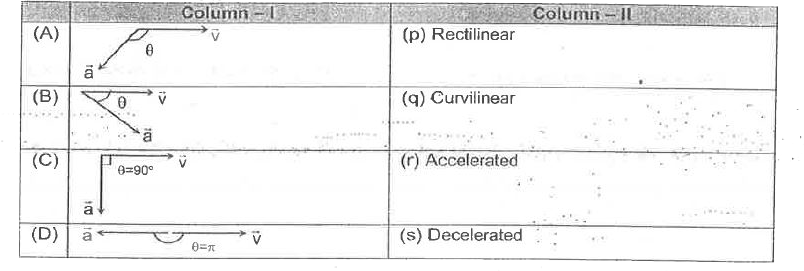
View SolutionAt a certain moment in time the angle between the velocity vector v of a material particle and the acce leration vector w of that particle is θ. What will be the motion of the particle at this moment for different θs: rectilinear or curvilinear, accelerated or uniform or decelerated?
View Solution
Knowledge Check
If 0<θ<90∘, the (sinθ+cosθ) is :
Aless than 1Bequal to 1Cgreater than 1Dnone of the aboveIf 0∘<θ<90∘, then the value of sinθ+cosθ is
Aequal to 1Bgreater than 1Cless than 1Dequal to 2The path of a charged particle in a uniform magnetic field depends on the angle θ between velocity vector and magnetic field, When θis0∘or180∘,Fm=0 hence path of a charged particle will be linear.
When θ=90∘, the magnetic force is perpendicular to velocity at every instant. Hence path is a circle of radius r=mvqB.
The time period for circular path will be T=2πmqB
When θ is other than 0∘,180∘and90∘, velocity can be resolved into two components, one along →B and perpendicular to B.
v|/|=cosθ
v^=vsinθ
The v⊥ component gives circular path and v|/| givestraingt line path. The resultant path is a helical path. The radius of helical path
r=mvsinθqB
ich of helix is defined as P=v|/|T
P=(2imvcosθ)
p=2πmvcosθqB
A charged particle moves in a uniform magnetic field. The velocity of particle at some instant makes acute angle with magnetic field. The path of the particle will beAA stralight lineBA circleCA helix with uniform pitchDA helix with non-uniform pitch
If sin θ=725 and 90∘<θ<180∘, find the value of sec θ+tanθ.
View Solutionlf 2cos3θ=√3 and 0∘<θ<90∘ then the value of θ is
View SolutionIf θ be the angle between the vectors i+jandj+k, then θ is
View SolutionIf tan2θ.tan3θ=1 , where 0∘<θ<90∘ then the value of θ is
View Solutionif θ is angle between the velocity and acceleration of a particle moving on a circular path with decreasing speed, then
View Solution
