Question

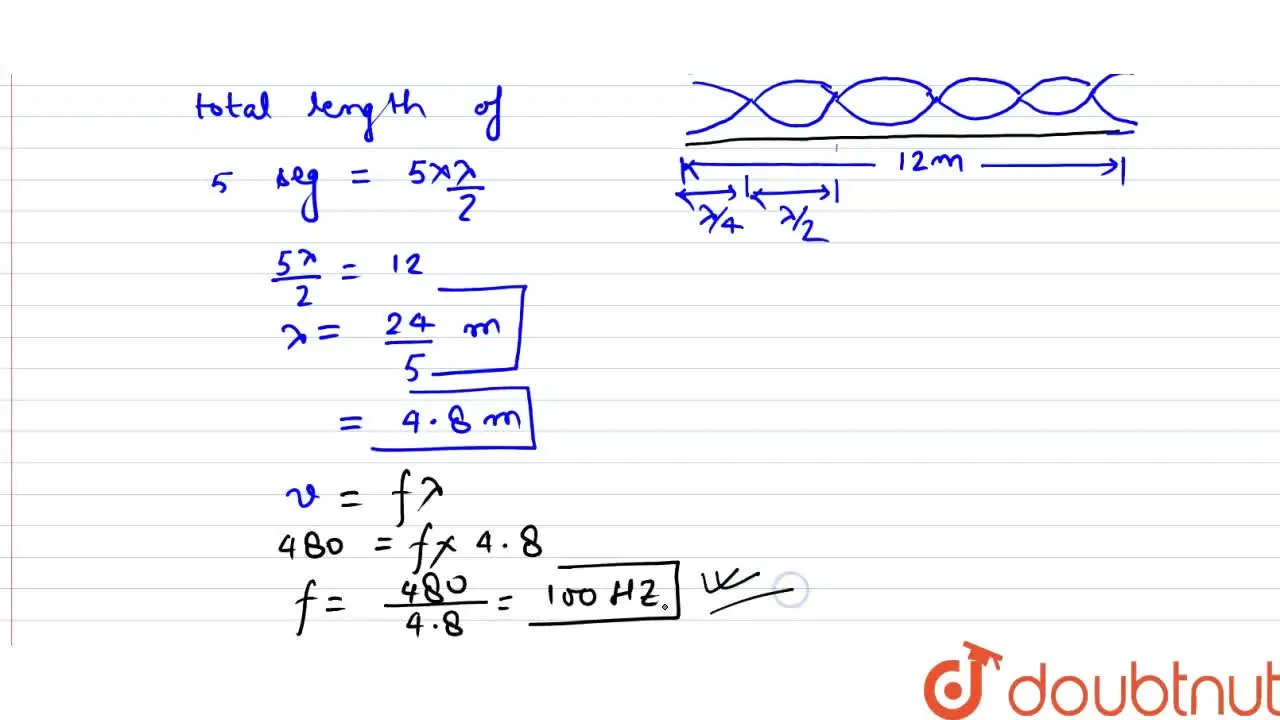
Standing waves are producedin a rubber tube ${\displaystyle 12m}$ long . If the tube vibrates in five segmens and the velocity of the wave is ${\displaystyle 480m/s}$, what is ${\displaystyle \left(a\right)}$ the wave length of the waves ${\displaystyle \left(b\right)}$ the frequency of the wave ?

Explanation

To solve the problem step by step, we will first determine the wavelength of the standing waves in the rubber tube and then calculate the frequency of the wave.

Step 1: Understand the relationship between segments and wavelength
In a standing wave, the number of segments (or loops) is related to the wavelength. Each segment corresponds to half a wavelength (λ/2).

Given that the tube vibrates in 5 segments, we can express the total length of the tube in terms of the wavelength:
\( \text{Length of the tube} = \text{Number of segments} \times \frac{\lambda}{2} \)
\( 12 \, \text{m} = 5 \times \frac{\lambda}{2} \)

Step 2: Solve for the wavelength (λ)
From the equation above, we can rearrange to find the wavelength:
\( 12 = \frac{5\lambda}{2} \)
Multiplying both sides by 2 to eliminate the fraction:
\( 24 = 5\lambda \)
Now, divide both sides by 5:
\( \lambda = \frac{24}{5} \, \text{m} = 4.8 \, \text{m} \)

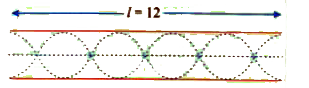
Step 3: Calculate the frequency (f)
We know the wave speed (v) and the wavelength (λ). The relationship between wave speed, frequency, and wavelength is given by:
\( v = f \lambda \)
Rearranging this formula to solve for frequency (f):
\( f = \frac{v}{\lambda} \)
Substituting the values we have:
\( v = 480 \, \text{m/s}, \quad \lambda = 4.8 \, \text{m} \)
\( f = \frac{480}{4.8} \)

Step 4: Perform the calculation for frequency
Calculating the frequency:
\( f = 100 \, \text{Hz} \)

Final Answers
(a) The wavelength of the waves is \(4.8 \, \text{m}\).
(b) The frequency of the wave is \(100 \, \text{Hz}\).