Two identical tall jars are filled with water to the brim. The first jar has a small hole on the side wall at a depth h/3 and the second jar has a small hole on the side wall at a depth of 2h/3, where h is the height of the jar. The water issuing out from the first jar falls at a distance R1 from the base and the water issuing out from the second jar falls at a distance R2 From the base. The correct relation between R1 and R2 is
R1>R2
R1<R2
R2=2×R1
R1=R2
The correct Answer is:D
R1=v1t1,R2=v2t2
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE - II (C.W) (POISEUILLE.S EQUATION)
4 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE - II (C.W) (TERMINAL VELOCITY)
2 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE - II (C.W) (EQUATION OF CONTINUITY, BERNOULLI.S THEOREM AND ITS APPLICATIONS)
1 Videos
- MATHEMATICAL REVIEW & PHYSICAL WORLDBOOK - NARAYNACHAPTER - MATHEMATICAL REVIEW & PHYSICAL WORLDEXERCISE - C.U.Q
13 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
Similar Questions
Water stands at a depth H in a tank whose side walls are vertical. A hole is made in one of the walls at a height h below the water surface. The stream of water emerging from the hole strikes the floor at a distance R from the tank, where R is given by
View SolutionA large open tank has two holes in the wall. One is a square hole of side L at a depth h from the top and the other is a circular hole of radius r at a depth 4h from the top. Whwn the tank is completely filled with water, the quantity of water flowing out per second from both the holes are the same. What is the value of r?
View Solution
Knowledge Check
A cylinderical vessel is filled with water up to height H. A hole is bored in the wall at a depth h from the free surface of water. For maximum range h is equal to
AH/4BH/2C3H/4DHA tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
A2H√3B√3H2C√3HD3H4A water bgarrel having water up to a depth d is placed on a table of height H. A small hole is made on the wall of the barrel at its bollom. If the stream of water comiong out of the hole falls on the ground at a horizontal distance r from the barrel, then the value of d is
A4Hr2B4Hr2Cr24HDH4r2
A cylindrical vessel filled with water to a hight H. A vessel has two small holes in the side, from which water iins rushing out horizontal and the two steams stike the ground at the same poin, if the lower hole Q Q is h height above the ground, then the height of hole P above the ground will be
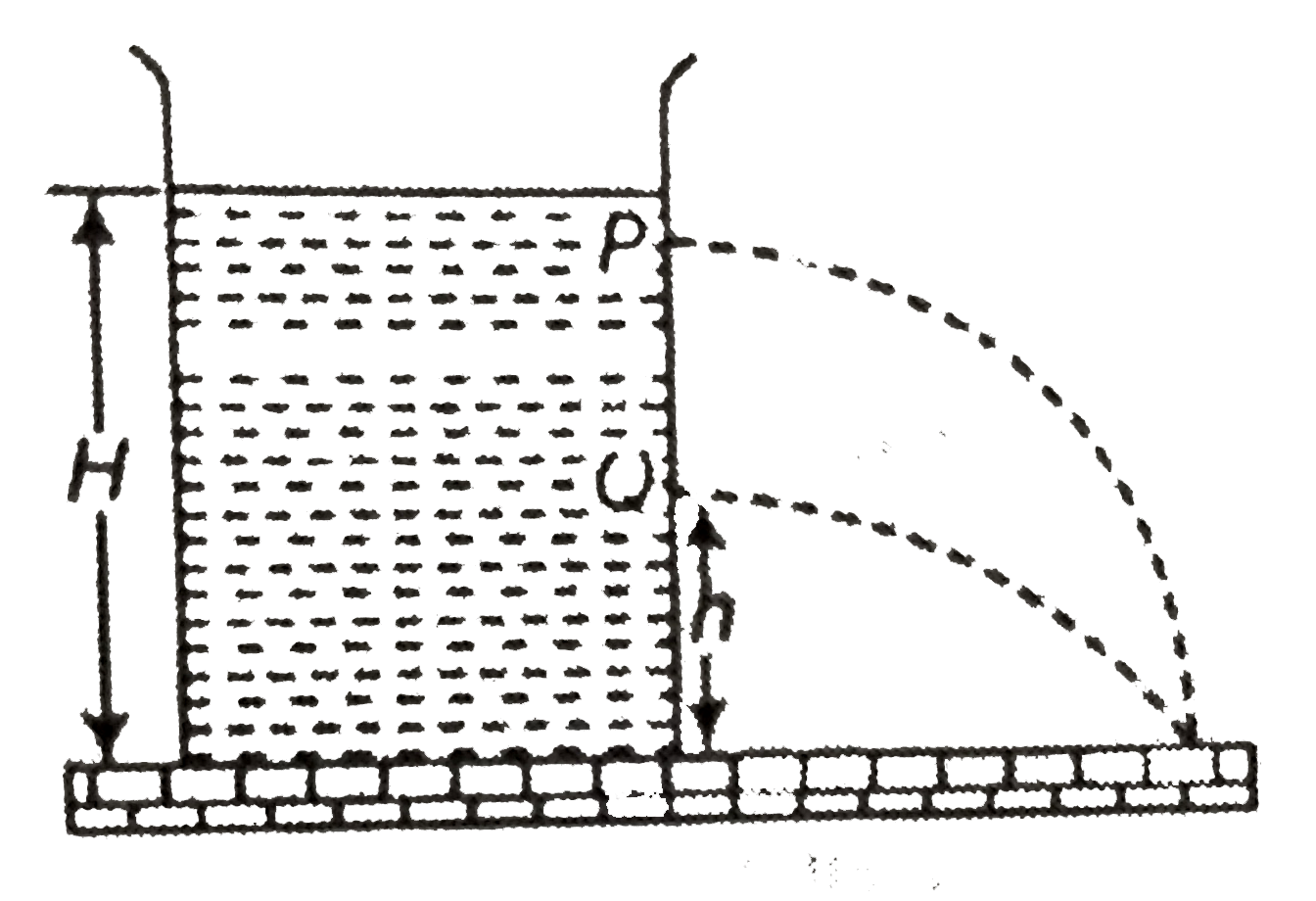
View SolutionA water barrel stands on a table of height h.If a small hole is punched in the side of the side of the barrel at its base. It is found that the resultant stream of water striks the ground at a horizontal distance R from the barrel. What is the depth of water in the berrel?
View SolutionIn a cylindrical water tank there are two small holes Q and P on the wall at a depth of h1 from the upper level of water and at a height of h2 from the lower end of the tank, respectively, as shown in the figure. Water coming out from both the holes strike the ground at the same point. The ratio of h1 and h2 is
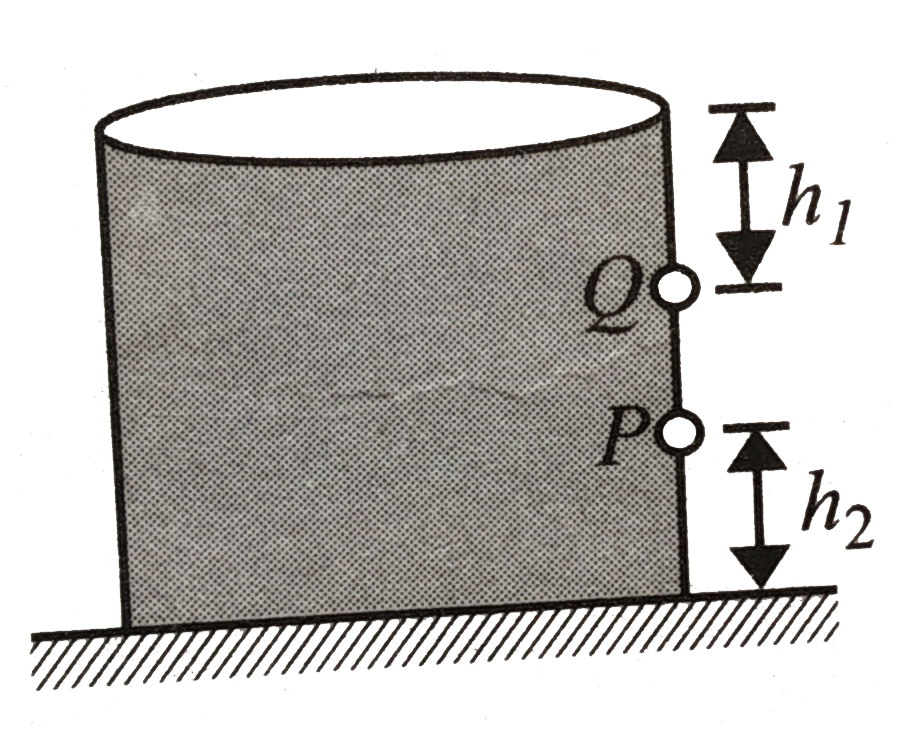
View SolutionThe height of the water in a tank is H. The range of the liquid emerging out from a hole in the wall of the tank at a depth 3H4 from the upper surface of water, will be
View SolutionA water tank placed on the floor has two small holes, pinched in the vertical wall, one above the other. The holes are 3.3cm and 4.7cm above the floor. If the jets of water issuing out from the holes hit the floor at the same point on the floor, then the height of water in the tank is
View Solution
