A rod of length 3m and its mass par unit length is directly propo

A rod of length 3 m has its mass per unit length directly proportional to distance x from one of its ends then its centre of gravity from that end will be at
A1.5 m
B2 m
C2.5 m
D3.0 m
If the linear density (mass per unit length) of a rod of length $3 m$ is proportional to $x$ , where $x$ , where $x$ is the distance from one end of the rod, the distance of the centre of gravity of the rod from this end is.
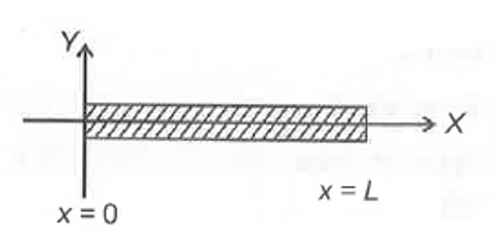
A2.5 m
B1 m
C1.5 m
D2 m
A rod of length L is of non uniform cross-section. Its mass per unit length varies linearly with distance from left end. Then its centre of mass from left end is at a distance. (Take linear density at left end=0).

A $\frac{L}{3}$
B $\frac{L}{6}$
C $\frac{L}{2}$
D $\frac{2 L}{3}$

A rod of length 3m and its mass par unit length is directly proportional to the distance x from its one end . The centre of gravity of the rod from that end will be at :-