Question

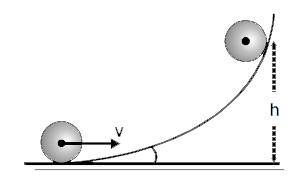
A uniform solid sphere rolls on a horizontal surface at $20m{s}^{-1}$ . It then rolls up in incline having an angle of inclination at ${30}^{\circ }$ with the horizontal . If the friction losses are negligible , the value of height h above the ground where the ball stops is

Answer

28m

Explanation

To solve the problem of a uniform solid sphere rolling up an incline, we will use the principle of conservation of energy. Here are the step-by-step calculations:

Step 1: Identify the initial and final states
- The sphere rolls on a horizontal surface with an initial velocity \( v = 20 \, \text{m/s} \).
- The sphere rolls up an incline with an angle of inclination \( \theta = 30^\circ \).
- We need to find the height \( h \) above the ground where the sphere stops.

Step 2: Write the conservation of energy equation
The total mechanical energy at the bottom (initial state) is equal to the total mechanical energy at the top (final state) when the sphere comes to a stop.

\( \text{Initial Kinetic Energy} = \text{Final Potential Energy} \)

Step 3: Calculate the initial kinetic energy
The sphere has both translational and rotational kinetic energy. The total kinetic energy \( KE \) is given by:

\( KE = KE_{\text{translational}} + KE_{\text{rotational}} \)

Where:
- \( KE_{\text{translational}} = \frac{1}{2} mv^2 \)
- \( KE_{\text{rotational}} = \frac{1}{2} I \omega^2 \)

For a solid sphere, the moment of inertia \( I \) is:

\( I = \frac{2}{5} m r^2 \)

And using the relationship \( \omega = \frac{v}{r} \), we have:

\( \omega^2 = \left(\frac{v}{r}\right)^2 = \frac{v^2}{r^2} \)

Thus, the rotational kinetic energy becomes:

\( KE_{\text{rotational}} = \frac{1}{2} \left(\frac{2}{5} m r^2\right) \frac{v^2}{r^2} = \frac{1}{5} mv^2 \)

Combining both kinetic energies:

\( KE = \frac{1}{2} mv^2 + \frac{1}{5} mv^2 = \left(\frac{5}{10} + \frac{2}{10}\right) mv^2 = \frac{7}{10} mv^2 \)

Step 4: Calculate the potential energy at height \( h \)
When the sphere reaches height \( h \), all kinetic energy is converted into potential energy:

\( PE = mgh \)

Step 5: Set the initial kinetic energy equal to the final potential energy
Using conservation of energy:

\( \frac{7}{10} mv^2 = mgh \)

Step 6: Cancel mass \( m \) from both sides
Assuming \( m \neq 0 \):

\( \frac{7}{10} v^2 = gh \)

Step 7: Substitute known values
Given \( v = 20 \, \text{m/s} \) and \( g = 10 \, \text{m/s}^2 \):

\( \frac{7}{10} (20)^2 = 10h \)

Calculating \( (20)^2 \):

\( \frac{7}{10} \times 400 = 10h \)

\( 280 = 10h \)

Step 8: Solve for height \( h \)
\( h = \frac{280}{10} = 28 \, \text{m} \)

Final Answer:
The height \( h \) above the ground where the ball stops is \( 28 \, \text{m} \).

---