Find moment of inertia of a sector cut from a disc of mass M, radius R and central angle θ
I=12MR2(θ2π)
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 19videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EVALUATE YOURSELF - 411videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-VI78videos
SYSTEM OF PARTICLES
- BOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)19videos
THERMAL PROPERTIES OF MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
The moment of inertia of a thin circular disc of mass M and radius R about any diameter is
AMR24BMR22CMR2D2MR2 - Question 2 - Select One
The moment of inertia of a thin circular disc of mass M and radius R about any diameter is
AMR24BMR22CMR2D2MR2 - Question 3 - Select One
Find moment of inertia of half disc of radius R2 and mass M about its centre. A smaller half disc of radius R1 is cut from this disc
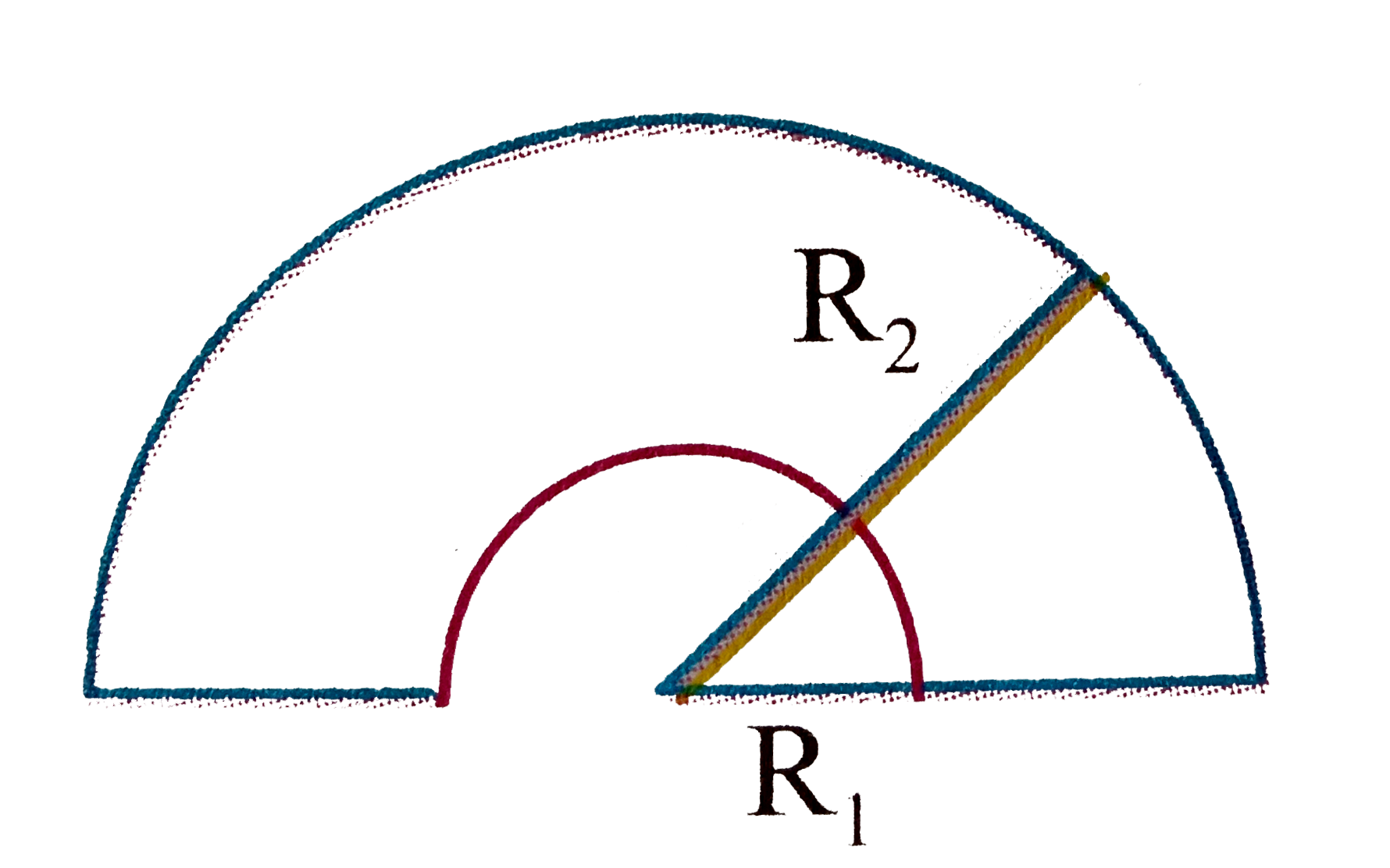 AM4(R21+R22)BM8(R21+R22)CM16(R21+R22)DM32(R21+R22)
AM4(R21+R22)BM8(R21+R22)CM16(R21+R22)DM32(R21+R22)
Find the moment of inertia of a section of circular disc of radius R about an axis perpendicualr to its plane as shown in the figure. The section has mass m and subtends an angle θ at the centre.
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View SolutionMoment of inertia of a disc of mass 'M' and radius 'R' about the diameter is
View SolutionThe moment of inertia of a uniform circular disc of mass M and of radius R about one of its diameter is
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View Solution

