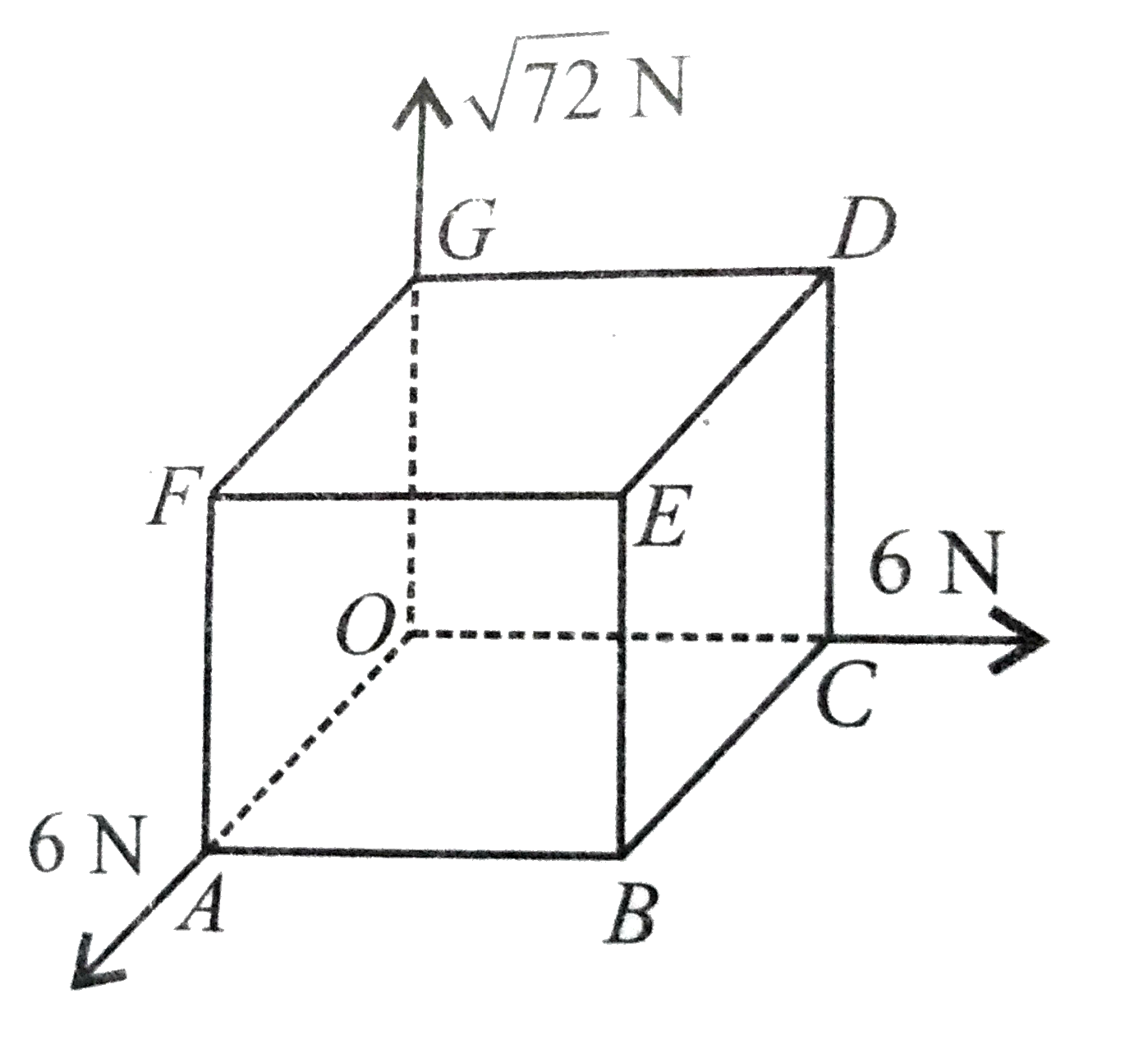
Three forces and are acting along and axes respectively on a mass at rest at the origin. The magnitude of its displacement after is .
50m
25m
60m
100m
The correct Answer is:D
Topper's Solved these Questions
- LAW OF MOTIONBOOK - NARAYNACHAPTER - LAW OF MOTIONEXERCISE - EXERCISE - II (C.W)(IMPULSE)
2 Videos
- LAW OF MOTIONBOOK - NARAYNACHAPTER - LAW OF MOTIONEXERCISE - EXERCISE - II (C.W)(OBJECTS SUSPENDED BY STRINGS AND APPARENT WEIGHT)
21 Videos
- LAW OF MOTIONBOOK - NARAYNACHAPTER - LAW OF MOTIONEXERCISE - EXERCISE - I (H.W)(CIRCULAR MOTION)
3 Videos
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)
52 Videos
- MATHEMATICAL REVIEW & PHYSICAL WORLDBOOK - NARAYNACHAPTER - MATHEMATICAL REVIEW & PHYSICAL WORLDEXERCISE - C.U.Q
13 Videos
Similar Questions
Knowledge Check
Forces of 4 N and 5 N are applied at origin along X-axis and Y-axis respectively. The resultant force will be
A√41N,tan−1(54)B√41N,tan−1(45)C−√41N,tan−1(54)D−√41N,tan−1(45)Five forces 2N,√3N,5N,√3and2N respectively act at a particel P as shown in figure.
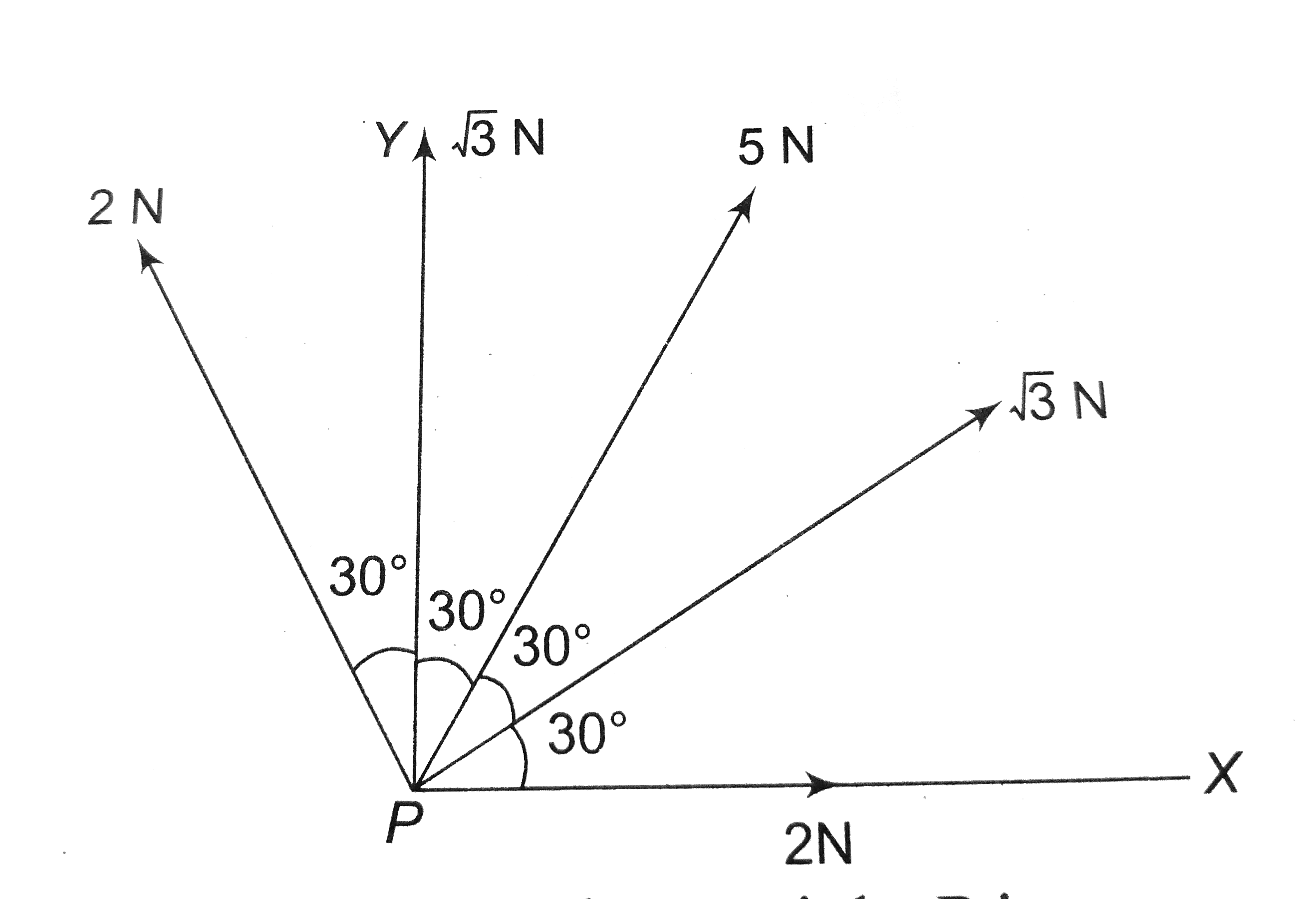
The resultant force on the particle P isA10N making angle 60∘ with X-axisB10N making angle 60∘ with Y-axisC20N along Y-axisDNone of theseThe two forces 2√2N and xN are acting at a point their resultant is perpendicular to ˆxN and having magnitude of √6N.The angle between the two forces and magnitude of x are.
Aθ=120∘,X=√2NBθ=30∘,X=√2NCθ=150∘,X=√3NDθ=150∘,X=√2N
Forces of 5√2 and 6√2N are acting on a body of mass 1000 kg at an angle of 60∘ to each other. Find the acceleration, distance covered and the velocity of the mass after 10 s.
View SolutionForces of √2N and 6√2N are acting on a body of mass 10 kg at an angle of 60∘ to each other . Find the acceleration , distance covered and velocity of the body after 10 second , if the body is initially at rest .
View SolutionThree ant P,Q and R are pulling a grain with forces of magnitude 6N,3√3N and 3√2N as shown in the figure. Find the magnitude of resultant force (in N ) acting on the grain .
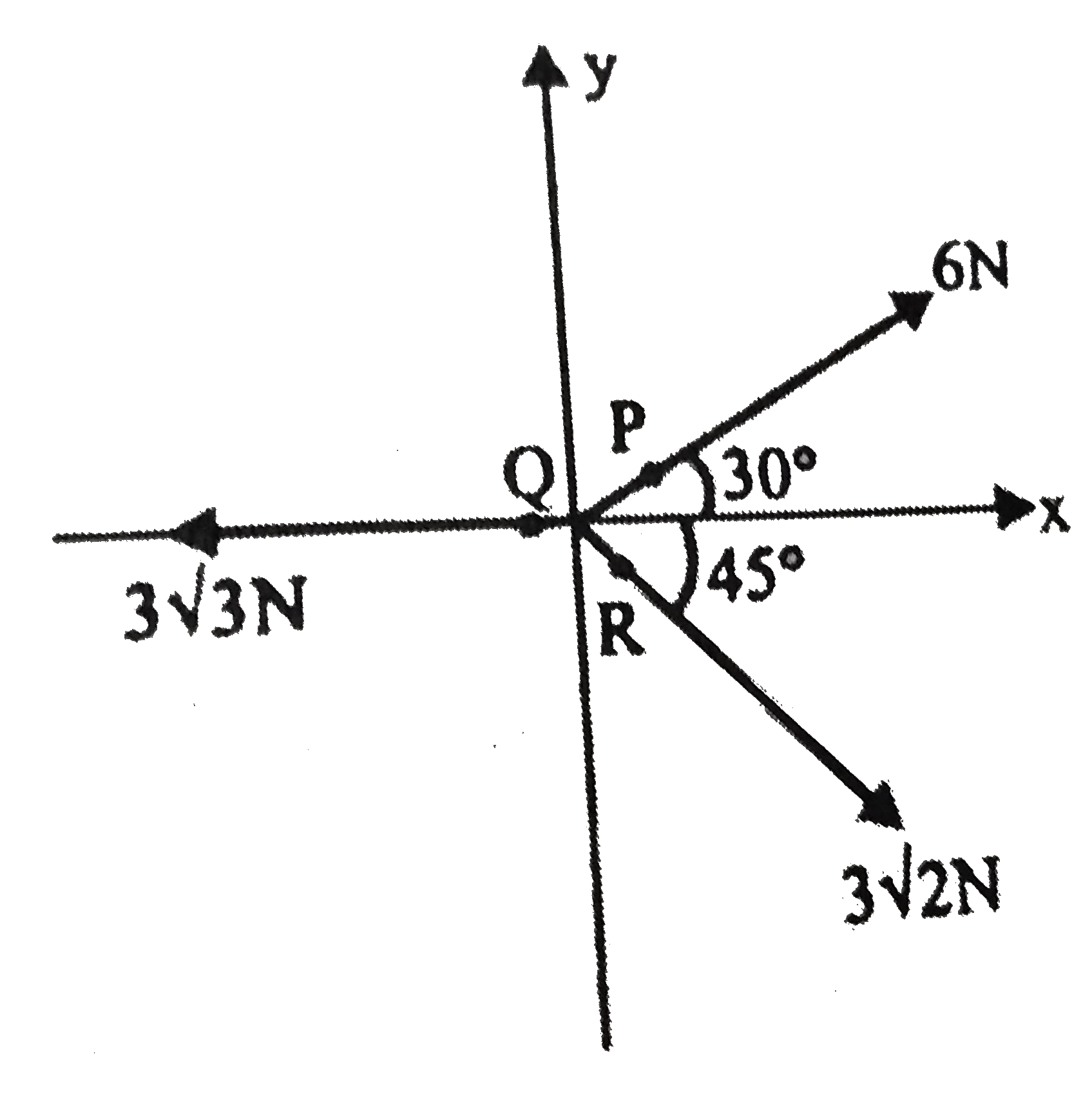
View SolutionForces X,Y and Z have magnitudes 10N5(√3−1)N and 5(√3+1)N, respectively. The forces Y and Z act in the same direction as shown in (figure) The resultant of X and Y and the resultant of X and Z have the same magnitudes. Find θ, the angle between X and Y.

View SolutionIf two forces of 5 N each are acting along X and Y axes, then the magnitude and direction of resultant is
View Solution