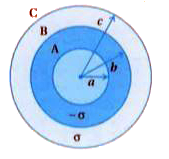
तीन सकेंद्रिये गोलिये कोशो की त्रिज्ये a,b व् c $(a < b < c)$ हैं तथा पृष्ठि आवेश घनत्व क्रमश : $σ - σ$ तथा $σ$ है। यदि $V_{A}, V_{B}$ तथा $V_{C}$ तीनो कोशो के विभव हैं, तब $c = a + b$ के लिए,

A

$V_{A} = V_{C} \neq V_{B}$

B

$V_{C} = V_{B} \neq V_{A}$

C

$V_{C} \neq V_{B} \neq V_{A}$

D

$V_{C} = V_{B} = V_{A}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

a त्रिजिया के गोले पर विभव तीनो गोलों के विभव का योग होगा। $∵$ आवेशित गोले की सतह पर विभव का सूत्र,
$V = \frac{1}{4 π ε_{0}} \frac{q}{R^{2}}$ (यहाँ, $ε_{0}$ = निर्वार्त की विधतुशीलता, q = सतह पर आवेश व R = त्रिजिया )
यहाँ: $V_{A} = \frac{1}{4 π ε_{0}} \frac{σ 4 π a^{2}}{a} - \frac{1}{4 π ε_{0}} \frac{σ 4 π b^{2}}{b} + \frac{1}{4 π ε_{0}} \frac{σ 4 π c^{2}}{c}$
= $\frac{σ}{ε_{0}} (a - b + c) = \frac{σ}{ε_{0}} (2 a)$ ( $∵$ c = a+b) ...(i)

$∵$ किसी गोलिये कोष के अंदर विभव नियत व सतह के समान होता है। इसी प्रकार त्रिजिया के गोले पर विभव,
$V_{B} = \frac{1}{4 π ε_{0}} \frac{σ 4 π a^{2}}{b} - \frac{1}{4 π ε_{0}} \frac{σ 4 π b^{2}}{b} + \frac{1}{4 π ε_{0}} . \frac{σ 4 π c^{2}}{c}$
$\frac{σ}{ε_{0}} (\frac{a^{2}}{b} - b + c) = \frac{σ}{ε_{0}} (\frac{a^{2}}{b} + a)$ ...(ii)
( $∵$ c = a + b)
तथा .c. त्रिजिया के गोले पर विभव ,
$V_{c} = \frac{1}{4 π ε_{0}} . \frac{σ 4 π a^{2}}{c} - \frac{1}{4 π ε_{0}} \frac{σ 4 π b^{2}}{c} + \frac{1}{4 π ε_{0}} . \frac{σ 4 π c^{2}}{c}$
$\frac{σ}{ε_{0}} (\frac{a^{2}}{c} - \frac{b^{2}}{c} + (c)) = \frac{σ}{ε_{0}} (2 a)$ ...(iii)
( $∵$ c = a + b)
तीनो समीकरणों से स्पष्ट है की प्रतिबंध c = a+b के लिए $V_{A} = V_{C} \neq V_{B}$ होगा।

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

NEET PREVIOUS YEAR-स्थिर वैद्युत विभव तथा धारिता-MCQs

Home
Ask Doubt
Profile