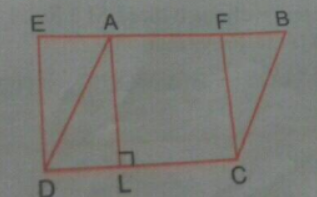
ABCD is a parallelogram and EFCD is a rectangle. Also, AL⊥DC. Prove that:
(i) ar (ABCD) = ar (EFCD)
(ii) ar (ABCD) = DC × AL
To solve the problem, we need to prove two statements about the areas of the parallelogram ABCD and the rectangle EFCD.
Given:
- ABCD is a parallelogram.
- EFCD is a rectangle.
- AL⊥DC (meaning AL is perpendicular to DC).
To Prove:
1. Area(ABCD)=Area(EFCD)
2. Area(ABCD)=DC×AL
1. Identify the bases and heights:
- In parallelogram ABCD, the base can be taken as CD and the height is the perpendicular distance from point A to line CD.
- In rectangle EFCD, the base is also CD and the height is the perpendicular distance from point E to line CD.
Hint: Identify the common base and the heights from the respective vertices to the base.
2. Use the property of parallelograms:
- According to the theorem, if two parallelograms lie on the same base and between the same parallels, their areas are equal.
Hint: Recall the theorem about the areas of parallelograms that share a base and are between the same parallels.
3. Conclusion for Part (i):
- Since both ABCD and EFCD share the base CD and lie between the same parallels (lines through AB and EF), we conclude:
Area(ABCD)=Area(EFCD)
Part (ii): Proving Area(ABCD)=DC×AL
1. Area of parallelogram formula:
- The area of a parallelogram is given by the formula:
Area=Base×Height
- Here, we can take DC as the base and AL as the height (since AL is perpendicular to DC).
Hint: Remember the formula for the area of a parallelogram and identify the base and height.
2. Substituting values:
- Therefore, we can write:
Area(ABCD)=DC×AL
3. Conclusion for Part (ii):
- This shows that the area of parallelogram ABCD can also be expressed as the product of its base DC and height AL:
Area(ABCD)=DC×AL
Final Conclusion:
We have successfully proved both parts of the question:
1. Area(ABCD)=Area(EFCD)
2. Area(ABCD)=DC×AL
- ABCD is a parallelogram.
- EFCD is a rectangle.
- AL⊥DC (meaning AL is perpendicular to DC).
1. Area(ABCD)=Area(EFCD)
2. Area(ABCD)=DC×AL
- In parallelogram ABCD, the base can be taken as CD and the height is the perpendicular distance from point A to line CD.
- In rectangle EFCD, the base is also CD and the height is the perpendicular distance from point E to line CD.
- According to the theorem, if two parallelograms lie on the same base and between the same parallels, their areas are equal.
- Since both ABCD and EFCD share the base CD and lie between the same parallels (lines through AB and EF), we conclude:
Area(ABCD)=Area(EFCD)
- The area of a parallelogram is given by the formula:
Area=Base×Height
- Here, we can take DC as the base and AL as the height (since AL is perpendicular to DC).
- Therefore, we can write:
Area(ABCD)=DC×AL
- This shows that the area of parallelogram ABCD can also be expressed as the product of its base DC and height AL:
Area(ABCD)=DC×AL
We have successfully proved both parts of the question:
1. Area(ABCD)=Area(EFCD)
2. Area(ABCD)=DC×AL
Topper's Solved these Questions
- AREAS OF PARALLELOGRAMS AND TRIANGLESBook:NCERTChapter:AREAS OF PARALLELOGRAMS AND TRIANGLESExercise:Exercise 9.2
Explore 6 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLESBook:NCERTChapter:AREAS OF PARALLELOGRAMS AND TRIANGLESExercise:EXERCISE 9.4
Explore 8 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLESBook:NCERTChapter:AREAS OF PARALLELOGRAMS AND TRIANGLESExercise:EXERCISE 9.1
Explore 1 Video
- CIRCLESBook:NCERTChapter:CIRCLESExercise:EXERCISE 10.2
Explore 2 Videos
Similar Questions
In Figure, ABCD is a parallelogram and EFCD is a rectangle. Also AL⊥DC. Prove that : ar(ABCD)=ar(EFCD) ar(ABCD)=DC×AL
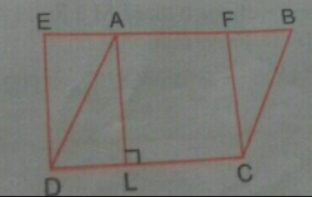
View SolutionIn the following figure, ABCD is a parallelogram and EFCD is a rectangle. Also, AL⊥DC. Prove that
(i) ar(ABCD)=ar(EFCD)
(ii) ar(ABCD)=DC×AL.
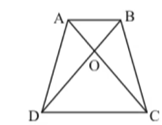
View SolutionABCD is a parallelogram whose diagonals intersect at O. If P is any point on BO, prove that: ar (ADO)=AR (CDO) ar ( ABP)= ar ( CBP)
View SolutionIf ABCD is a parallelogram, then prove that ar(△ABD)=ar(△BCD)=ar(△ABC)=ar(△ACD)=12ar(∣∣gmABCD)
View SolutionIn Figure, ABCD is a trapezium in which AB∣∣CD . Prove that: ar(AOD)=ar(BOC).
View SolutionIn a parallelogram ABCD, the bisector of ∠A also bisects BC at X Prove that AD=2AB
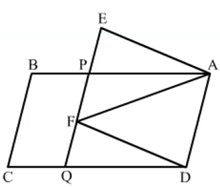
View SolutionIn Figure, ABCDandAEFD are two parallelograms? Prove that: ar(APE):ar(PFA)=ar(QFD):ar(PFD)
View SolutionIn a ABC , If LandM are points ABandAC respectively such that LMparallelBC
Prove that: ar(ABM)=ar(ACL)
View SolutionIn Figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that: ar (ACB)=ar (ACF) ar (AEDF)=ar (ABCDE)
View SolutionA point D is taken on the side BC of a ABC such that BD=2DC. Prove that ar ( ABD)= 2 ar ( ADC)
View Solution