Question

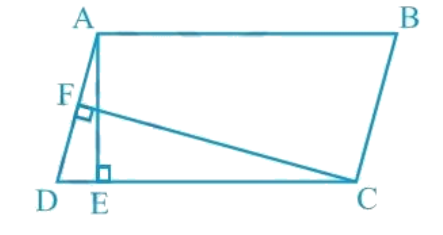
$ABCD$ is a parallelogram, $AE\perp DC$and $CF\perp AD$. If $AB=16cm$, $AE=8cm$ and $CF=10cm$, find $AD$.

Explanation

To solve the problem, we will follow these steps:

Step 1: Write down the given information.
We have:
- \( AB = 16 \, \text{cm} \)
- \( AE = 8 \, \text{cm} \)
- \( CF = 10 \, \text{cm} \)
- \( AE \perp DC \) (AE is perpendicular to DC)
- \( CF \perp AD \) (CF is perpendicular to AD)

Step 2: Understand the area of the parallelogram.
The area of a parallelogram can be calculated using the formula:
\( \text{Area} = \text{Base} \times \text{Height} \)
We can calculate the area of parallelogram \( ABCD \) using two different bases and their corresponding heights.

Step 3: Calculate the area using base \( DC \).
Using \( DC \) as the base and \( AE \) as the height:
\( \text{Area} = DC \times AE \)
Since \( DC = AB \) (opposite sides of a parallelogram are equal):
\( DC = 16 \, \text{cm} \)
Thus, the area can be expressed as:
\( \text{Area} = 16 \times 8 = 128 \, \text{cm}^2 \)

Step 4: Calculate the area using base \( AD \).
Now, using \( AD \) as the base and \( CF \) as the height:
\( \text{Area} = AD \times CF \)
Substituting \( CF = 10 \, \text{cm} \):
\( \text{Area} = AD \times 10 \)

Step 5: Set the two area expressions equal to each other.
Since both expressions represent the area of the same parallelogram, we can set them equal:
\( 128 = AD \times 10 \)

Step 6: Solve for \( AD \).
To find \( AD \), we rearrange the equation:
\( AD = \frac{128}{10} = 12.8 \, \text{cm} \)

Conclusion:
The length of \( AD \) is \( 12.8 \, \text{cm} \).

---