Solve the inequalities graphically in two-dimensional plane: 3y−5x<30
Video Solution
Text Solution
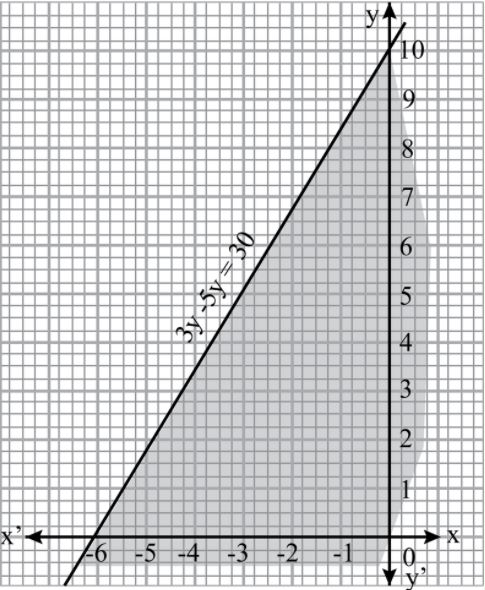
Given inequality is 3y−5x<30.
Line that is dividing the region will be 3y−5x=30
When y=0,x=−6
So, x-intercept is (−6,0).
When x=0,y=10
So, y-intercept is (0,10).
Using these two intercepts, we can draw the line graphically.
Please refer to video for the graph.
If, we take point (0,0) in the shaded region, our inequality becomes,
Please refer to video for the graph.
3(0)−5(0)<30⇒0<30,which is true.
So, the shaded region in the graph is the required region.
|
Topper's Solved these Questions
Similar Questions
Solve the inequalities graphically in two-dimensional plane: 3y−5x<30
View SolutionSolve the inequalities graphically in two-dimensional plane: y<2
View SolutionSolve the inequalities graphically in two-dimensional plane: x+y<5
View SolutionSolve the inequalities graphically in two-dimensional plane: 2x−3y>6
View SolutionSolve the inequalities graphically in two-dimensional plane: 3x+4y≤12
View SolutionSolve the inequalities graphically in two-dimensional plane: y+8≥2x
View SolutionSolve the inequalities graphically in two-dimensional plane: −3x+2y≥−6
View SolutionSolve the inequalities graphically in two-dimensional plane: x−y≤2
View SolutionSolve the inequalities graphically in two-dimensional plane: x>−3
View SolutionSolve the inequalities graphically in two-dimensional plane: 2x+y≥6
View Solution
