Solve the inequalities graphically in two-dimensional plane: 3x+4y≤12
Video Solution
Text Solution
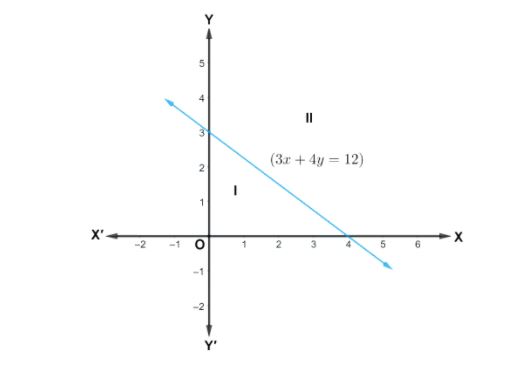
The graphical representation of 3x + 4 y = 12 is given in the figure below.
This line divides the XY plane into two half-planes, I and II.
Select a point (not on the line), which lies in one of the half-planes, to determine whether the point satisfies the given inequality or not.
We select the point as (0, 0).
It is observed that,
3(0)+4(0)≤12or0≤12, which is true
Thus, the solution region of the given inequality is the shaded half plane I, including the points on the line
This can be represented as follows:
|
Topper's Solved these Questions
Similar Questions
Solve the inequalities graphically in two-dimensional plane: y<2
View SolutionSolve the inequalities graphically in two-dimensional plane: x+y<5
View SolutionSolve the inequalities graphically in two-dimensional plane: 3x+4y≤12
View SolutionSolve the inequalities graphically in two-dimensional plane: 2x−3y>6
View SolutionSolve the inequalities graphically in two-dimensional plane: 2x+y≥6
View SolutionSolve the inequalities graphically in two-dimensional plane: x−y≤2
View SolutionSolve the inequalities graphically in two-dimensional plane: x>−3
View SolutionSolve the inequalities graphically in two-dimensional plane: 3y−5x<30
View SolutionSolve the inequalities graphically in two-dimensional plane: y+8≥2x
View SolutionSolve the inequalities graphically in two-dimensional plane: −3x+2y≥−6
View Solution
