A man running at a speed of 5 kmph finds that the rain falls vertically. When he stops running, he finds that the rain is falling at an angle of 60° with the horizontal. The velocity of rain with respect to running man isSolution in Telugu
5√3km/h
5√32km/h
4√32km/h
5√3km/h
The correct Answer is:D
Consider the situational diagram as shown below.
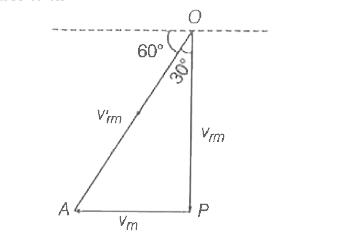
In ΔOAP,tan30∘=vmvrm=Velocity of manVelocity of rain with respect to man
⇒vrm=51/√3=5√3km/h
Topper's Solved these Questions
Similar Questions
Match the entries in List I with those in List II.

View SolutionAlbert Einstein was confermed with the Nobel prize in physics for his work on
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View SolutionA man running on a horizontal road at 6 km//h finds the rain falling vertically. He doubles his speed and find that the raindrops make an angle 37^(@) with the vertical. Find the velocity of rain with respect to the ground.
View SolutionTo a man walking at 5kmph rain appears to fall vertically, and when he doubles his speed it appears to make an angle of 30∘ with the vertical. Find the actual velocity of the rain. In what direction will rain appear to fall if the man suddenly turns around when his speed is 5 kmph?
View SolutionA stationary man observes that the rain is falling vertically downwards. When he starts running a velocity of 12kmh−1, he observes that the rain is falling at an angle 60∘ with the vertical. The actual velocity of rain is
View SolutionA man running at a speed of 5 km/h finds that the rain is falling vertically. When the stops running, the finds that the rain is falling at an angle of 60∘ with the horizontal. The velocity of rain with respect to running man is
View SolutionA man running at a speed of 5 kmph finds that the rain falls vertically. When he stops running, he finds that the rain is falling at an angle of 60° with the horizontal. The velocity of rain with respect to running man is
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View SolutionA man is travelling at 10.8 kmph in a topless car on a rainy day. He holds an umbrella at an angle of 37∘ with the vertical to protect himself from rain which is falling vertically downwards. What is the velocity of the rain drop ?
View SolutionA man running on a horizontal road at 8kmph finds that rain is falling vertically. He increases the speed to 12kmphr and find that the drops make angle 30∘ with the vertical.
View SolutionA staionary man observes that the rain is falling vertically downward. When he starts running with a velocity of 12 km/h observes that the rains is falling at an angle 60∘ with the vertical. The actual velocity of rain is
View SolutionA man is travelling at 10.8 kmph in a topless can on a raining day. He holds an umbrella at an angle 37^(@) with the vertical so that he does not wet. If rain drops fall vertically downwards, find the rain velocity?
View SolutionA stationary man observes that the rain is falling vertically downwards. When he starts running a velocity of 12kmh−1, he observes that the rain is falling at an angle 60∘ with the vertical. The actual velocity of rain is
View SolutionA man starts running along a straight road with uniform velocity observes that the rain is falling vertically downward. If he doubles his speed, he finds that the rain is coming at an angle θ to the vertical. The velocity of rain with respect to the ground is :
View Solution5 km/h की चाल से दौड़ने वाला एक व्यक्ति पाता है कि वर्षा ऊर्ध्वाधर रूप से हो रही है। जब वह दौड़ना बंद कर देता है, तो वह पाता है कि वर्षा क्षैतिज के साथ 60∘ के कोण पर हो रही है। दौड़ने वाले व्यक्ति के सापेक्ष वर्षा का वेग है:
View Solution
