A uniform rod of length 1m lies on the smooth part of a horizontal surface as shown. Calculate the minimum veloctiy V0 in m/s so that the rod can reach the rough surface completely. Take g=10m/s2 and coefficient of friction between the rod and the rough surface as 0.1.
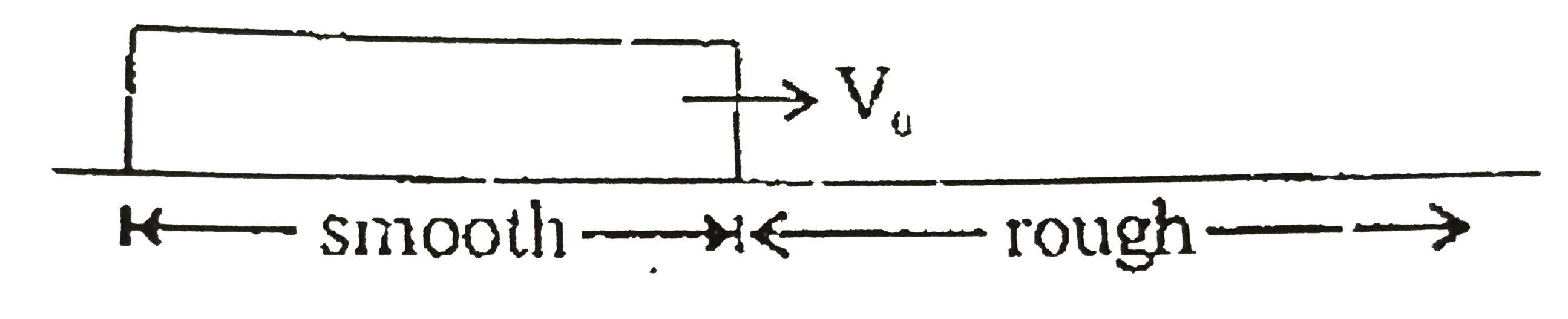
Work done by friction
∫0LμmgxLdx=μmgL2
∴12mv20=μmgL2
⇒v0=√μgL=1m/s
Topper's Solved these Questions
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (A)
Explore 32 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART (B)
Explore 3 Videos
- TEST PAPERSBook:BANSALChapter:TEST PAPERSExercise:PHYSICS PART- C
Explore 4 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
A uniform rod of length L and mass M has been placed on a rough horizontal surface. The horizontal force F applied on the rod is such that the rod is just in the state of rest. If the coefficient of friction varies according to the relation μ =Kx where K is a +ve constant. Then the tension at mid point of rod is?
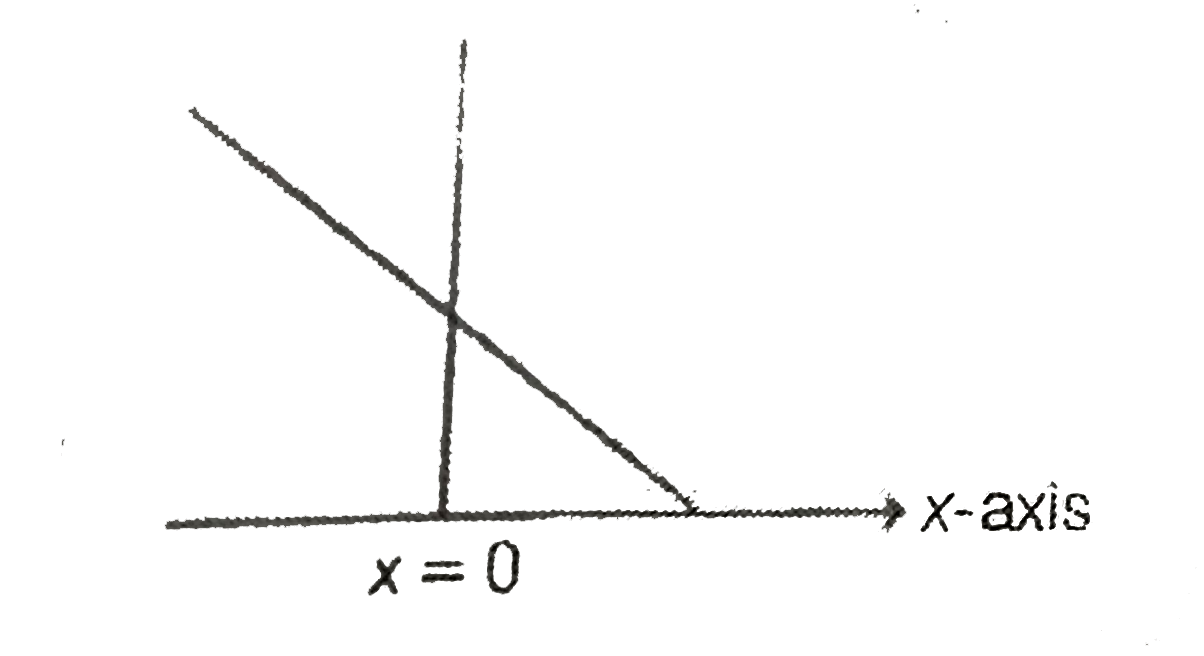
View SolutionA uniform rod of length l is kept vertically on a rough horizontal surface at x = 0 . It is rotated slightly and released . When the rod finally falls on the horizontal surface , the lower end will remain at
View Solution
Knowledge Check
A block slides with a velocity of 10 m/s on a rough horizontal surface. It comes to rest after covering a distance of 50 metre. If g is 10m/s2, then the coefficient of dynamic friction between the block and the surface is
A0.1B1C10D5A uniform ring placed on a rough horizontal surface is given a sharp impulse as shown in the figure-5.130. As a consequence, it acquires a linear velocity of 2m/s. If coefficient of friction between the ring and the horizontal surface is 0.4 :
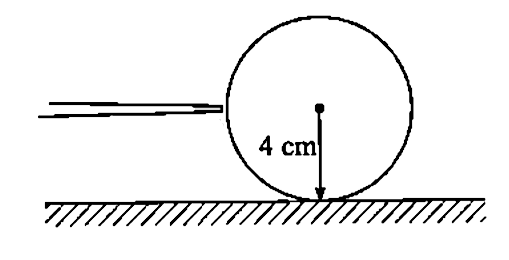 ARing wil lstart pure rolling afler O.25sBWhen ring will start pure rolling its .velocity is 1m//sCAfter 0.5s from impulse its velocity is 1m//s.DAfter0.125 s from impulse its velocity is 1m//s.
ARing wil lstart pure rolling afler O.25sBWhen ring will start pure rolling its .velocity is 1m//sCAfter 0.5s from impulse its velocity is 1m//s.DAfter0.125 s from impulse its velocity is 1m//s.A uniform rod of mass m and length l is at rest on smooth horizontal surface. An impulse P is applied to end B as shown.

Distance travelled by the centre of the rod, while rod rotates by 90∘Aπl7Bπl4Cπl12Dπl5
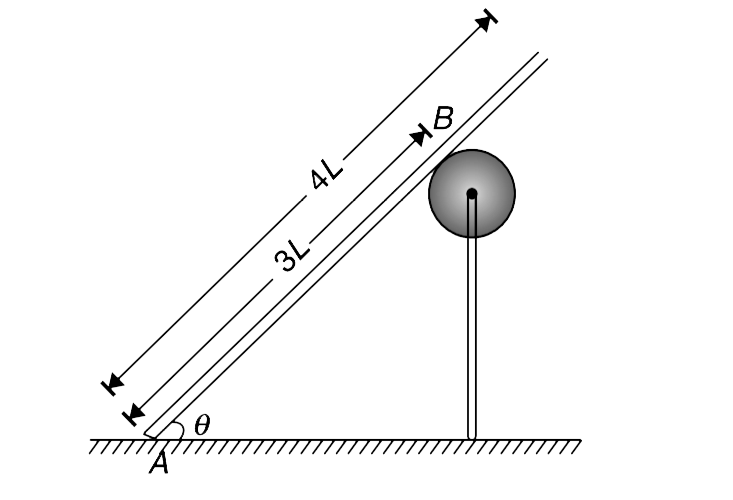
A uniform rod has mass M and length 4L. It rests in equilibrium with one end on a rough horizontal surface at A. At point B, at a distance 3L from A, it is supported by a fixed smooth roller. The rod just remains in equilibrium when θ=30∘
(a) Find the normal force applied by the horizontal surface on the rod at point A.
(b) Find the coefficient of friction between the rod and the surface.
View SolutionA uniform ring of mass m is placed on a rough horizontal fixed surface as shown in the figure. The coefficient of friction between the left part of the ring and left part of the horizontal surface is μ1=0.6π and between right half and the surface is μ2=0.2π. At the instant shown, now the ring has been imparted an angular velocity in clockwise sense in the figure shown. At this moment magnitude of acceleration of centre O of the ring (in m/s2) is (take g=10m/s2)
View SolutionA body of mass 10 kg lies on a rough horizontal surface. When a horizontal force of F newtons acts on it, it gets an acceleration of 5m/s2. And when the horizontal force is doubled, it gets an acceleration of 18m/s2. The coefficient of friction between the body and the horizontal surface is
View SolutionA uniform rod of mass m and length L is at rest on a smooth horizontal surface. A ball of mass m, moving with velocity v0, hits the rod perpendicularly at its one end and sticks to it. The angular velocity of rod after collision is

View SolutionA uniform rod of mass m and length l is at rest on smooth horizontal surface. An impulse P is applied to end B as shown.
The point about which rod rotates just after impulse being applied is located at
View Solution
