A man is standing on incline which makes θ with horizontal and rain is falling vertically with respect to man. Now man starts walking along the incline. Mark the correct option
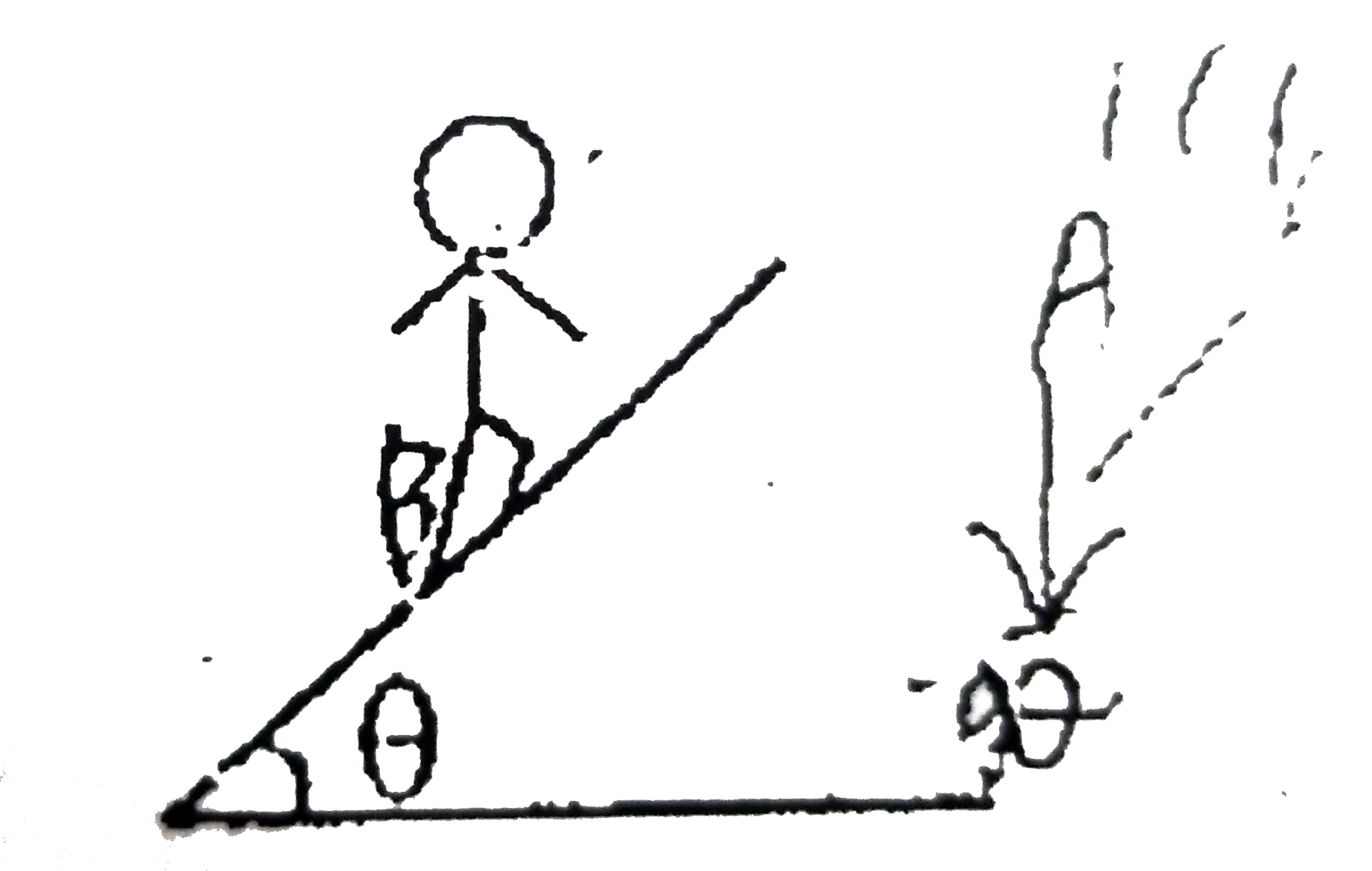
If man walks up the incline, rain may appear to come horizontally
If man walks down the incline, rain may appear to come horizontally
No matter whether man walks up or down, rain can never appear to come horizontally.
If man walks (up or down) along incline rain may appear to come along the incline.
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A man is moving due east with a speed 1 km/hr and rain is falling vertically with a speed √3 km/hr. At what angle from vertical the man has to hold his umbrella to keep the rain away. Also find the speed of rain drops w.r.t. man.
View SolutionA man moving with a velocity of 5m/s on a horizontal road observes that raindrops fall at an angle of 45∘ with the vertical. When he moves with a velocity of 16m/s along an inclined plane, which is inclined at 30∘ with the horizontal , he observes raindrops falling vertically downward as shown in the figure. Find the actual velocity of the raindrops.
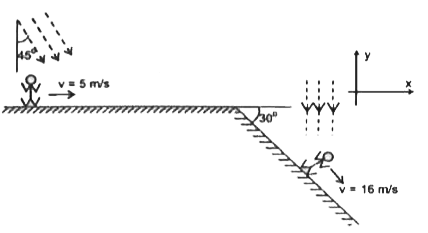
View Solution
Knowledge Check
A man is standing on a plank which is placed on smooth horizontal surface. There is sufficient friction between the feet of man and plank. Now man starts running over plank, correct statement is /are
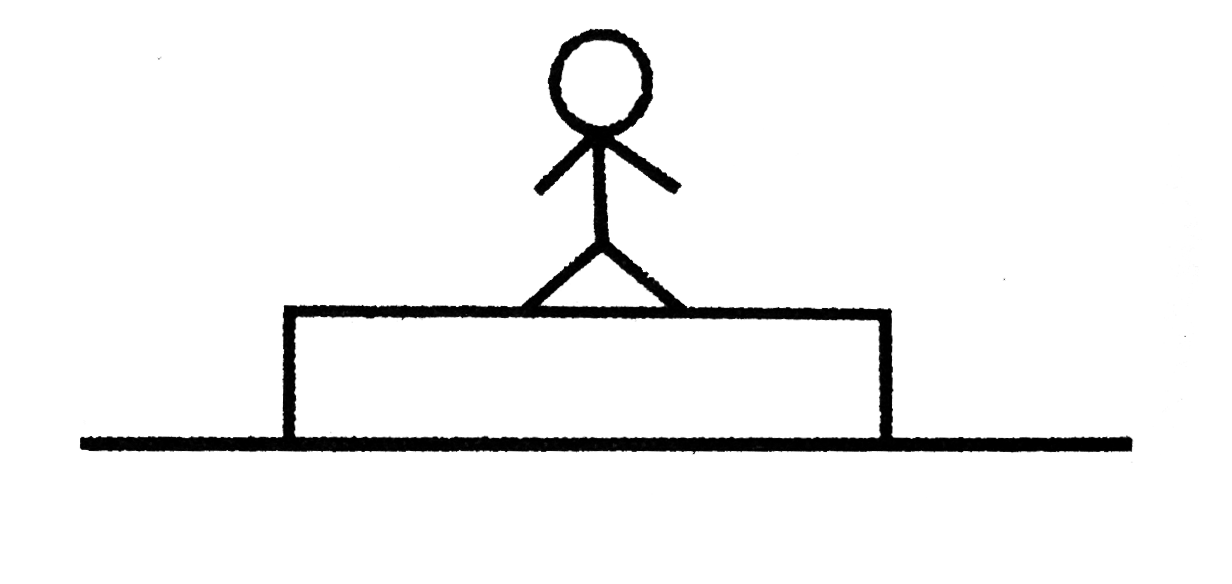 AWork done by friction on the man with respect to the ground is negativeBWork done by friction on the man with respect to the ground is positiveCWork done by friction on the plank with respect to the ground is positive.DWork done by friction on the man with respect to the plank is zero.
AWork done by friction on the man with respect to the ground is negativeBWork done by friction on the man with respect to the ground is positiveCWork done by friction on the plank with respect to the ground is positive.DWork done by friction on the man with respect to the plank is zero.A man is standing on a plank which is placed on smooth horizontal surface. There is sufficient friction between feet of man and plank. Now man starts running over plank, correct statement is/are
 AWork done by friction on man with respect to ground is negative.BWork done by friction on man with respect to ground is positiveCWork done by friction on plank with respect to groundDWork done by friction on man with respect to plank is zero.
AWork done by friction on man with respect to ground is negative.BWork done by friction on man with respect to ground is positiveCWork done by friction on plank with respect to groundDWork done by friction on man with respect to plank is zero.A man standing on a inclined plain observes rain is falling vertically. When he starts moving down the inclined plain with velocity v=6m/s observes rain hitting him horizontally.

The velocity of rain with respect to the man when he is moving down isA3m/sB3√3m/sC4m/sDnone of these
A man is walking due west with a velocity 3km/h and rain appears to be falling vertically with a velocity 4km/h. Find the velocity of rain with respect to the ground.
View SolutionA man of mass 50kg us standing on a 100kg plank kept on a fricitonless horizontal floor. Initially both are at rest. If the man starts walking on the plank with speed 6m/s towards right relative to the plank, then amount of muscle energy spent by the man is
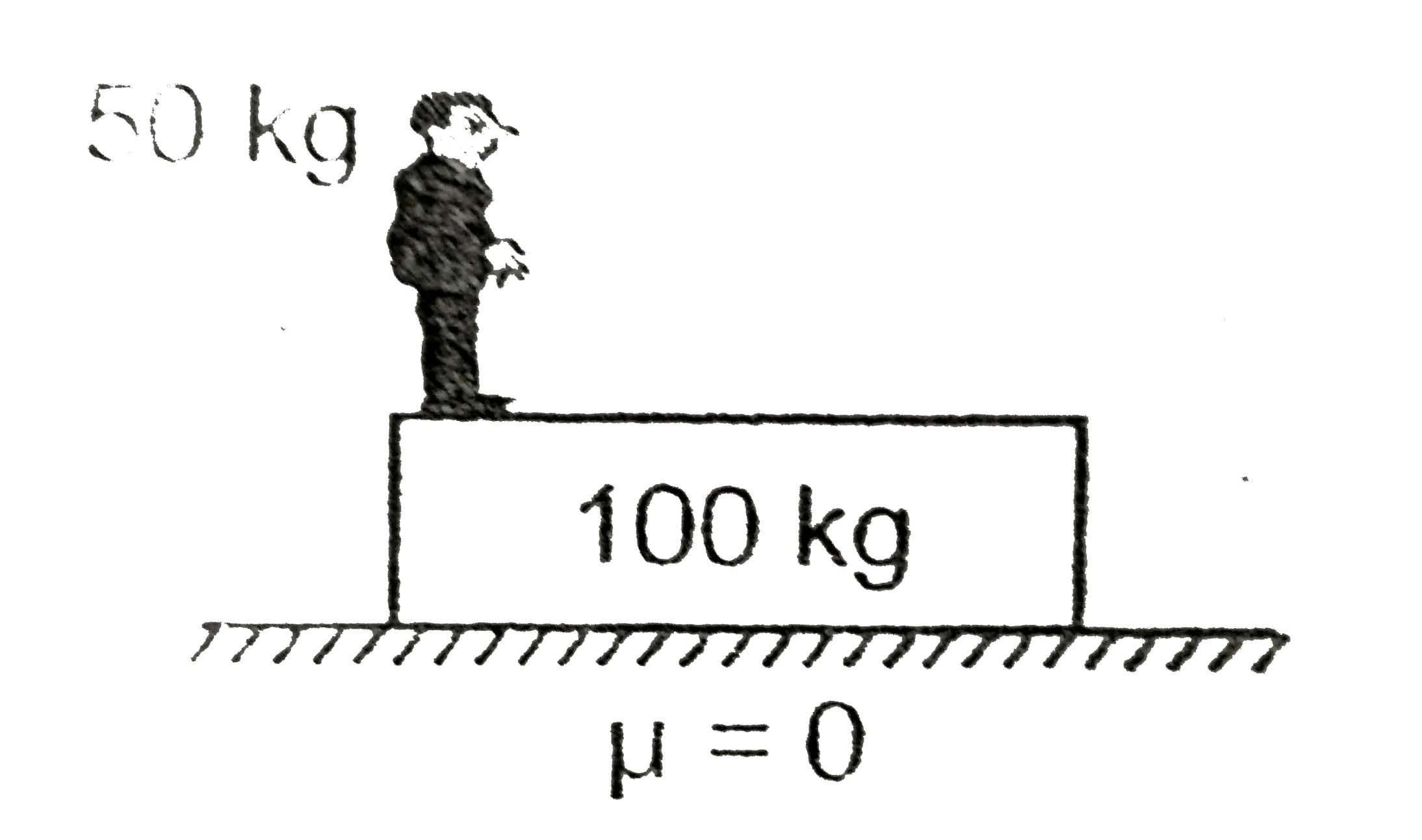
View SolutionA man standing on a inclined plain observes rain is falling verticaly. When he starts moving down the inclined plain with velocity v=6m/s observes rain hitting him horizontally.

The actual velocity of rain is
View SolutionA man is standing on a road and observes that rain is failing at angle 45∘ with the vertical. The man starts running on the road with constant acceleration 0.5m/s2. After a certain time from the start of the motion, it appears to him that rain is still falling at angle 45∘ with the vertical, with speed 2√2m/s. Motion of the man is in the same vertical plane in which the rain is falling. Then which of the following staement(s) are true.
View SolutionWhen a man moves down the inclined plane with a constant speed 5ms−1 which makes an angle of 37∘ with the horizontal, he finds that the rain is falling vertically downward. When he moves up the same inclined plane with the same speed, he finds that the rain makes an angle θ=tan−1(78) with the horizontal. The speed of the rain is
View Solution

