Question

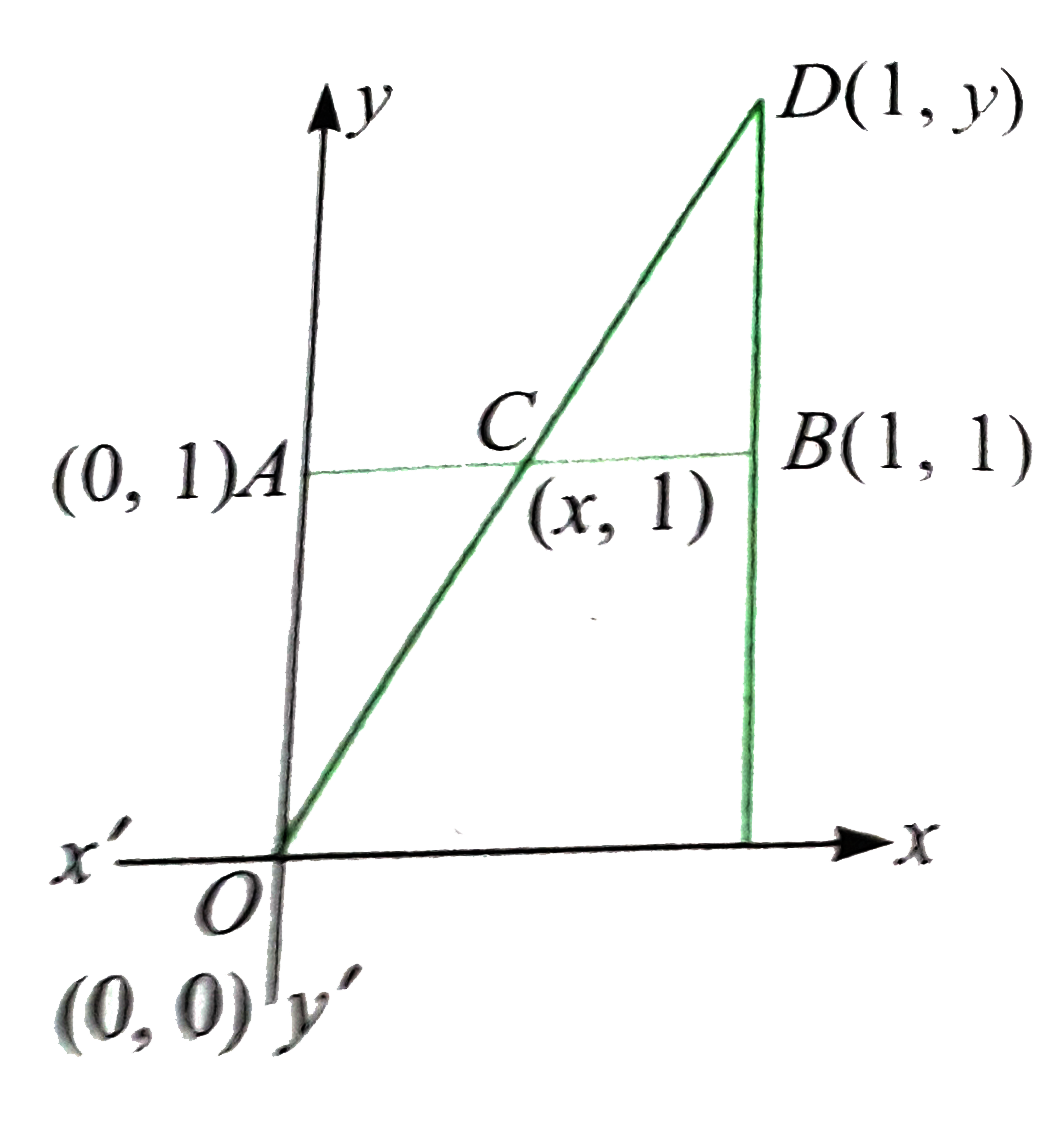
Consider the points $O(0,0),A(0,1)$, and $B(1,1)$ in the x-y plane. Suppose that points $C(x,1)\text{and}D(1,y)$ are chosen such that $0<x<1$. And such that $O,C,\text{and}D$ are collinear. Let the sum of the area of triangles OAC and BCD be denoted by S. Then which of the following is/are correct?.

Options

A. Minimum value of S is irrational lying in `(1//3,1//2)` .
B. Minimum value of S is irrational in `(2//3,1)`.
C. The value of x for the minimum value of S lies in `(2//3,1)`.
D. The value of x for the minimum values of S lies in `(1//3,1//2)`.

Answer

A::C

Explanation

`S="Area of" Delta OAC+ "Area of" DeltaBCD`
`=(1.x)/(2)+(1-x)(y-1)/(2),0 lt x lt 1`
`therefore S=(x)/(2)-(x-1)(y-1)/(2)` (1)
Now,`Delta`'s CBD and OCA are similar. Therfore,
`(y-1)/(1)=(1-x)/(x)`
`therefore S=(x)/(2)-((x-1){(1//x)-1})/(2)`
`=(x)/(2)+(x-1)^(2)/(2x)`
`=(x^2+(x-1)^1)/(2x)=((2x^2-2x+1))/(2x)`
`=x+(1)/(2x)-1=(sqrtx-(1)/(sqrt(2x)))^(2)-1+sqrt(2)`
Therefore, A is minimum if `sqrt(x)=1//sqrt(2x)`. therefore , `x=1//sqrt(2)`
which lies in `(2//3,1)` and `A_("min")=sqrt(2)` which lies in `(1//3,1//2)`.