Given A(0,0) and B(x,y) with x $\in$ (0,1) and y>0. Let the slope of line AB be $m_{1}$ . Point C lies on line $x = 1$ such that the slope of BC is equal to $m_{2}$ where $0 < m_{2} < m_{1}$ If the area of triangle ABC can be expressed as $(m_{1} - m_{2}) f (x)$ then the largest possible value of x is

$1 / 2$

$1 / 4$

$1 / 8$

Video Solution

Text Solution

Verified by Experts

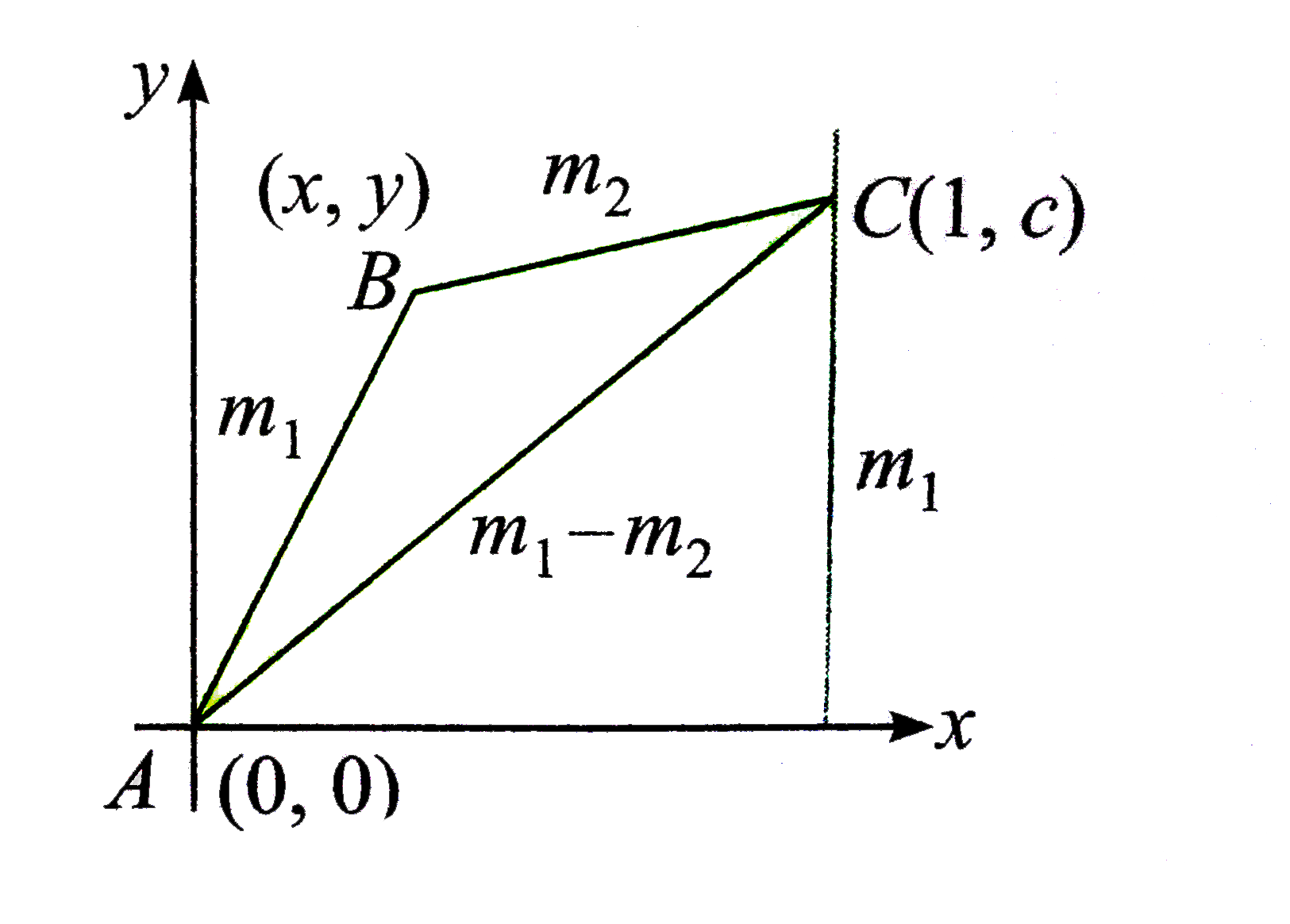
Let the coordinates of C be (1,c). Then,
$m_{2} = \frac{c - y}{1 - x}$
$or m_{2} = \frac{c - m_{1} x}{1 - x}$
$or m_{2} - m_{2} x = c - m_{1} x$
$or (m_{1} - m_{2}) x = c - m_{2}$
$or c = (m_{1} - m_{2}) x + m_{2} (1)$
Now, the area of $Δ A B C$ is
$\frac{1}{2} ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ \begin{matrix} 0 & 0 & 1 x & m_{1} x & 1 1 & c & 1 \end{matrix} ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ = \frac{1}{2} | [c x - m_{1} x] |$
$= \frac{1}{2} | [{(m_{1} - m_{2}) x + m_{2}} x - m_{1} x] |$
$= \frac{1}{2} ∣ ∣ [(m_{1} - m_{2}) x^{2} + m_{2} x - m_{1} x] ∣ ∣$
$= \frac{1}{2} (m_{1} - m_{2}) (x - x^{2}) [∵ x > x^{2} in (0, 1)]$
$Hence, f (x) = \frac{1}{2} (x - x^{2})$
${f (x)}_{max} = \frac{1}{8} when x = \frac{1}{2}$

Updated on:21/07/2023

If the line $y = m x$ meets the lines $x + 2 y - 1 = 0$ and $2 x - y + 3 = 0$ at the same point, then m is equal to
A1
B $- 1$
C2
D $- 2$
The slope of the line $a^{2} X - a Y + 1 = 0$ , where a is constant, is
A $- a^{2}$
B $- a$
Ca
DNone of these
The slopes of the tangents to the curve $y = (x + 1) (x - 3)$ at the points where it cuts the x - axis, are $m_{1} and m_{2}$ , then the value of $m_{1} + m_{2}$ is equal to
A8
B $- 2$
C2
D0