θ1andθ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x,y), then the equation of one of the angle bisector of these lines is
x−x1cos(θ1+θ22)=y−y1sin(θ1+θ22)
x−x1-sin(θ1−θ22)=y−y1cos(θ1−θ22)
x−x1sin(θ1+θ22)=y−y1cos(θ1+θ22)
x−x1-sin(θ1+θ22)=y−y1cos(θ1+θ22)
The correct Answer is:A, D
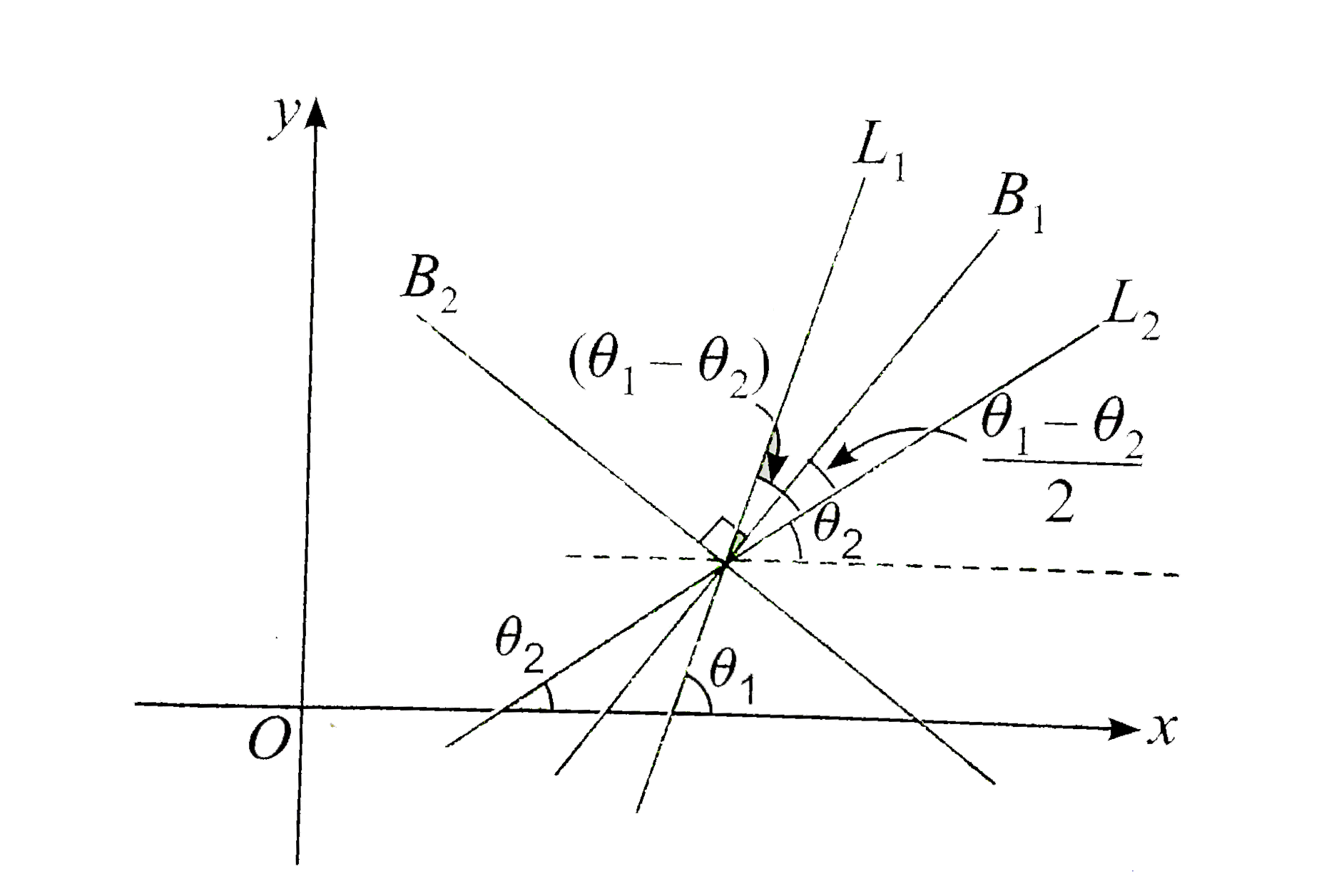
From the figure, angle bisectors B1 and B2 have inclinations of
θ1+θ22 and (π2+θ1+θ22) with the x-axis.
Therefore, equations of angle bisectors are
x−x1cos(θ1+θ22)=y−y1sin(θ1+θ22)
and x−x1cos(π2+θ1+θ22)=y−y1sin(π2+θ1+θ22)
or x−x1-sin(θ1+θ22)=y−y1cos(θ1+θ22)
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Comprehension)
27 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Matrix)
8 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Single)
82 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
θ1 and θ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1) , then the equation of one of the angle bisector of these lines is (a) x−x1cos(θ1−θ22)=y−y1sin(θ1−θ22) (b)x−x1−sin(θ1−θ22)=y−y1cos(θ1−θ22) (c)x−x1sin(θ1−θ22)=y−y1cos(θ1−θ22) (d)x−x1−sin(θ1−θ22)=y−y1cos(θ1−θ22)
View SolutionThe equations of bisectors of two lines L1&L2 are 2x−16y−5=0 and 64x+8y+35=0. lf the line L1 passes through (−11,4), the equation of acute angle bisector of L1 & L2 is:
View Solution
Knowledge Check
The area of triangle formed by the lines l1andl2 and the x-axis is:
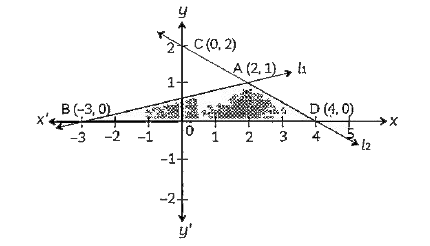 A7 sq. unitsB92 sq.unitsC72 sq.unitsD4 sq. units
A7 sq. unitsB92 sq.unitsC72 sq.unitsD4 sq. unitsA line L passeds through the points (1,1)and(2,0) and another line L' passes through (12,0) and perpendicular to L. Then the area of the triangle formed by the lines L, L' and Y-axis is
A158B254C258D2516Let L1andL2 be the foollowing straight lines.
L1:x−11=y−1=z−13andL2:x−1−3=y−1=z−11
Suppose the striight line L:x−αl=y−mm=z−γ−2 lies in the plane containing L1andL2 and passes throug the point of intersection of L1andL2 if the L bisects the acute angle between the lines L1andL2, then which of the following statements is /are TRUE ?Aα−γ=3Bl+m=2Cα−γ=1Dl+m=0
The bisector of two lines L and L are given by 3x2−8xy−3y2+10x+20y−25=0. If the line L1 passes through origin, find the equation of line L2.
View SolutionIf lines l1,l2 and l3 pass through a point P, then they are called…………. Lines.
View SolutionIn the xy plane, the line L1 passes through the point (1,1) and the line L2 passes through the point (−1,1). If the difference of the slopes of the lines is 2. Then the locus of the point of intersection of the lines L1andL2 is
View SolutionLet L be the line passing through the point P (1, 2) such that its intercepted segment between the coordinate axes is bisected at P. If L1 is the line perpendicular to L and passing through the point (−2,1), then the point of intersection of L and L1 is :
View SolutionLet L be the line passing through the point P (1,2) such that its intercepted segment between the co-ordinate axes is bisected at P. If L1 is the line perpendicular to L and psssing through the point (-2,1), then the point of intersection of L and L1 is
View Solution
