If the straight lines 2x+3y−1=0,x+2y−1=0,andax+by−1=0form a triangle with the origin as orthocentre, then (a,b)is given by

A

(6,4)

B

(−3,3)

C

(−8,8)

D

(0,7)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

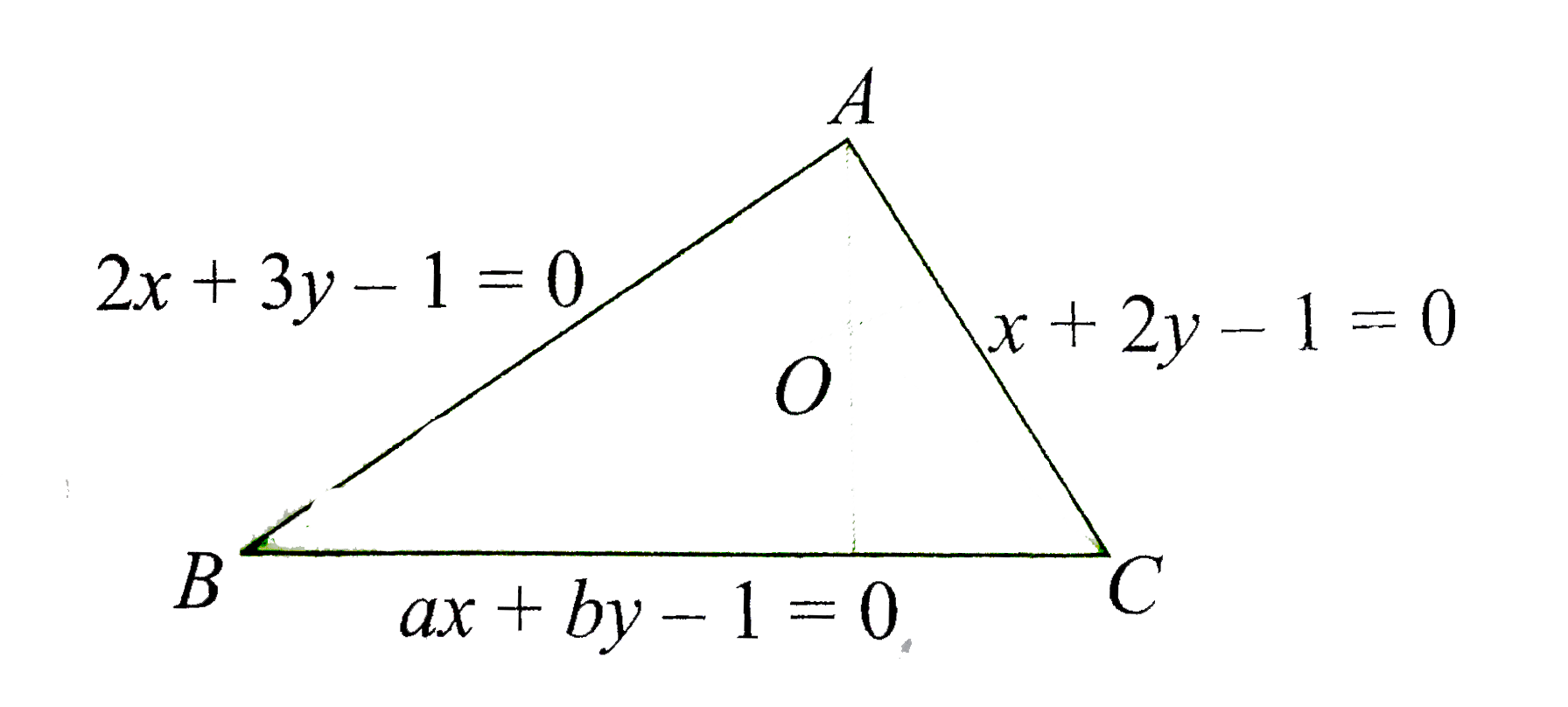
ocr_image
The equation of AO is 2x+3y−1+λ(x+2y−1)=0, where λ=−1, since the line passes through the origin. So, x+y=0. Since AO is perpendicular to BC, we have (−1)(−ab)=−1
∴a=−b
Similarly,
(2x+3y−1)+μ(ax−ay−1)=0
will be the equation of BO for μ=−1. Now, BO is perpendicular to AC. Hence,
{−(2−a)3+a}(−12)=−1
∴a=−8,b=8

Updated on:21/07/2023
|
Class 12MATHSSTRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The straight lines x+y=0, 3x+y-4=0 and x+3y-4=0 form a triangle which is :

    Aisoscles
    Bequilateral
    Cright angled
    DNone of the above

  • The straight lines x+y−4=0,3x+y−4=0 and x+3y−4=0 form a triangle, which is

    Aisoscles
    Bequilateral
    Cright angled
    DNone of the above

  • The straight lines x+y−4=0,3x+y−4=0,x+3y−4=0 form a triangle which is

    Aisosceles
    Bright angled
    Cequilateral
    DNone