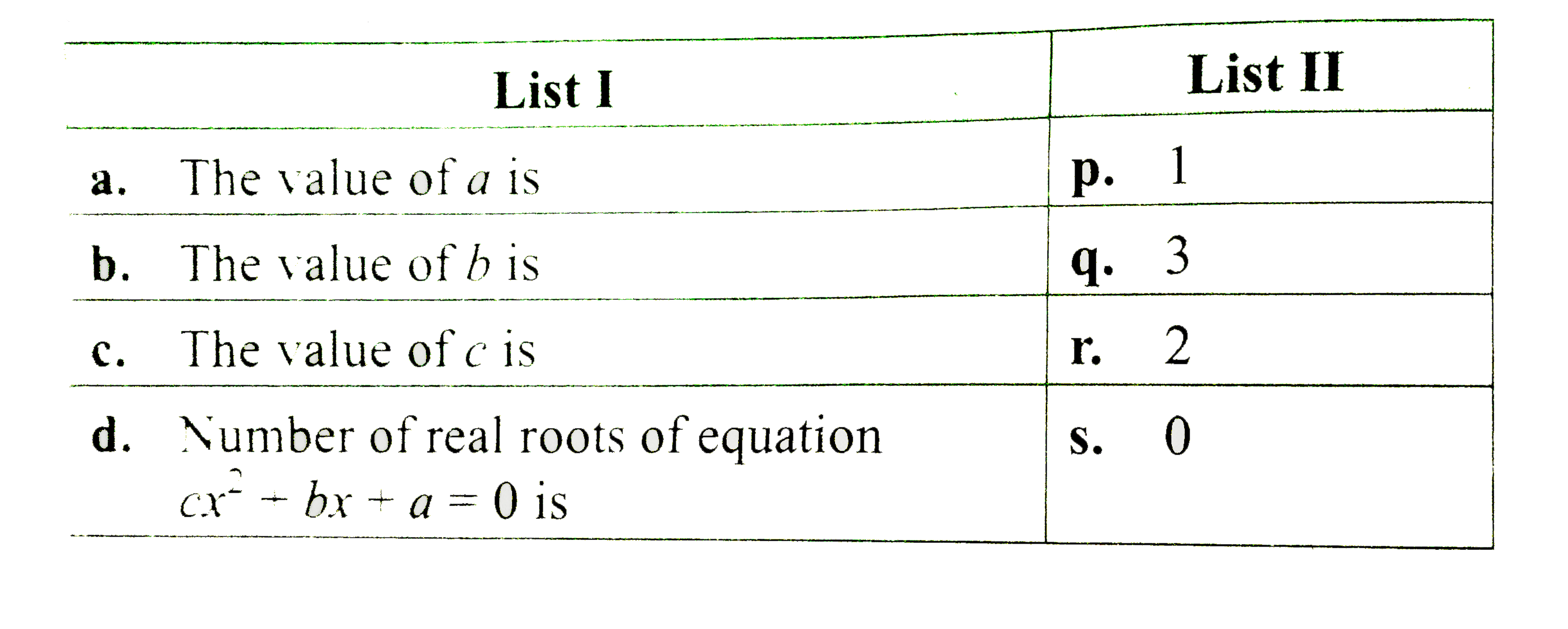
Consider limx→∞(x3+x2+x+sinxx2+2cosx−asinx−bx+c)=4. Now, match the following lists and then choose the correct code.
Video Solution
Text Solution
The correct Answer is:(4)
limx→∞(x3+x2+x+sinxx2+2cosx−asinx−bx+c)=4
⇒ limx→∞⎛⎜⎝x+1+1x+sinxx21+2cosxx2−asinx−bx+c⎞⎟⎠=4
⇒ limx→∞(x+1−asinx−bx+c)=4
⇒ limx→∞((1−b)x−asinx+1+c)=4
⇒ 1−b=0,a=0 and 1+c=4
⇒ b=1,a=0 and c=3
|
Topper's Solved these Questions
- LIMITSBOOK - CENGAGECHAPTER - LIMITSEXERCISE - Exercise (Numerical)
23 Videos
- LIMITSBOOK - CENGAGECHAPTER - LIMITSEXERCISE - JEE Main Previous Year
8 Videos
- LIMITSBOOK - CENGAGECHAPTER - LIMITSEXERCISE - Exercise (Comprehension)
20 Videos
- JEE 2019BOOK - CENGAGECHAPTER - JEE 2019EXERCISE - Chapter 10
9 Videos
- LINEAR COMBINATION OF VECTORS, DEPENDENT AND INDEPENDENT VECTORSBOOK - CENGAGECHAPTER - LINEAR COMBINATION OF VECTORS, DEPENDENT AND INDEPENDENT VECTORSEXERCISE - DPP 1.2
10 Videos
Similar Questions
Let ∫∞0sinxxdx=α Then match the following lists and choose the correct code. :

View SolutionConsider
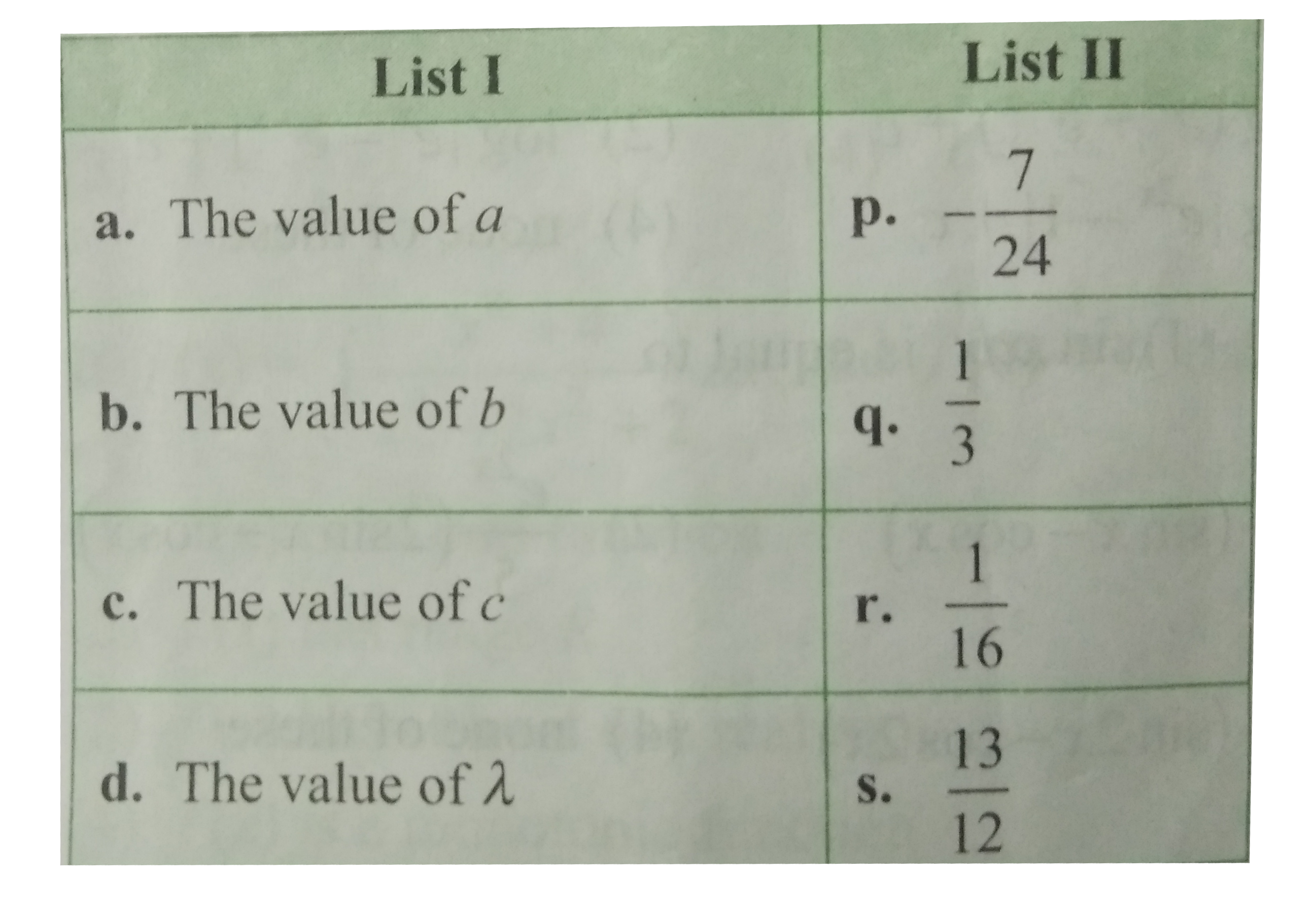
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View Solutionlimx→∞x+3x2+4
View Solutionlimx→∞x+3x2+4
View Solutionlimx→∞x+3x2+4
View Solutionlimx−>∞x2+bx+bx2+bx+c=?
View Solutionlimx−>−∞3x3+2x2−7x+94x3+9x−2
View Solutionlimx→∞[x2+x+3x2−x+2]x=
View Solutionlimx→∞(4+3x2)=
View Solutionlimx→∞2x+13x−2
View Solution
