An open rail road car of mass M is moving with initial velocity v0 along a straight horizontla and friction less track.It suddenly starts raining at time t=0. The rain drops fall vertically with velocity with velocity u and add a mass of m kg/sec of water . The velocity of car after t second is
M+mMV0t
MV0tM+v
Mv0M+mt
M(M+m)v0
The correct Answer is:C
To solve the problem, we will apply the principle of conservation of momentum. Here's the step-by-step solution:
Step 1: Understand the Initial Conditions
- The mass of the railroad car is M.
- The initial velocity of the car is v0.
- The rain adds mass at a rate of m kg/s.
Step 2: Determine the Initial Momentum
The initial momentum Pinitial of the car can be calculated as:
Pinitial=M⋅v0
Step 3: Calculate the Mass Added After Time t
After t seconds, the mass of water added to the car is:
mass of water=m⋅t
Thus, the total mass of the car after t seconds becomes:
Total mass=M+m⋅t
Step 4: Define the Final Velocity
Let the final velocity of the car after t seconds be V. The final momentum Pfinal can be expressed as:
Pfinal=(M+m⋅t)⋅V
Step 5: Apply Conservation of Momentum
According to the conservation of momentum:
Pinitial=Pfinal
Substituting the expressions we derived:
M⋅v0=(M+m⋅t)⋅V
Step 6: Solve for Final Velocity V
Rearranging the equation to solve for V:
V=M⋅v0M+m⋅t
Final Answer
The velocity of the car after t seconds is:
V=M⋅v0M+m⋅t
---
- The mass of the railroad car is M.
- The initial velocity of the car is v0.
- The rain adds mass at a rate of m kg/s.
The initial momentum Pinitial of the car can be calculated as:
Pinitial=M⋅v0
After t seconds, the mass of water added to the car is:
mass of water=m⋅t
Thus, the total mass of the car after t seconds becomes:
Total mass=M+m⋅t
Let the final velocity of the car after t seconds be V. The final momentum Pfinal can be expressed as:
Pfinal=(M+m⋅t)⋅V
According to the conservation of momentum:
Pinitial=Pfinal
Substituting the expressions we derived:
M⋅v0=(M+m⋅t)⋅V
Rearranging the equation to solve for V:
V=M⋅v0M+m⋅t
The velocity of the car after t seconds is:
V=M⋅v0M+m⋅t
Topper's Solved these Questions
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:SECTION (II) : (SUBJECTIVE )
Explore 33 Videos
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:SECTION(II) : MCQ ( SINGLE CORRECT)
Explore 47 Videos
- KINEMATICSBook:FIITJEEChapter:KINEMATICSExercise:NUMERICAL BASED QUESTIONS
Explore 3 Videos
- HEAT AND TEMPERATUREBook:FIITJEEChapter:HEAT AND TEMPERATUREExercise:NUMERICAL BASES QUESTIONS
Explore 1 Video
- LAWS OF MOTIONBook:FIITJEEChapter:LAWS OF MOTIONExercise:COMPREHENSION-III
Explore 2 Videos
Similar Questions
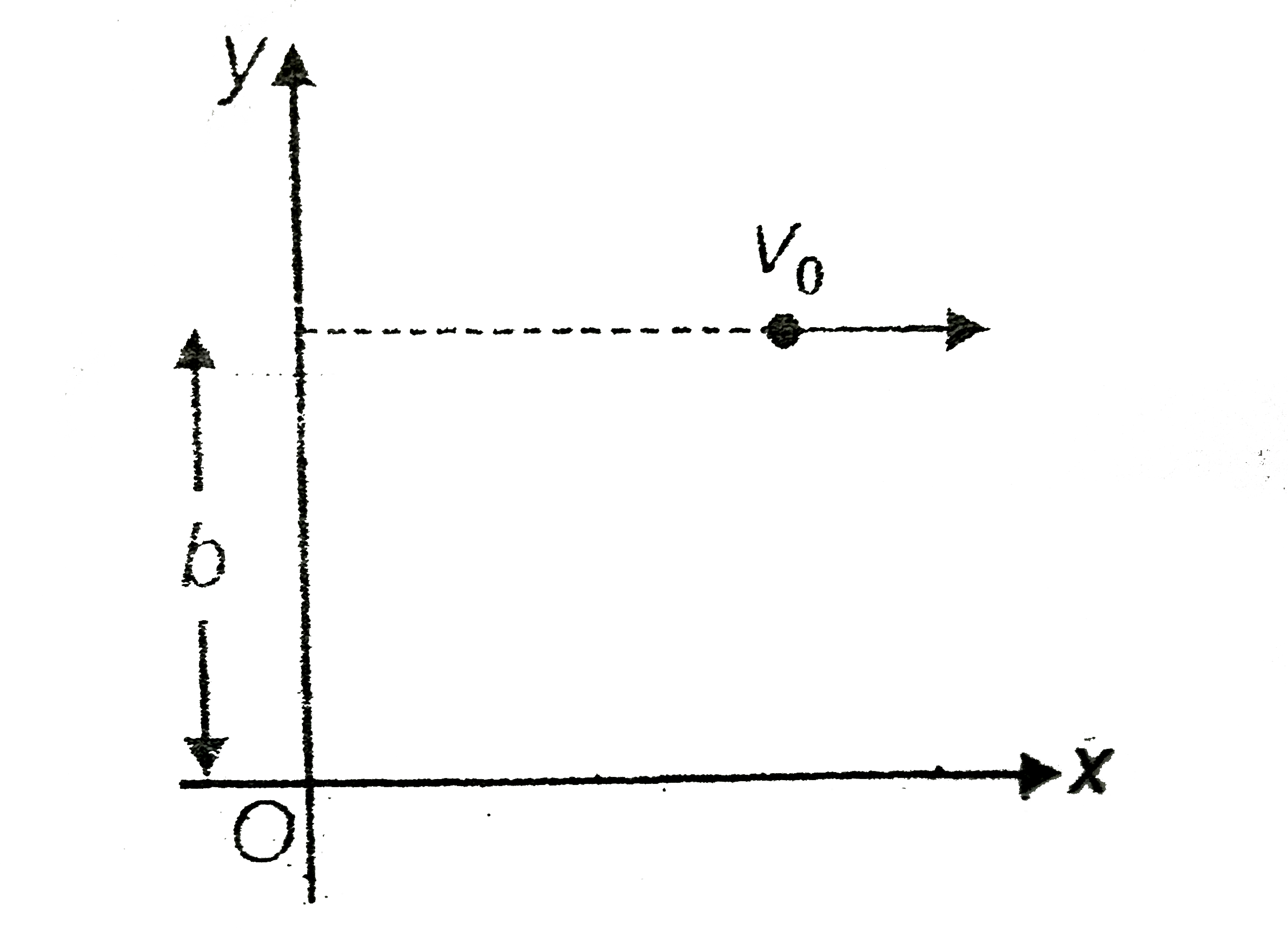
A particle of mass m is moving with constant velocity v0 along the line y=b. At time t=0 it was at the point (0,b). At time t=bv0, the
View SolutionAn open water tight railway wagon of mass 5×103kg moves at an initial velocity 1.2m/s without friction on a railway track. Rain drops fall vertically downwards into the wagon. The velocity of the wagon after it has collected103kg of water will be :-
View Solution
Knowledge Check
An open topped rail road car of mass M has an initial velocity v0 along a straight horizontal frictionless track. It suddenly starts raising at timet = 0. The rain drops fall vertically with velocity u and add a mass m kg/sec of water. The velocity of car after t second will be (assuming that it is not completely filled with water)
Av0+muMBMv0M+mtCMv0+utM+utDv0+mutM+utA particle moves with an initial velocity v0 and retardation αv, where v is the velocity at any time t.
AThe particle will cover a total distance v0αBthe particle will come to rest after time 1αCthe particle will continue to move for a along timeDThe velocity of particle will become v0e after time 1αAn open water tight railway wagon of mass 5×10(3)kg coats at an initial velocity 1.2m/s without friction on a railway track. Rain drops fall vertically downwards into the wagon. The velocity of the wagon after it has collected103kg of water will be :-
A0.5m/sB2m/sC1m/sD1.5m/s
A particle move with a velocity v=αt3 along a straight line.The average velocity in time interval t=0 to t=T is
View SolutionA railroad flatcar of mass M can roll without friction along a straight horizontal track. Initially, a man of mass 'm' is standing on the car, which is at rest. What is the velocity of the car if man runs to the left so that his speed relative to the car is vrel.
View Solutiona. A rail road car of mass M is moving without friction on a straight horizontal track with a velocity ct. A man of mass m lands on it normally from a helicopter. What will be the new velocity of the car? ltbgt b. If now the man begins to run on it with speed um with respect to car in a direction opposite to motion of the car, what will be the new velocity of the car?
View SolutionAn isolated rail car of mass M is moving along a straight, frictionless track at an initial speed v0. The car is passing under a bridge when a crate filled with N bowling balls, each of mass m, is dropped from the bridge into the bed of the rail car. The crate splits open and the bowling balls bounce around inside the rail car, but none of them fall out. Is the momentum of the rail car + bowling balls system conserved in this collision ?
View SolutionA particle moves with an initial velocity V0 and retardation αv , where α is a constant and v is the velocity at any time t.
Velocity of particle at time is :
View Solution