Find charge on each spherical shell after joining the inner most shell outer most shell by a conducting wire. Also find charges on each surface.
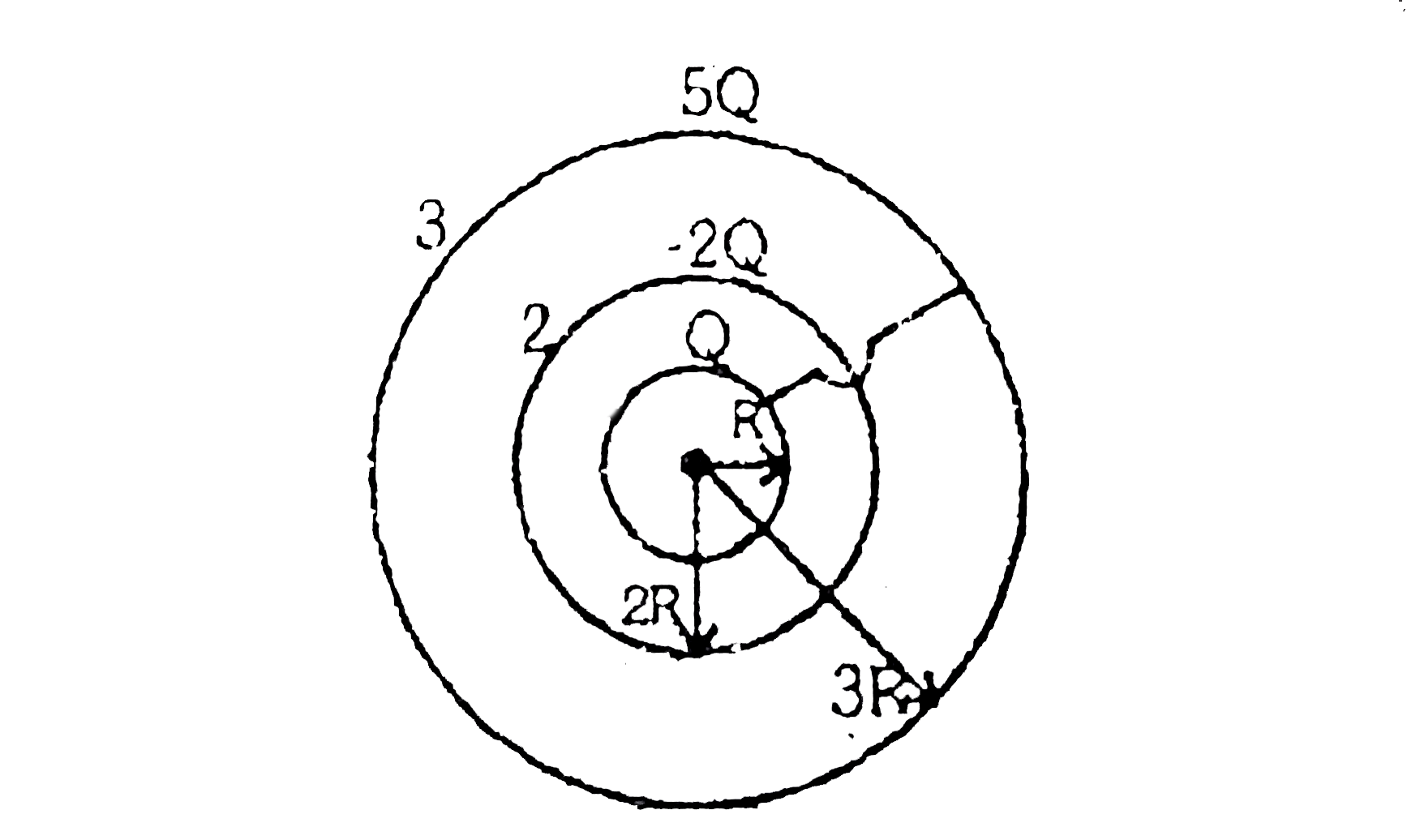
Let the charge on the innermost sphere be x.
Finally potential of shell 1= potential of shell 3
∴KxR+K(−2Q)2R+K(6Q−x)3R=Kx3R+K(−2Q)3R+K(6Q−x)3R
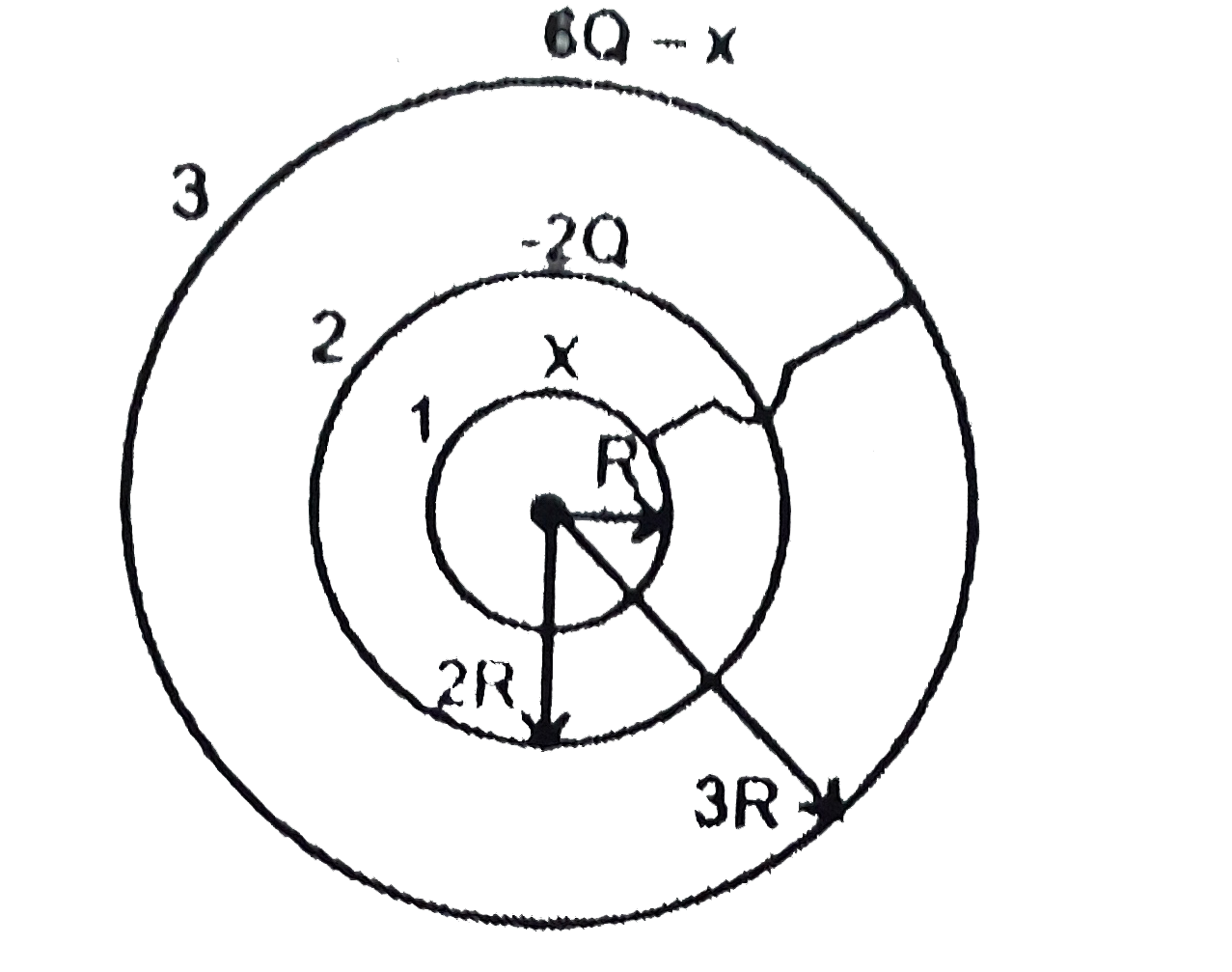
3x−3Q+6Q−x=4Q,2x=Q,x=Q2
∴ Charge on innermost shell =Q2 & Charge on outermost shell =5Q2
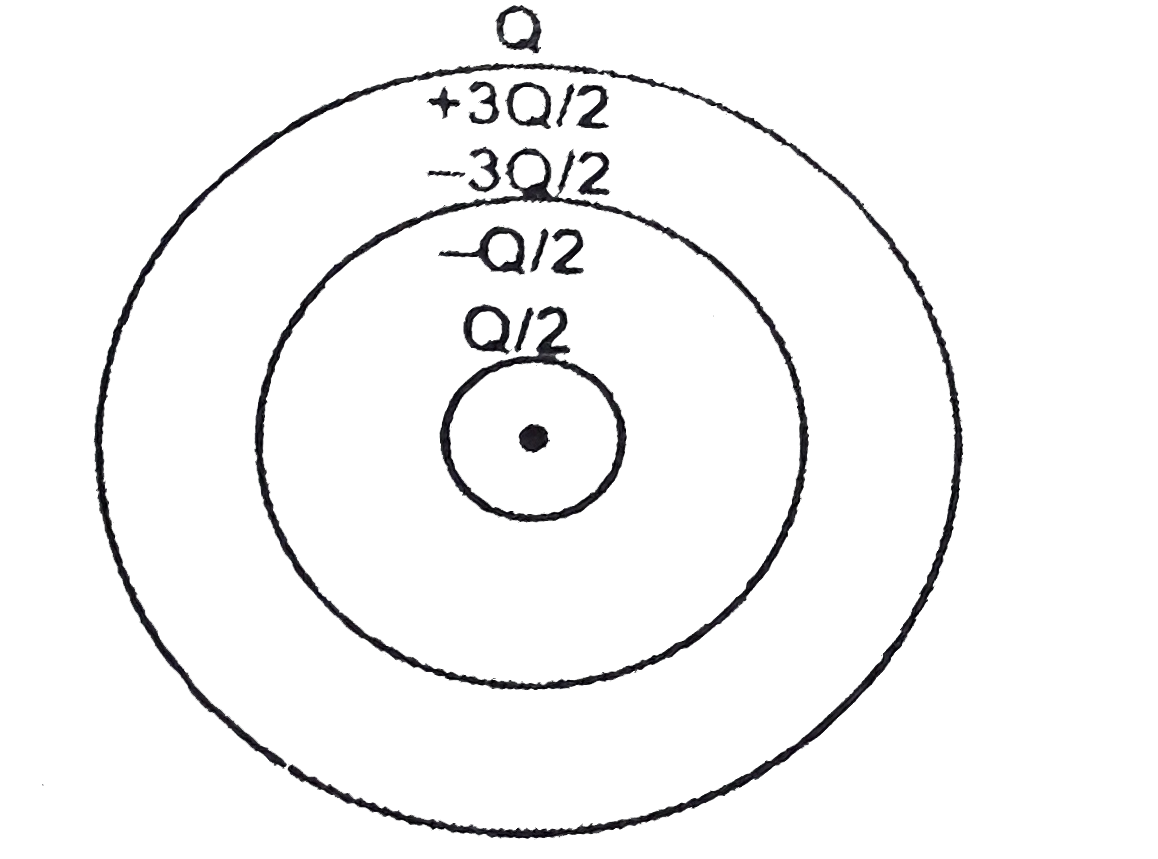
Charge on middle shell =−2Q
∴ final charge distribution is as shown in figure.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Problems
22 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (A)
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
There are three concentric metallic spherical shells of radius R, 2R and 3R. Charge Q is given to the innermost spherical shell. If the innermost spherical shell is connected with a metal wire to the outermost spherical shell without touching the middle shell, which is connected to the earth, then, find charges appearing on all surfaces.
View SolutionThe outer diameter of a spherical shell is 12 cm and its inner diameter is 8 cm. Find the volume of metal contained in the shell. Also, find its outer surface area.
View Solution
Knowledge Check
Three concentric conducting spherical shells carry charges as : +4Q on the inner shell, −2Q on the middle shell and −5Q on the outer shell. The charge on the inner surface of the outer shell is
A0B4QC−QD−2QThree concentric conducting spherical shells carry charges +4Q on the inner shell −2Q on the middle shell and +6Q on the outer shell. The charge on the inner surface of the outer shell is
A0B4QC−QD−2QShown in the figure a spherical shell with an inner radius 'a' and an outer radius 'b' is made of conducting metarial. A charge +Q is placed at the centre of the spherical shell and a total charge -q s placed on the shell. Charge -q is districuted on the surfaces as :
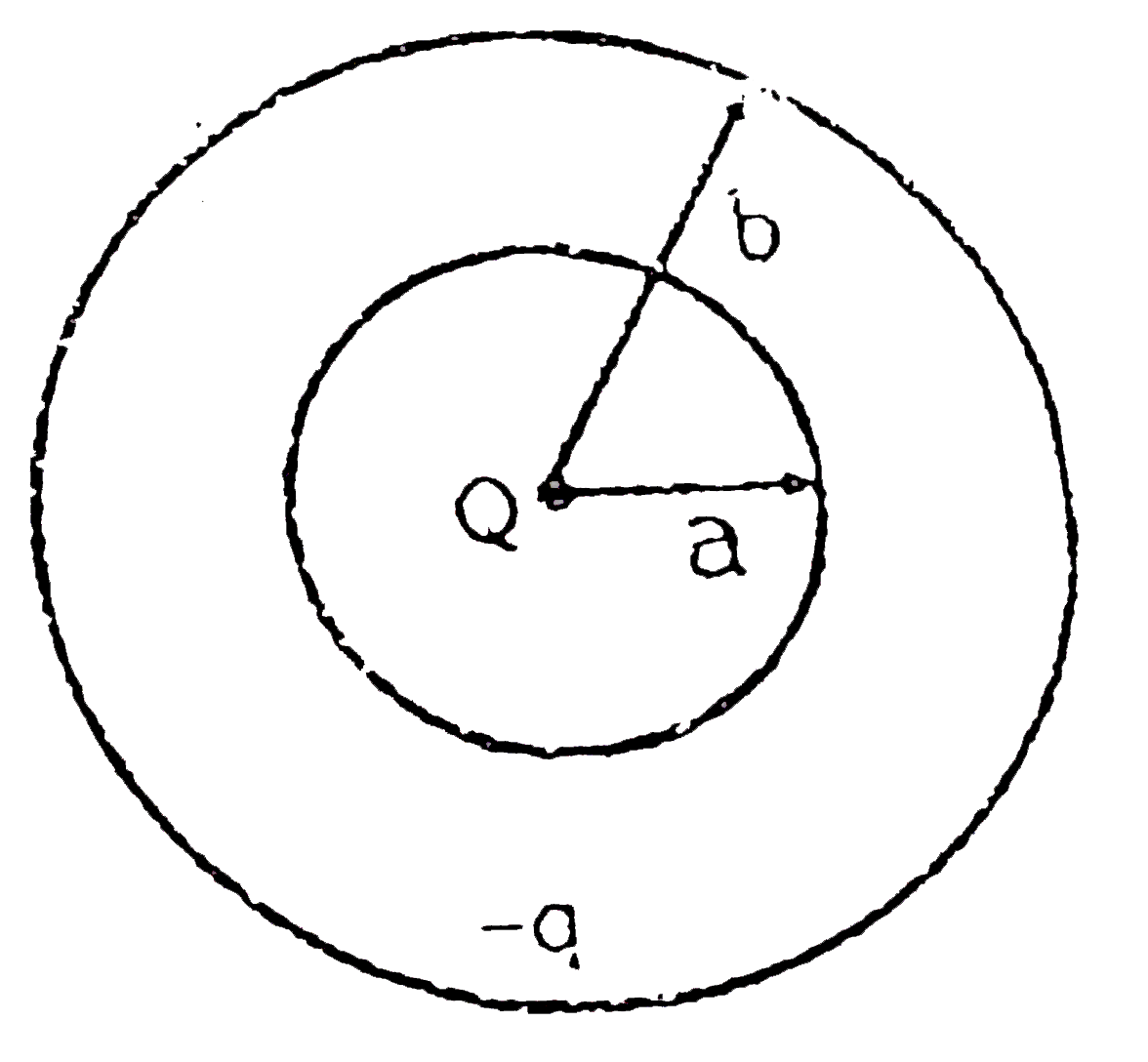 A−Q on the inner surface, −q on outer surfaceB−Q on the inner surface, −q+Q on the outer surfaceC+Q on the inner surface, −q−Q on the outer surfaceDThe charge −q is spread uniformly between the inner and outer surface
A−Q on the inner surface, −q on outer surfaceB−Q on the inner surface, −q+Q on the outer surfaceC+Q on the inner surface, −q−Q on the outer surfaceDThe charge −q is spread uniformly between the inner and outer surface
If a thin spherical shell of radius r and charge q is kept inside a thick spherical shell of inner radius 2r, outer radius 3r, and total charge 2q. (a) Find the charge distribution on each surface. (b) Find the self-energy of each charge distribution. (c) Find the total electrostatic energy stored in the system.
View SolutionThere are two concentric metal shells of radii r1 and r2(>r1). If initially, the outer shell has a charge q and the inner shell is having zero charge and then inner shell is grounded. Find :
(i) Charge on the inner surface of outer shell.
(ii) Final charges on each sphere.
(iii) Charge flown through wire in the ground.
View SolutionFigure shows three concentric conducting spherical shells with inner and outer shells earthed and the middle shell is given a charge q. Find the charge distribution of sphere

View SolutionThere are three conducting and concentricspherical shells of radii R,2R and 3R. The charge on inner and outer most shells are q and 3q while middle shell is earthed. Find the ratio of number of field lines emitted by outermost shell and inner shell.
View SolutionTwo concentric spherical conducting shells of radii r and R (r<R) carry charges q and Q respectively . The two shells are now connected by a conducting wire. The final charge on the inner shell is
View Solution