An uncharged conductor of inner radius R1 and outer radius R2 constains a point charge q at the centre as shown in figure
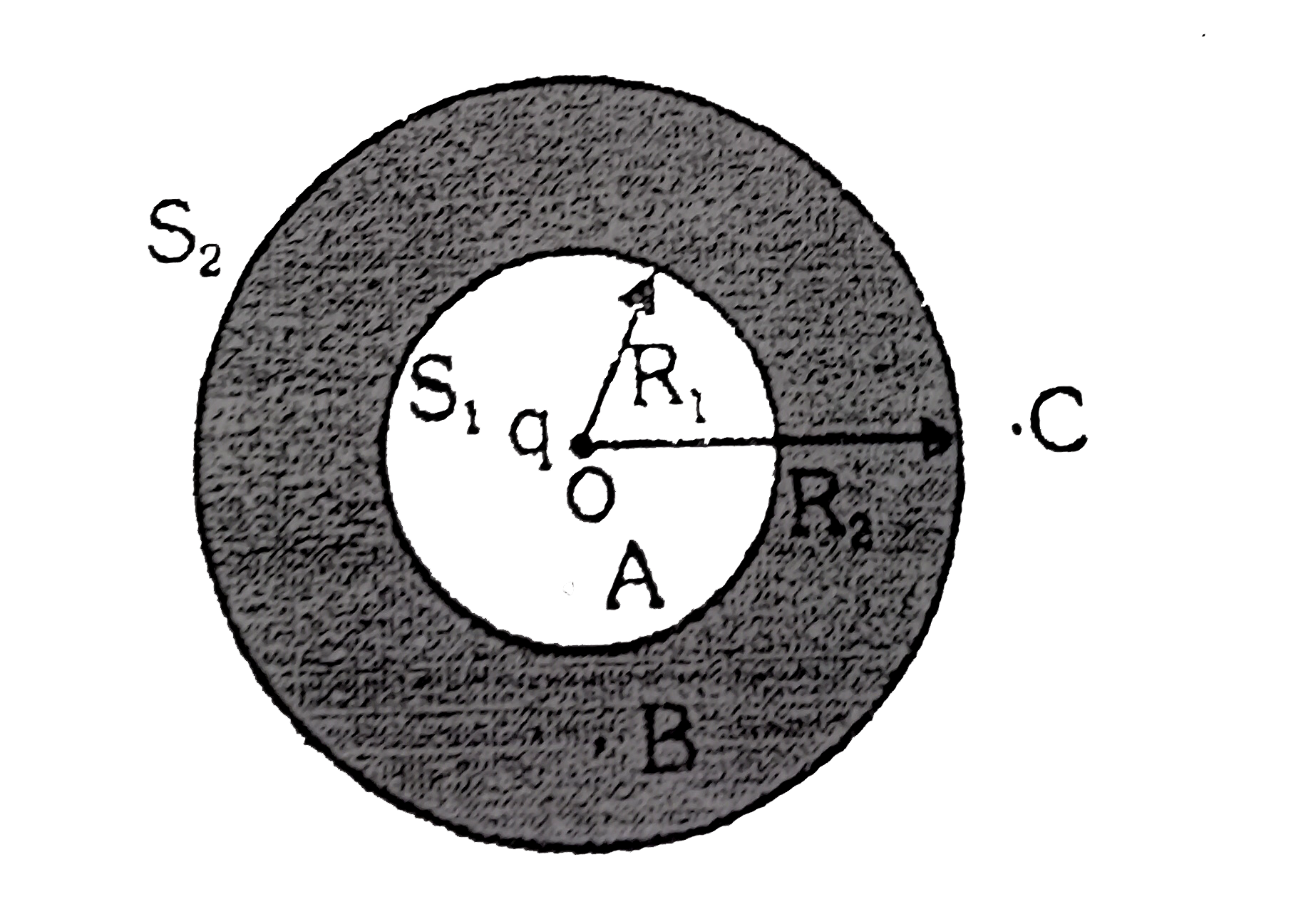
(i) Find →E and V at points A, B and C
(ii) If a pint charge Q is kept out side the sphere at a distance 'r'(>>R2) from centre then find out resultant force on charge Q and charge q.
At point A :

VA=KqOA+KqR2+K(−q)R1,→EA=KqOA3−−→OA
Note : Electric field at 'A' due to -q od S1 and +q of S2 is zero individually because they are uniformly distributed
at point B : VB=KqOB+K(−q)OB+KqR2=KqR2,EB=0
At point C : VC=KqOC,→EC=KqOC3−−→OC
(ii) Force on point charge Q:
(Note : Here, force on 'Q' will be only due to 'q' of S2 (see result (iii))
→F0=KqQr2ˆr (r = distance of 'Q' from centre 'O')
Force on point charge q :
→F0=0 (using result (iii) & charge on S1 uniform)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Problems
22 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (A)
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
An unchanged conductor of inner radius R1 and outer radius R2 contains a point charge q placed at point P (not at the centre) as shown in figure? Find out the following:

(i) VC (ii) VA (iii) VB (iv) EA (v) EB
(vi) force on charge Q if it is placed at B
View SolutionInside a conducting hollow sphere of inner radius R1 and outer radius R2, a point charge q is placed at a distance x from the center as shown in (Fig. 3.70.) Find.
 .
.
(i) electric potential at C
(ii) electric field and potential at a distance r from the center outside the shell.
View Solution
Knowledge Check
A hollow conducting sphere of inner radius r and outer radius 2R is given charge Q as shown in figure, then the
 Apotential at A and B is same.Bpotential at O and B is same.Cpotential at O and C is same.Dpotential at A,B,C and O is same.
Apotential at A and B is same.Bpotential at O and B is same.Cpotential at O and C is same.Dpotential at A,B,C and O is same.A conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
AVrBR2Vr3CRVr2DrVR2A conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
ARVr2BVRCrVR2DR2Vr2
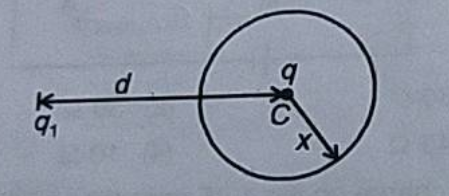
Inside a neutral hollow conducting sphere of radius X and centre C, a point charge q is placed as shown in the figure. Another point charge q1, is placed outside the sphere at distance d from centre. The net- electrostatic force on charge q placed at the centre is K=14πε0
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the centre of the shell. What is the surface charge density on the inner and outer surfaces of the shell ?
View SolutionA metal spherical shell has an inner radius R1 and outer radius R2. A charge Q is placed at the center of the spherical cavity. What will be surface charge density on (i) the inner surface, and (ii) the outer surface ?
View SolutionA point charge Q is placed outside a hollow spherical conductor of radius R , at a distance r(r>R) from its centre C . The field at C due to the inducted charges on the conductor is
View SolutionIf charge q induced on outer surface of sphere of radius R, then intensity at point P at distance S from centre is
View Solution