Figure shows three large metallic plates with chrges and respectively. Determine the final charges on all the surfaces.
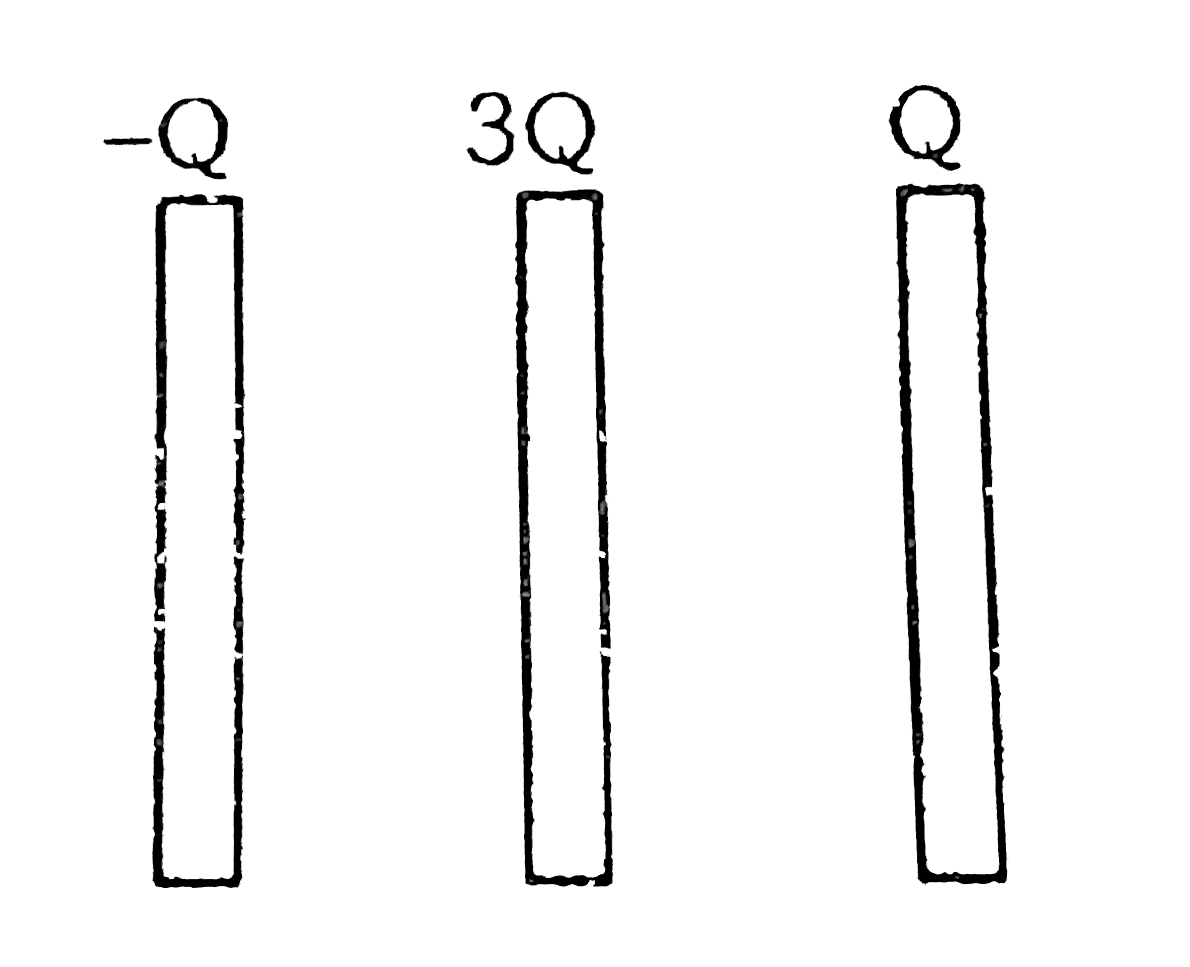
We assume that charge on surface 2 is x. Following conservation of charge, we see that surfaces 1 has charge . The electric field inside the metal plate is zero. So, field at P is zero.
Resultant field at P
or

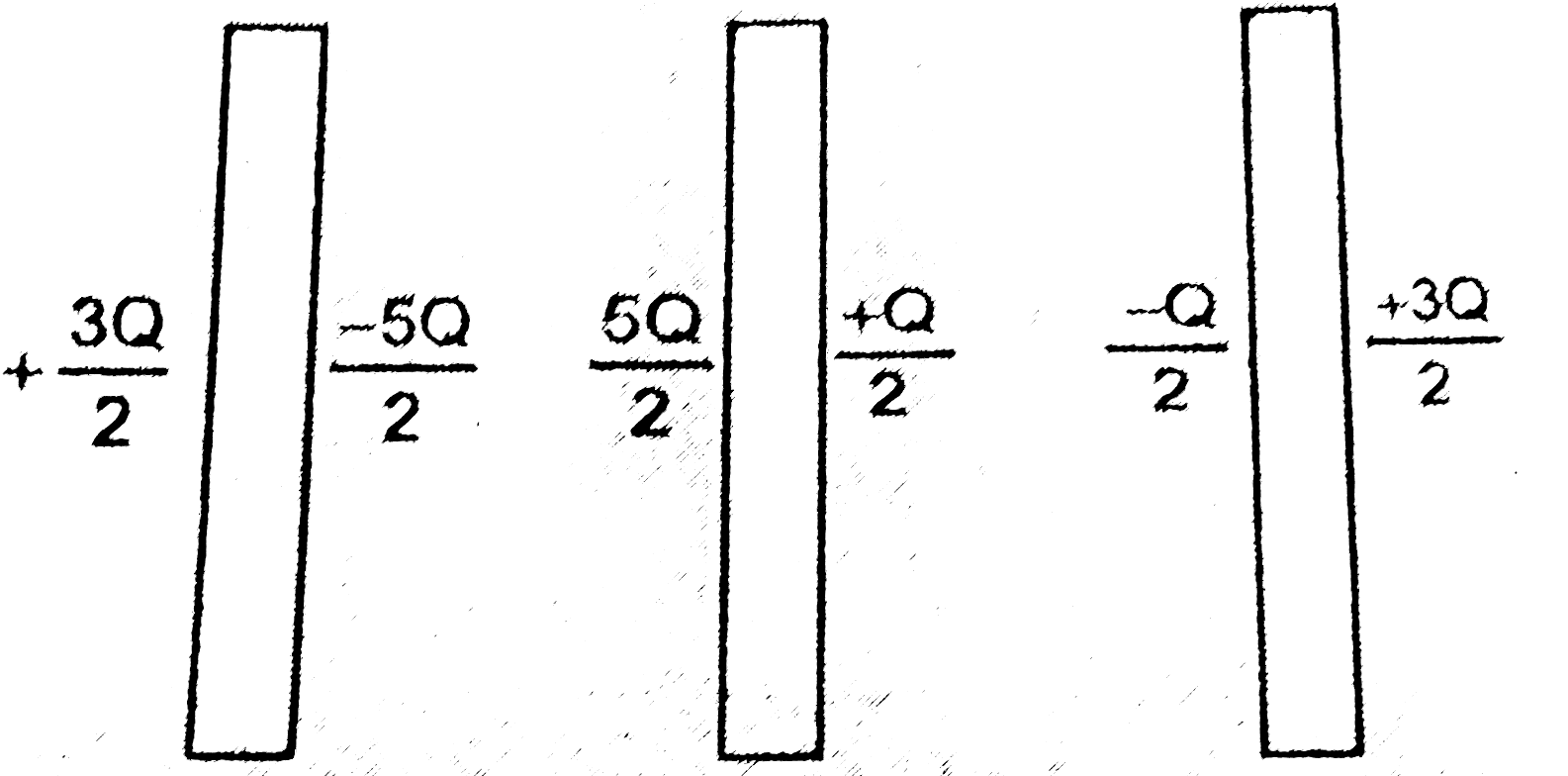
Note : We see that charges on the facing surfaces of the plates are of equal magnitude and opposite sign. This can be in general proved by Gauss theorem also. Remember this, it is important result. Thus the final charge distribution on all the surface is as shown in figure :
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Problems
22 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (A)
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
Figure shows three metallic plates with charges Q, 4Q, and -Q, respectively. Determine the final charges on all the surfaces.
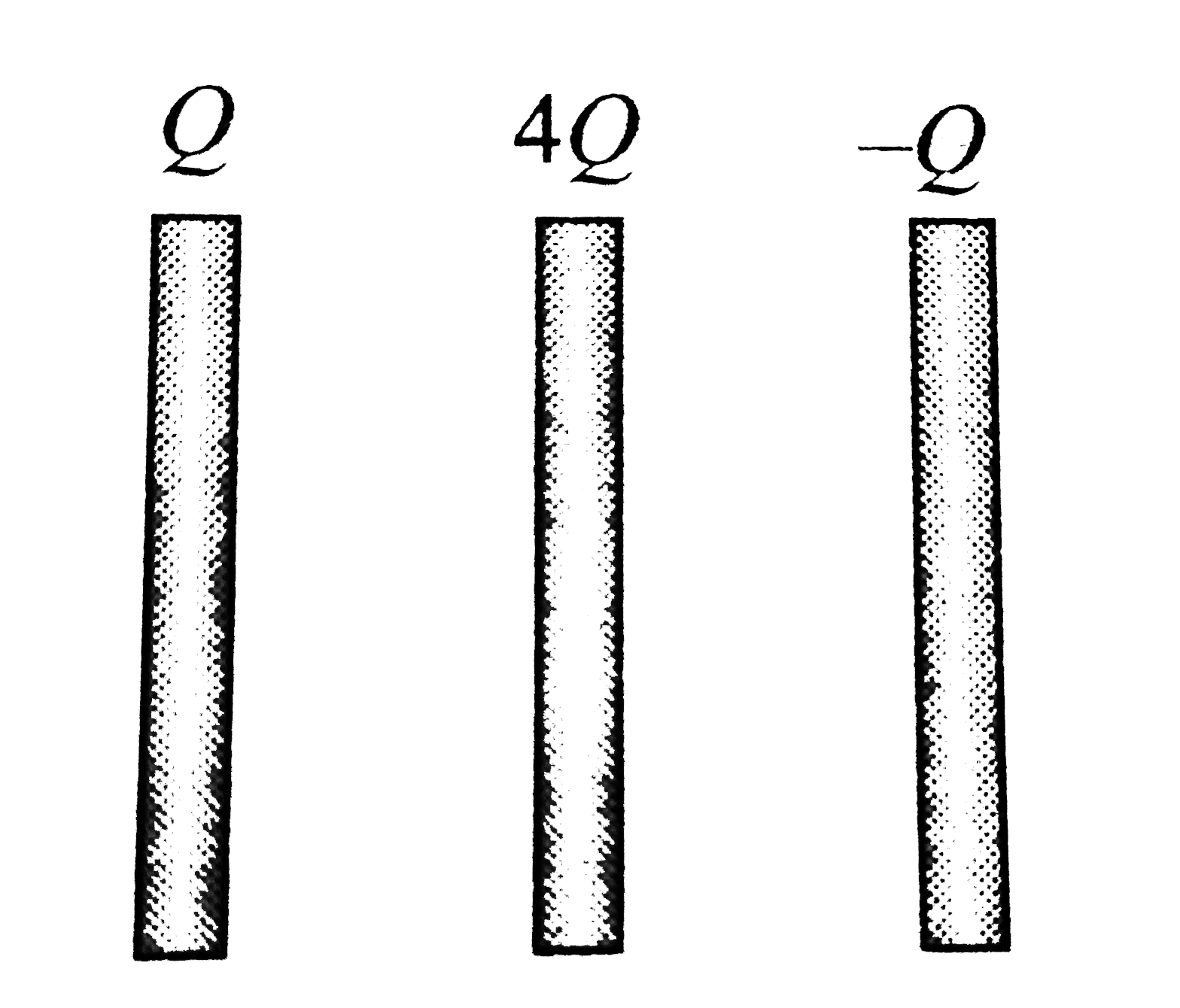
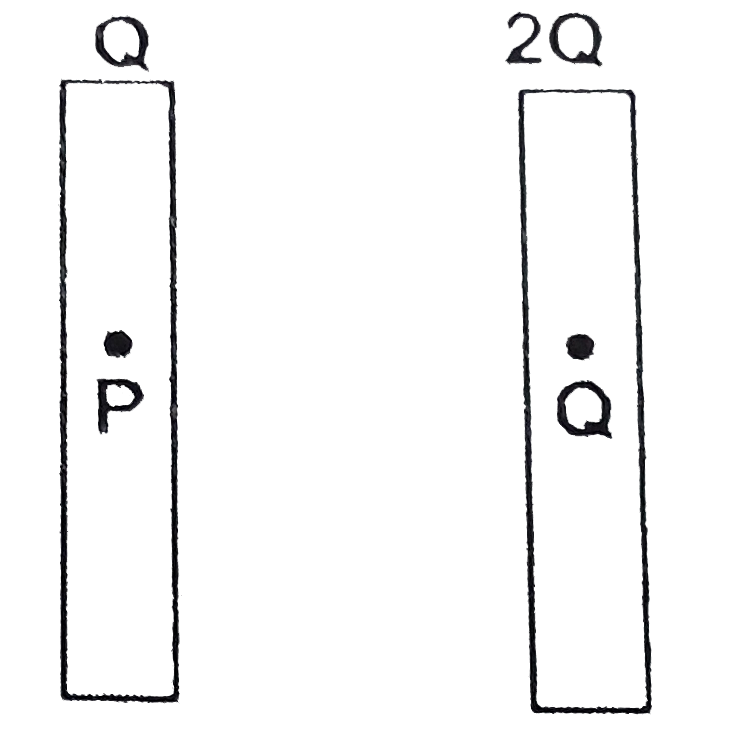
View SolutionTwo large parallel conducting sheets (placed at finite distance) are given charges Q and 2Q respectively. Find out charges appearing on all the surfaces.
View Solution
Knowledge Check
Three large identical conducting plates of area A are closely placed parallel to each other as shown (the area A is perpendicular to plane of diagram). The net charge on left, middle and right plates are QL,QM and QR respectively. Three infinitely large parallel surface SL,SM and SR are drawn passing through middle of each plate such that surface are perpendicular to plane of diagram as shown. Then pick up the correct option(s).
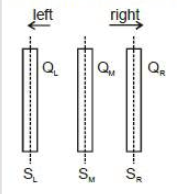 AThe net charge on left side of surface SL is equal to net charge on right side of surface SRBThe net charge on left side of surface SL is equal to net charge on right side of surface SMCThe net charge on left side of surface SL is equal to net charge on right side of surface SLDThe net charge on right side of surface SL is equal to net charge on left side of surface SR
AThe net charge on left side of surface SL is equal to net charge on right side of surface SRBThe net charge on left side of surface SL is equal to net charge on right side of surface SMCThe net charge on left side of surface SL is equal to net charge on right side of surface SLDThe net charge on right side of surface SL is equal to net charge on left side of surface SRThree concentric metallic spherical shells of radii R, 2R, 3R, are given charges Q1, Q2, Q3, respectively. It is found that the surface charge denisties on the outer surfaces of the shells are equal. Then, the ratio of the charges given to the shells, Q1:Q2:Q3, is
A(a) 1:2:3B(b) 1:3:5C(c) 1:4:9D(d) 1:8:18
Figure shows three concentric thin conducting spherical shells A, B and C of radii R, 2R and 3R. The shell B is earthed A and C are given charges q and 2q respectively. Find the charges appearing on all the surface of A,B and C.

[inner surface (A)0,(B)−q,(C)43q
Outer surface (A)q,(B)−43q,(C)23q]
View SolutionThere are n large parallel plate conductors carrying charge Q1,Q2,………..Qn respectively.

i. Find the charge induced at surface A.
ii. Find the charge induced at surface B.
iii. If the left conductor is earthed, find the magnitude of charge flowing from plate to earth.
iv. If any conductor is earthed, find the magnitude of charge flowing from plate to earth.
View SolutionThree identical metal square plates of surface area A of each side is arranged as shown in the figure.Plates 1 2 and 3 are given charges +20, -Q and -Q respectively. Find out the correct options A) Charge on the two faces of the middle plate are -2Q and Q B) Charge on the two faces of the middle plate are -Q and 0 C) Charge on the two faces of the left most plate are zero and 2Q D) Charge on the two faces of the right most plate are -Q and zero
View SolutionFigure shows three concentric thin spherical shells A, B and C of radii a, b and c respectively. The shells A and C are given charges q and -q respectively and the shell B is earthed. Find the charges appearing on the surfaces of B and C.

View SolutionTwo large conducting plates of a parallel plate capacitor are given charges Q and 3Q respectively. If the electric field in the region between the plates is E0 , then the force of interaction between the plates is

View SolutionThree identical metallic plates are kept parallel to one another at a separation of a and b The outer plates are connected by a thin conducting wire and a charge Q is placed on the central plate Find final charges on all the surfaces of the three plates.
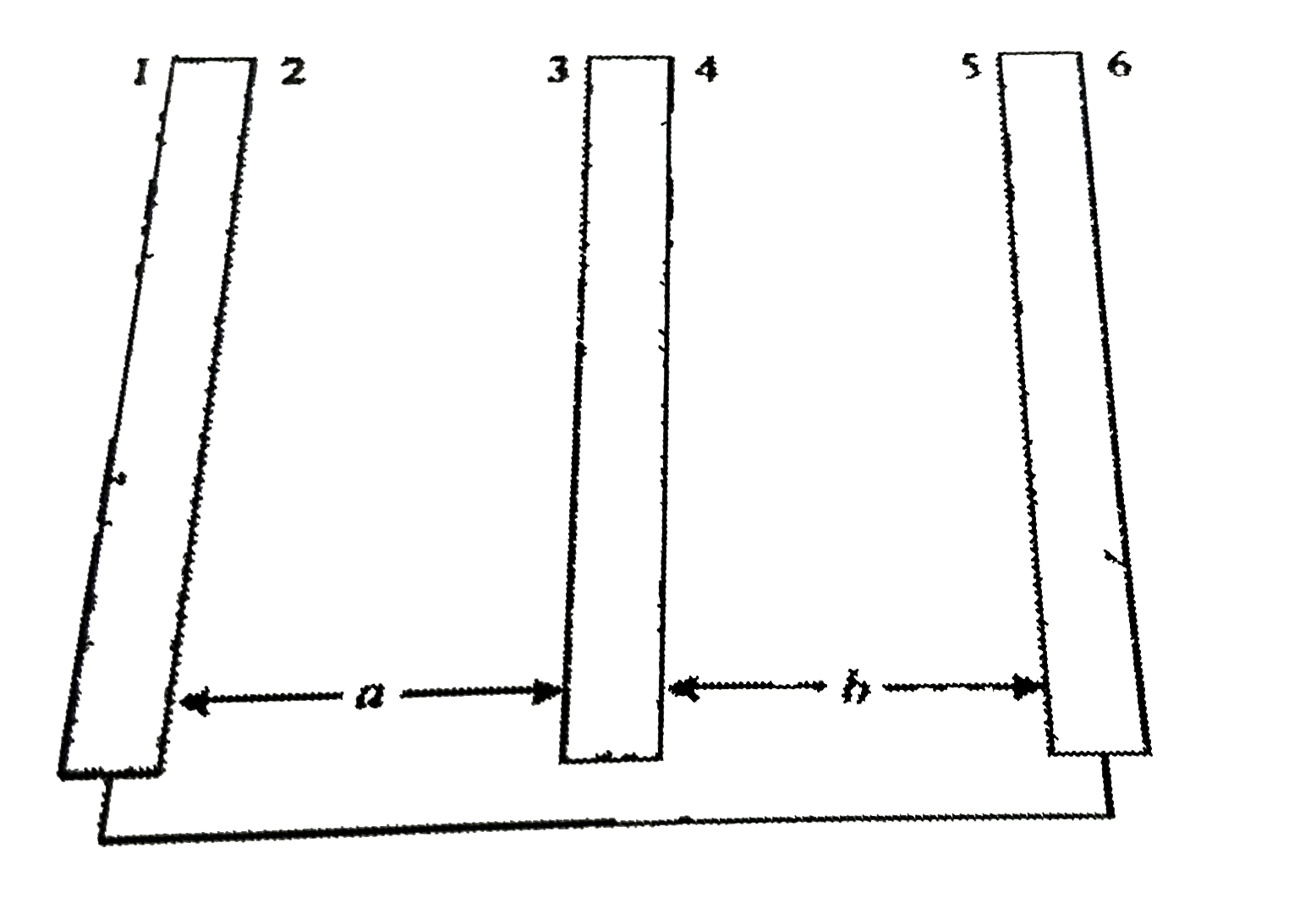
[faces(1)Q2,(2)−Qba+b,(3)Qba+b,(4)Qaa+b,(5)−Qaa+b,(6)Q3]
View Solution
