A ring of radius a contains a charge q distributed. uniformly ober its length. Find the electric field at a point. on the axis of the ring at a distance x from the centre.
Consider an element of small angle dϕ at an angle ϕ as shown.
Soordinates of element : (Rcosϕ,Rsinϕ,0)
Coordinates of point : (0, 0, x)
Now electric field due to element :
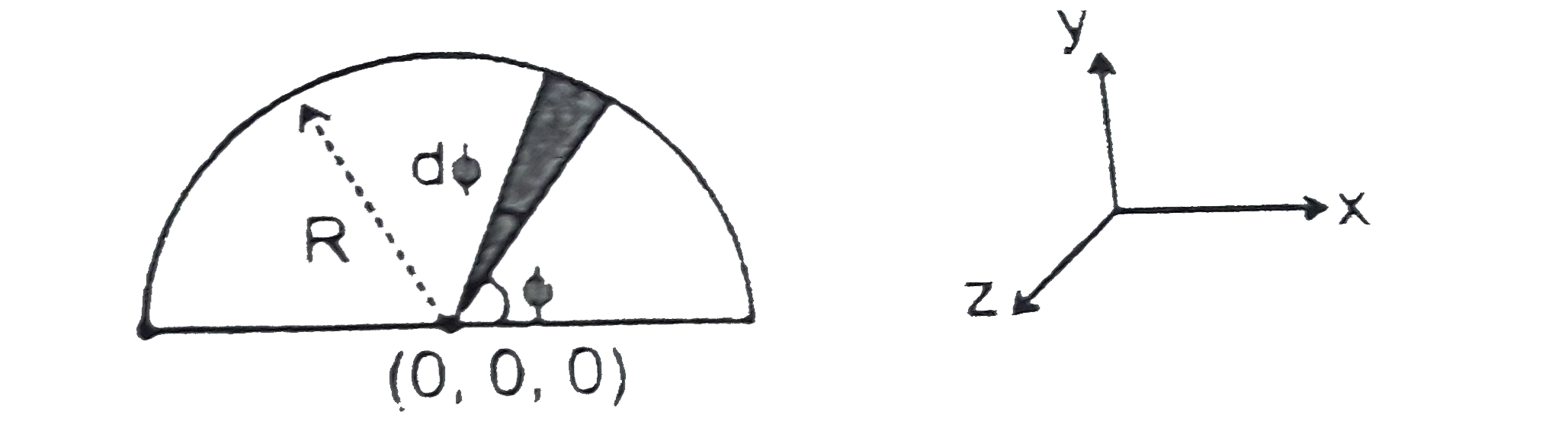
−→dE=K(λRdϕ).[−Rcosϕˆi−Rsinϕˆj+xˆk](R2cos2ϕ+R2sin2ϕ+x2)3/2⇒Ex=ΣdEx=−π∫0KλR2cosϕdϕ(R2+x2)3/2=0
Ey=ΣdEy=−π∫0KλR2sinϕdϕ(R2+x2)3/2=2KλR2(R2+x2)3/2=2KQRπ(R2+x2)3/2
Ez=ΣdEz=π∫0KλRxdϕ(R2+x2)3/2=KQx(R2+x2)3/2
Enet=√E2x+E2y+E2z=KQ(R2+x2)3/2√4R2π2+x2
alternate solution :
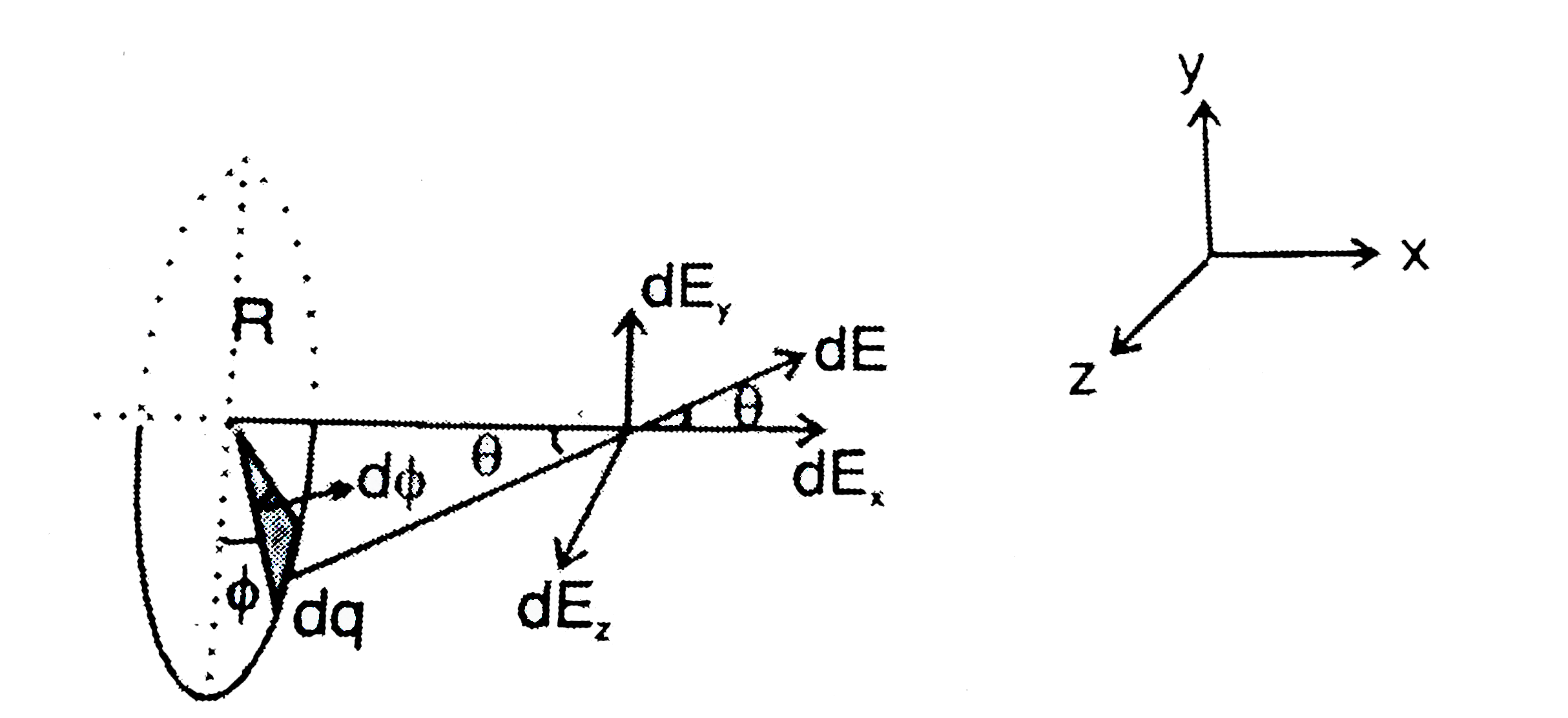
Consider an element of charge dq at an angle ϕ on circumference of half ring. Due to this element electric field at the point on axis, which is at a distance x from the centre of half ring is dE. This electric field can be resolved into three component.

EZ=π/2∫−π/2dEsinθsinϕ=0
EX=π/2∫−π/2dEcosθ=KQx[R2+x2]3/2 ...(1)
EY=π/2∫−π/2dEsinθcosϕ=∫KdqR2+x2sinθ.cosϕ=2KλRsinθR2+x2 ...(2)
∴dq=λRdϕ,sinθ=R√R2+x2 ⇒ Enet=√E2X+E2Y
Topper's Solved these Questions
- ELECTROSTATICSBook:RESONANCEChapter:ELECTROSTATICSExercise:Problems
Explore 22 Videos
- ELECTROSTATICSBook:RESONANCEChapter:ELECTROSTATICSExercise:Exercise-1 Section (A)
Explore 9 Videos
- ELECTROMAGNETIC INDUCTIONBook:RESONANCEChapter:ELECTROMAGNETIC INDUCTIONExercise:A.l.P
Explore 19 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCEChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
Similar Questions
A thin circular ring of radius r is uniformly charged over its length.Consider two points,the centre of ring 0 ,and a point P on the axis of the ring at a distance a from the centre of the ring.If ratio of potential at points O and P is V0Vp=√101, then value of ar is ..
View SolutionA thin circular ring of radius r is uniformly charged over its length.Consider two points the centre of ring 0 and a point P on the axis of the ring at a distance a from the centre of the ring.If ratio of potential at points 0 and P is (V_(0))/(V_(p))=(sqrt(10))/(1) then value of (a)/(r) is
View SolutionMass of 10 kg is distributed uniformly over a ring of radius 1m. Find the gravitational potential at a point lies on the axis of the ring at a distance of 1m from the centre.
View SolutionMass of 20 kg is distributed uniformly over a ring of radius 2m. Find the the grvitational field at a point lies an th axis of the ring at a distance of 2√3 m from the centre.
View SolutionA ring of radius r has a uniformly spread charge + q on quarter of its circumference. The opposite quarter of the ring carries a charge – q uniformly spread over it. Find the electric potential at a point A shown in the figure. Point A is at a distance R(gtgt r) from the centre of the ring.
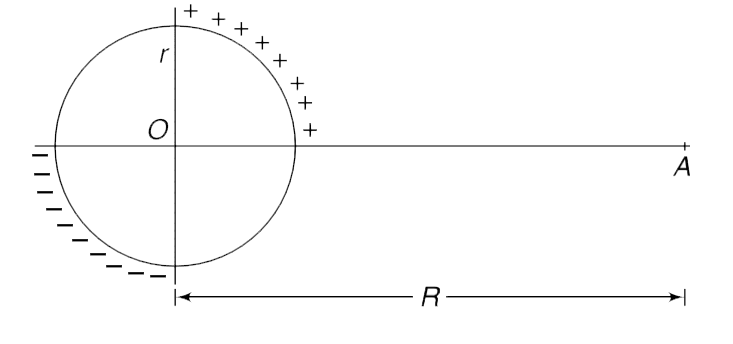
View SolutionConsider a circular ring of radius r, uniformly charged with linear charge density λ. Find the electric potential aty a point on the exis at a distance x from the centre of the ring. Using this expression for the potential, find the electric field at this point.
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionConsider a uniformly charged ring of radius R and total charge Q. What is the magnitude of maximum possible electric field intensity on the axis of ring? What is the distance of point from the centre of ring at which the electric field is maximum?
View SolutionA point charge q is located at the centre fo a thin ring of radius R with uniformly distributed charge −q, find the magnitude of the electric field strength vectro at the point lying on the axis of the ring at a distance x from its centre, if x>>R.
View SolutionA charge is distributed uniformly over a ring of radius ‘a’. Obtain an expression for the electric intensity E at a point on the axis of the ring. Hence show that for points at large distances from the ring, it behaves like a point charge.
View Solution