Two cells of unequal emfs ε1 and ε2, and internal resistances r1 and r2 are joined as shown. VA and VB are the potentials as A and B respectively.
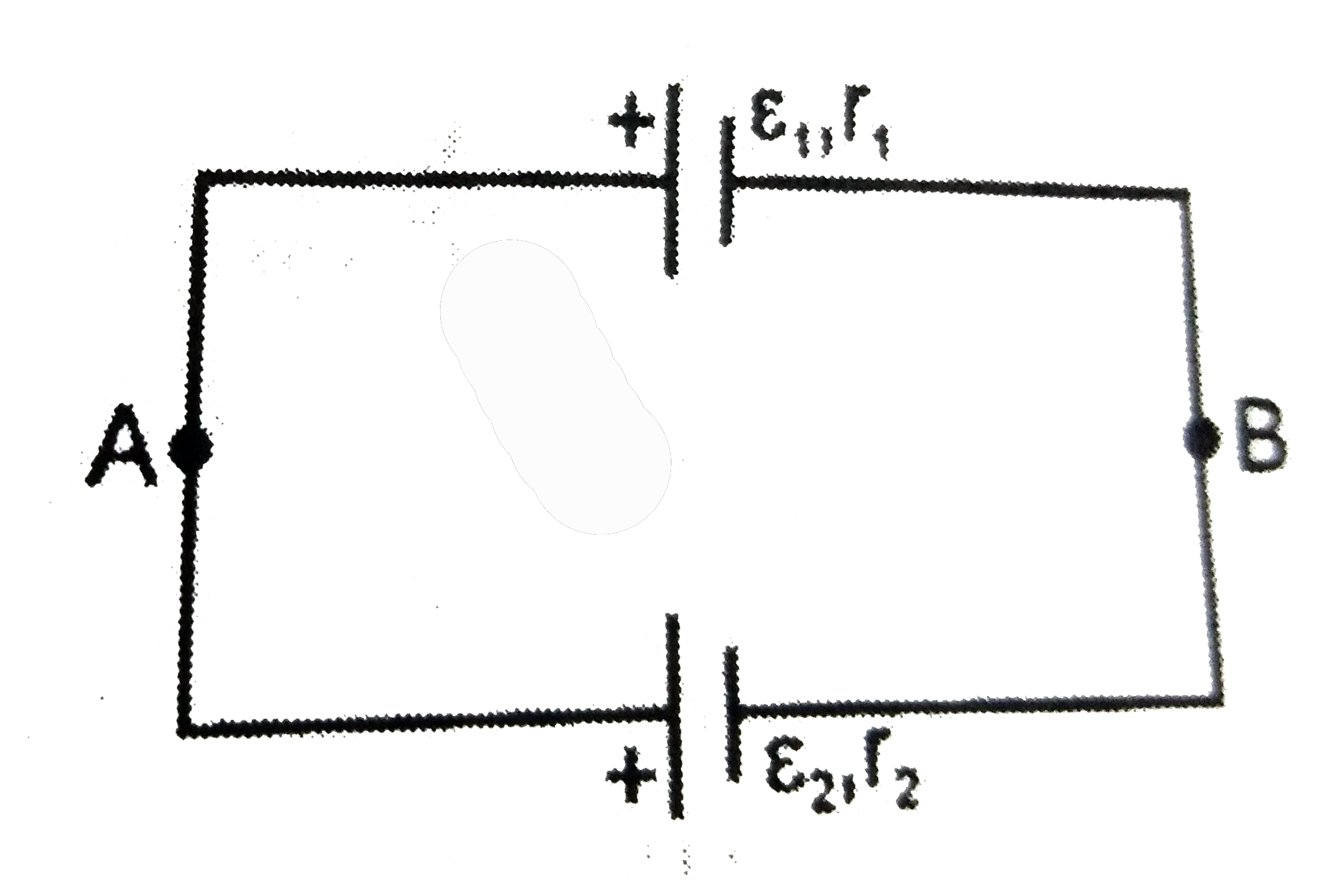
One cell will supply energy to the other
The potential difference across both the cells will be equal
The potential difference across one cell will be greater then its emf
DA−VB=(ε1r2+ε2r1)r1+r2
The correct Answer is:A, B, C, D
Step by step video & text solution for Two cells of unequal emfs epsi_(1)" and "epsi_(2), and internal resistances r_(1)" and "r_(2) are joined as shown. V_(A)" and "V_(B) are the potentials as A and B respectively. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise-2 (Part-4)
12 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - Exrcies-3(PART-1)
22 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise-2 (Part-2)
16 Videos
- COMMUNICATION SYSTEMSBOOK - RESONANCECHAPTER - COMMUNICATION SYSTEMSEXERCISE - Exercise 3
13 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP.No.71
1 Videos
Similar Questions
Two cells of emfs ε1andε2 and internal resistances r1andr2 respectively are connected in parallel . Obtain expressions for the equivalent (i) resistance and , (ii) emf of the combination.
View SolutionTwo cells of emf ε1 and ε2 having internal resistances r1 and r2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the pointsB1 and B2 .

View Solution
Knowledge Check

Two cells of unequal emfs, ɛ1 and ɛ2 and internal resistances r1 and r2 are joined as shown. VA and VB are the potentials at A and B respectively.AOnce cell wil continuously suply energy to the other.BThe potential difference across botht eh cells will be equal.CThe potential difference across one cell will be greater than its emf.DVA−VB=(ɛ1r2+ɛ2r1)(r1+r2)Two cells of unequal emfs ε1 and ε2, and internal resistance r1 and r2 are joined as shown. VA and VB are the potential at A and B respectively.
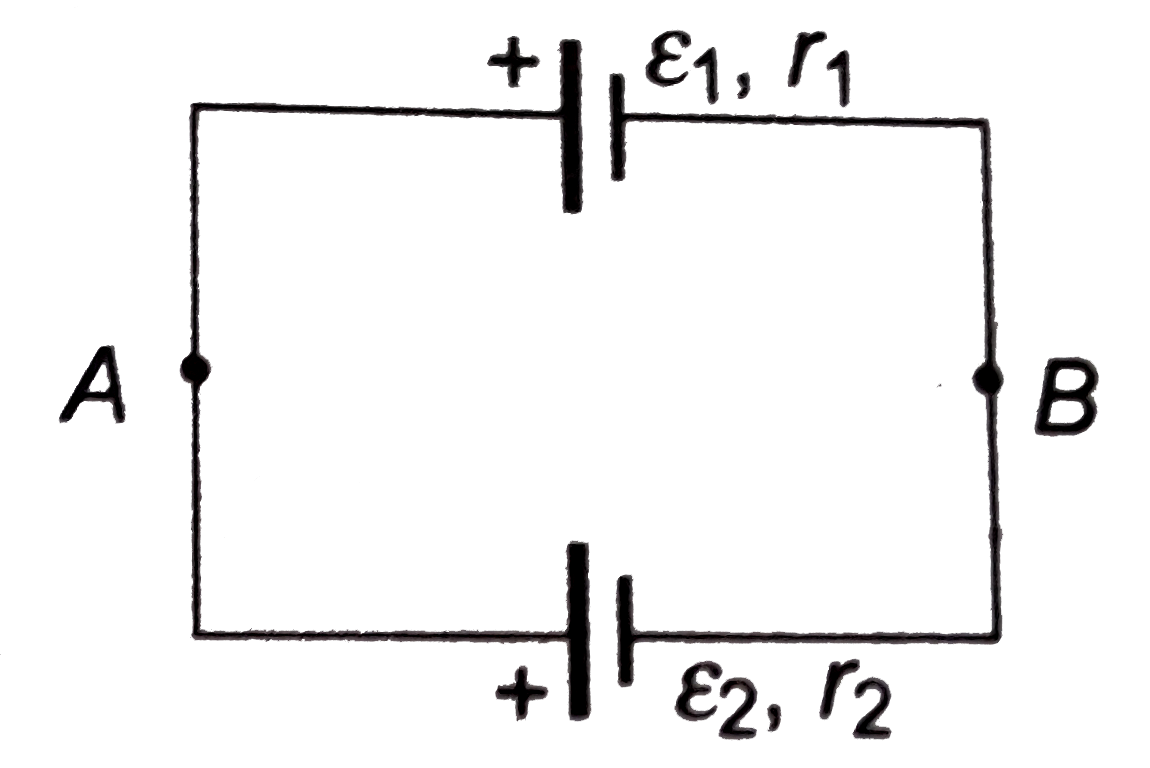
(i) One cell will continuously supply energy to the other
(ii) The potential difference across both the cells will be equal
(iii) The potential difference across one cell with be greater than its emf.
(iv) VA−VB=ε1r1+ε2r2r1+r2.A(i),(ii)B(ii),(iii)C(i),(iv)DallAssertion:- To cells of unequal emf E1 and E2 having internal resistances r1 and r3 are connected as shown in figure. Then the potential difference across any cell cannot be zero.

Reason:- If two cells having nonzero internal resistance and unequal emf are connected across each other as shown, then the current in the circuit cannot be zero.
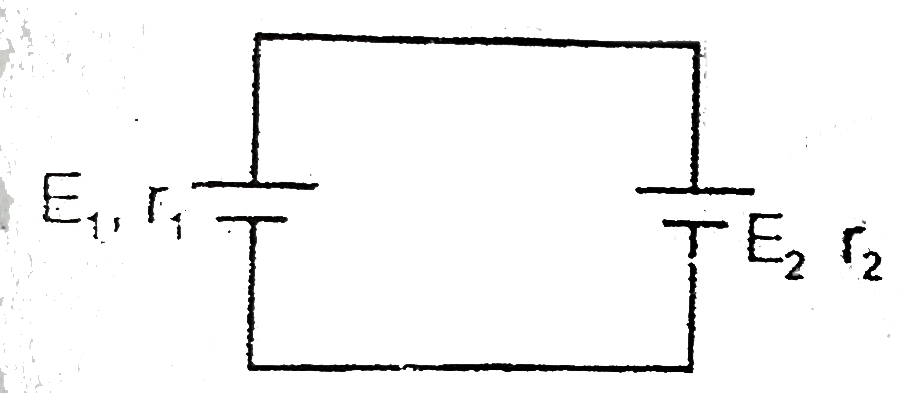 AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are False.
AIf both Assertion & Reason are True & the Reason is a correct explanation of the Assertion.BIf both Assertion & Reason are True but Reason is not a correct explanation of the Assertion.CIf Assertion is True but the Reason is False.DIf both Assertion & Reason are False.
(a) Two cells of emf ε1 and ε2 having internal resistances r1 andr2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the points B1 and B2 . (b) Calculate the current shown by the ammeter in the circuit diagram given below
View Solution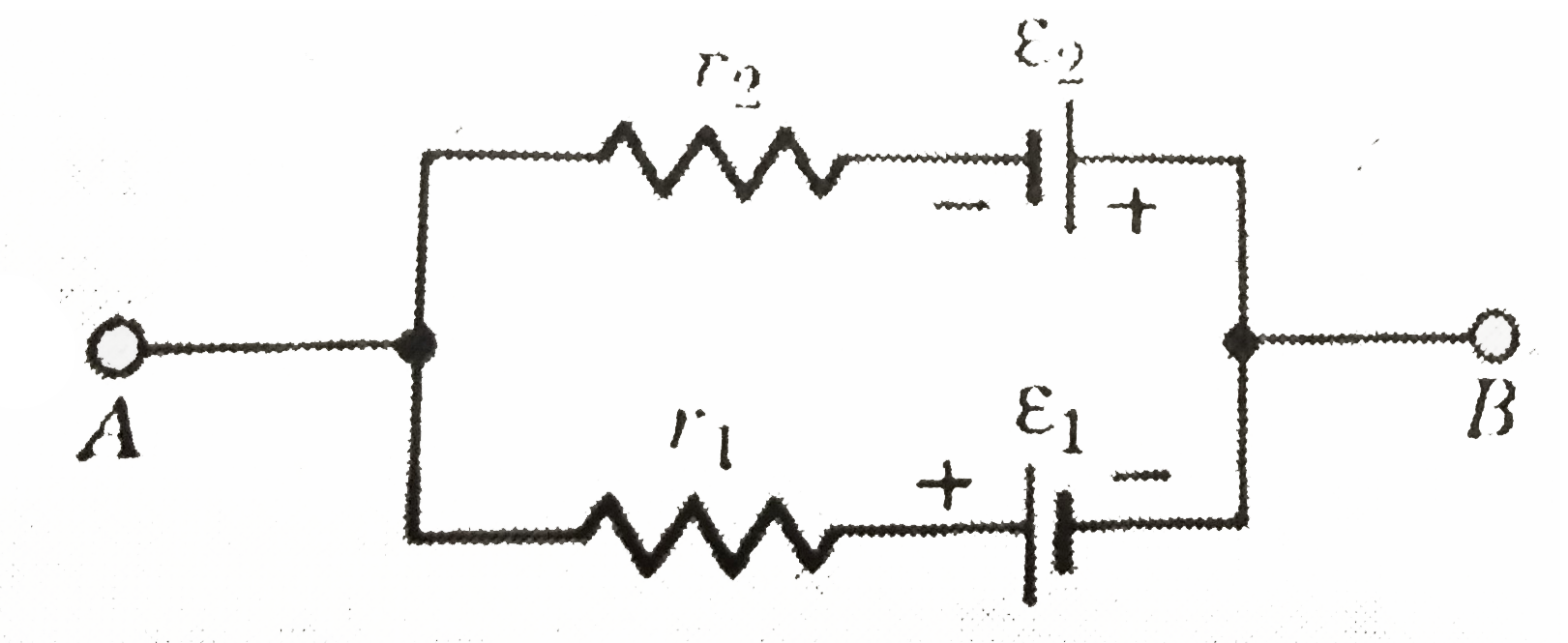
find the emf (ε0) and internal resistance (r0) if a battery which is equivalent to a parallel combination of two batteries of emfs ε1andε2 and internal resistances r1andr2 respectively, with polarities as shown in figure.
View Solution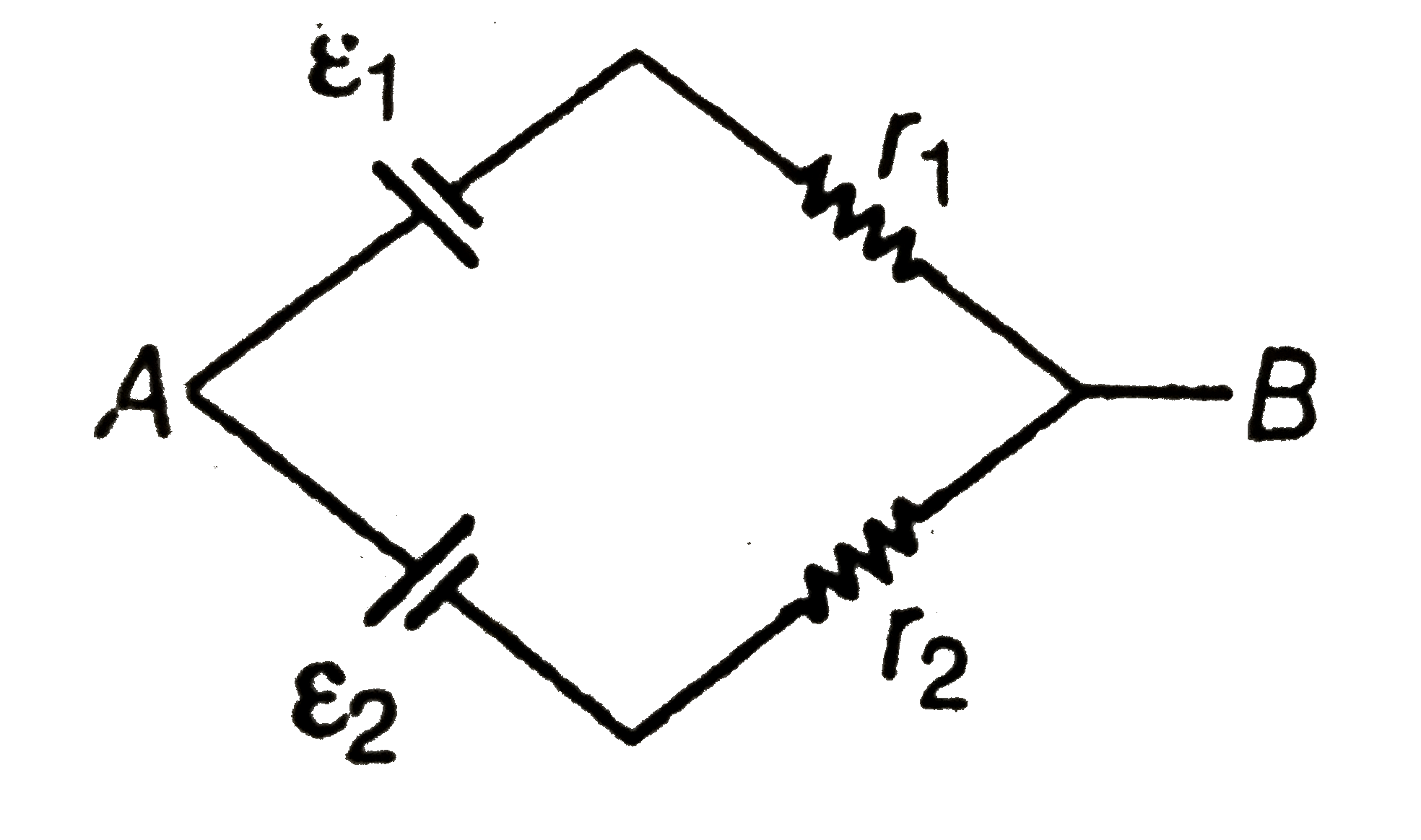
Two batteries of emf ε1andε2(ε2>ε1) and internal resistance r1andr2 respectively are connected in parallel as shown in figure.
View SolutionTwo batteries of emf ε1 and ε2(ε2>ε1) and internal resistances r_1 and r2 respectively are connected in parallel as shown in Fig. Then

View SolutionTwo cells of the same e.mf. e but different internal resistances r1andr2 are connected in series with an external resistance 'R'. The potential drop across the first cell is found to be zero. The external resistance Ris
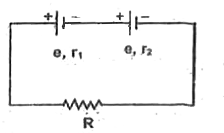
View Solution
