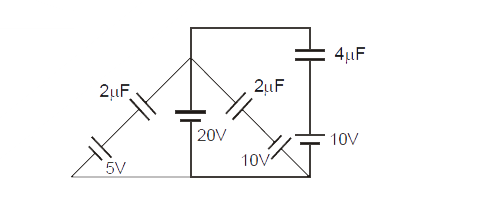
If A is connerted with D and B is connected with C find the potential difference a across each capacitor and the final charge in each capacitor.

Video Solution

Text Solution

Verified by Experts

Let potential of B and c is zero and common potential on capacitors is V, then at A and D it will be V
$2 V + 3 V = 10 \Rightarrow V = 2$ volt
Now charge on each plate is shown in the figure
Heat produced $= 400 + 150 - \frac{1}{2} \times 5 \times 4 = 550 - 10 = 545 μ J$

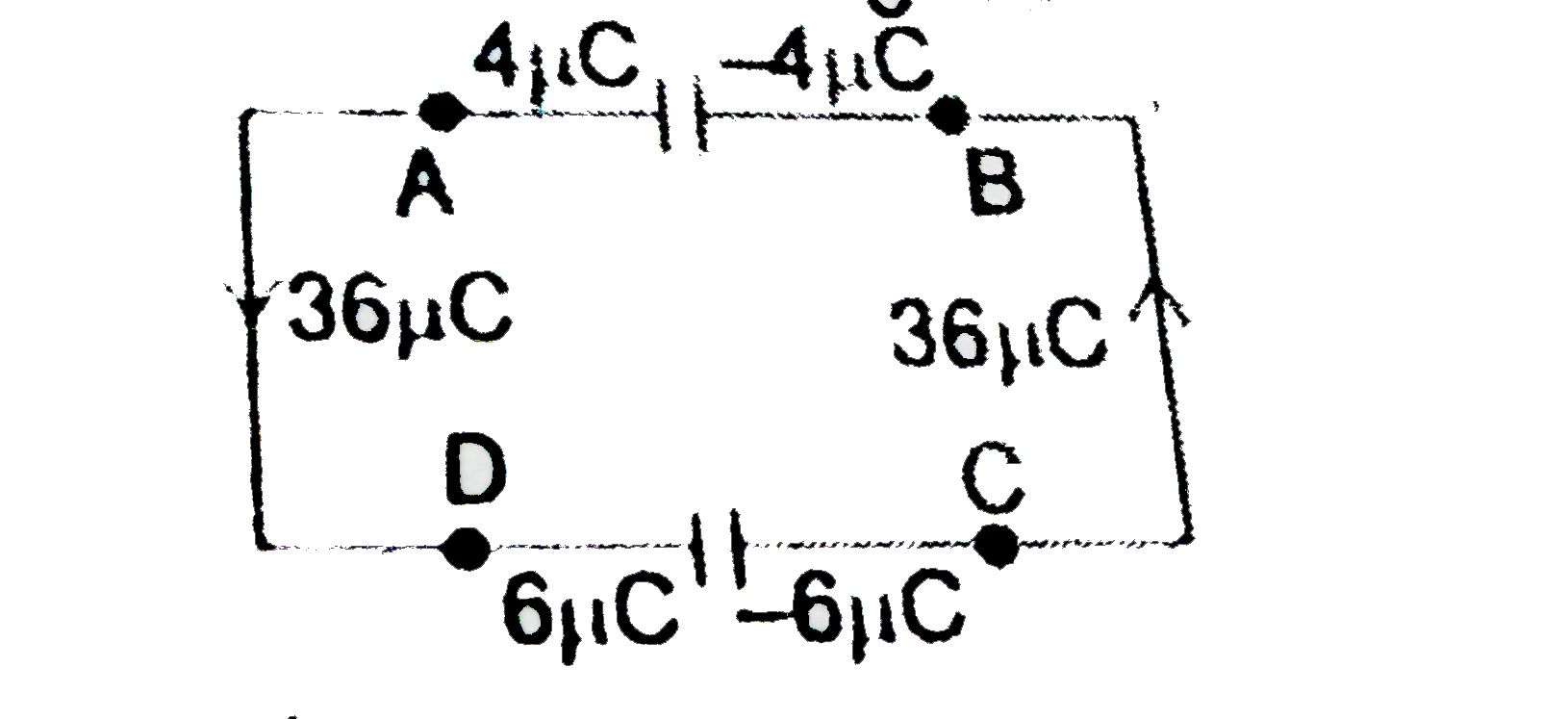
Note : Here heat produced is more. Think why ?

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two capacitors $C_{1}$ and $C_{2}$ are charged separately to potentials $20 V and 10 V$ , respectively. The terminals of capacitors $C_{1} and C_{2}$ are marked as (A-B) and (C-D), respectively. A is connected with $C and B$ is connected with D.
i. Find the final potential difference across each capacitors.
ii. Find the final charge in both capacitors
iii. How much heat is produced in the circuit.
A $128 μ C$ $142 μ C$ $60 u J$
B $28 μ C$ $42 μ C$ $60 u J$
C $28 μ C$ $42 μ C$ $160 u J$
D $2.8 μ C$ $4.2 μ C$ $60 u J$

RESONANCE-CAPACITANCE-High Level Problems

Home
Ask Doubt
Profile