Question

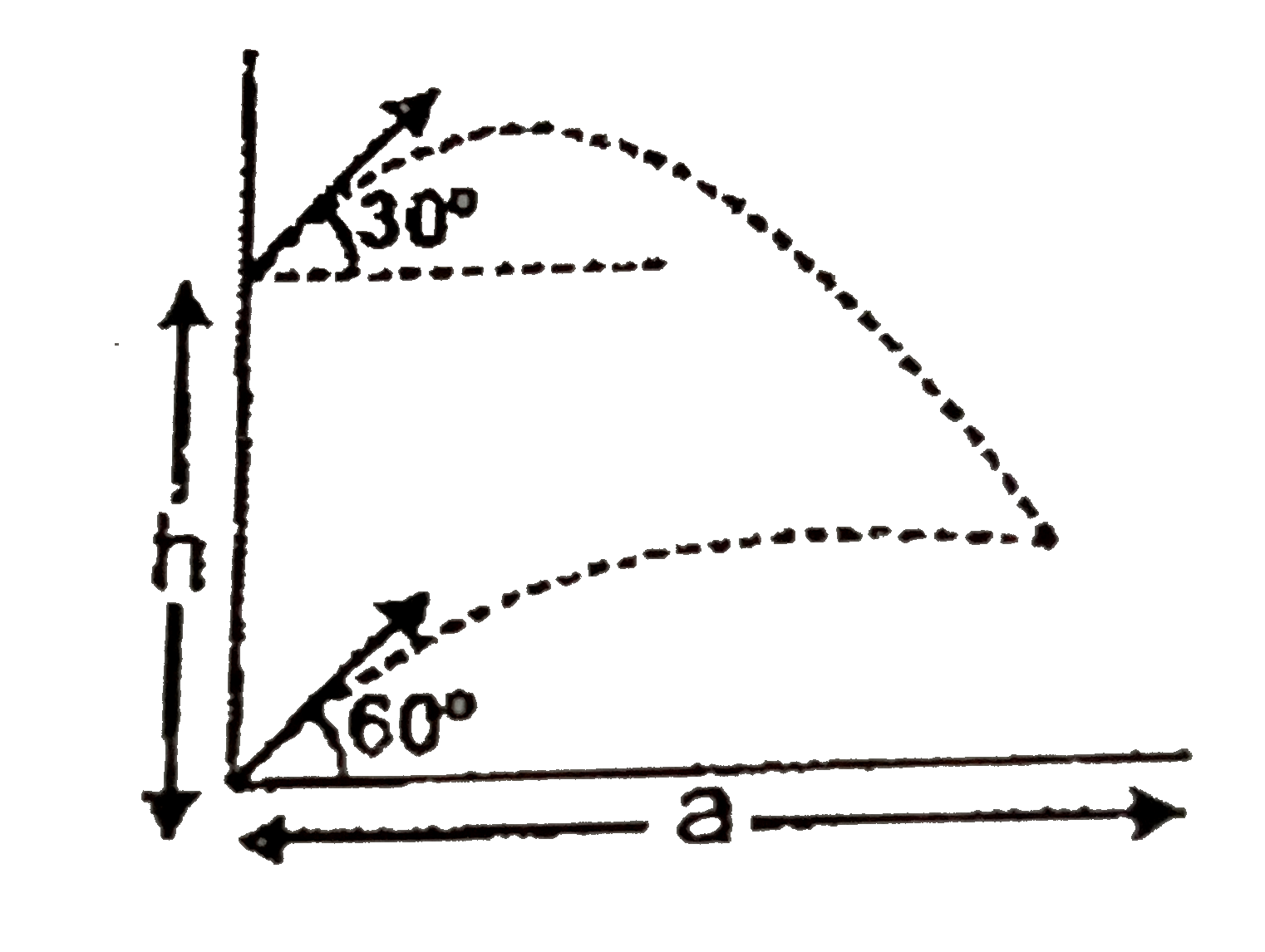
Shots are fired from the top of a tower and from bottom simultaneously at angle ${30}^{\circ }$ and ${60}^{\circ }$ as shown. If horizontal distance of the point of collision is at a distance 'a' from the tower. Then height of tower h is

Options

A. `(2a)/(sqrt(3))`
B. `(a)/(sqrt(3))`
C. 2a
D. `(4a)/(sqrt(3))`

Answer

A

Explanation

For collision to take to place collision of velocity along x-should the same
`therefore " "u_(1)cos 30^(@)" "implies u_(2)cos 60^(@)`
`u_(1)xxsqrt(3)/(2)" "impliesu_(2)xx(1)/(2)" "....(1)`
Relative velocity along y-direction w.r.t upper ball-1
`u implies (u_(2)sin60^(@)-u_(1)sin30^(@))`
a = 0
s implies +h
`t implies (h) /(u_(2)(sqrt(3))/(2)-u_(1)(1)/(2))implies (2h)/(sqrt(3)u_(2)-u_(1))`
time should be same for both direction.
`(a)/(u_(2)cos 60^(@))=(2h)/(sqrt(3)u_(2)-(u_(2))/(sqrt(3)))`
Solving this
`2a implies (2hsqrt(3))/(2)`
`h implies (2a)/(sqrt(3))`