A particle is projected from a point O with a velocity u at an angle α (upwards) to the horizontal. At a certain point P it moves at right angles to its initial direction. It follows that:
OP makes an angle tan−1(u/2g) to the horizontal
the distance of P from O is u/(2gsinα).
the time of flight from O to P is u/(gsinα)
the velocity of the particle at P is u cot α
The correct Answer is:C, D
Taking initial and final velocity on x and y axis.
0 = u - g sin αt
t=ugsin2
x=u[ugsin2]−12(gsinα)[u(gsin2)]2=
u22gsinα
y=12gcosa[ugsinα]2=u22g(cosαsin2α)
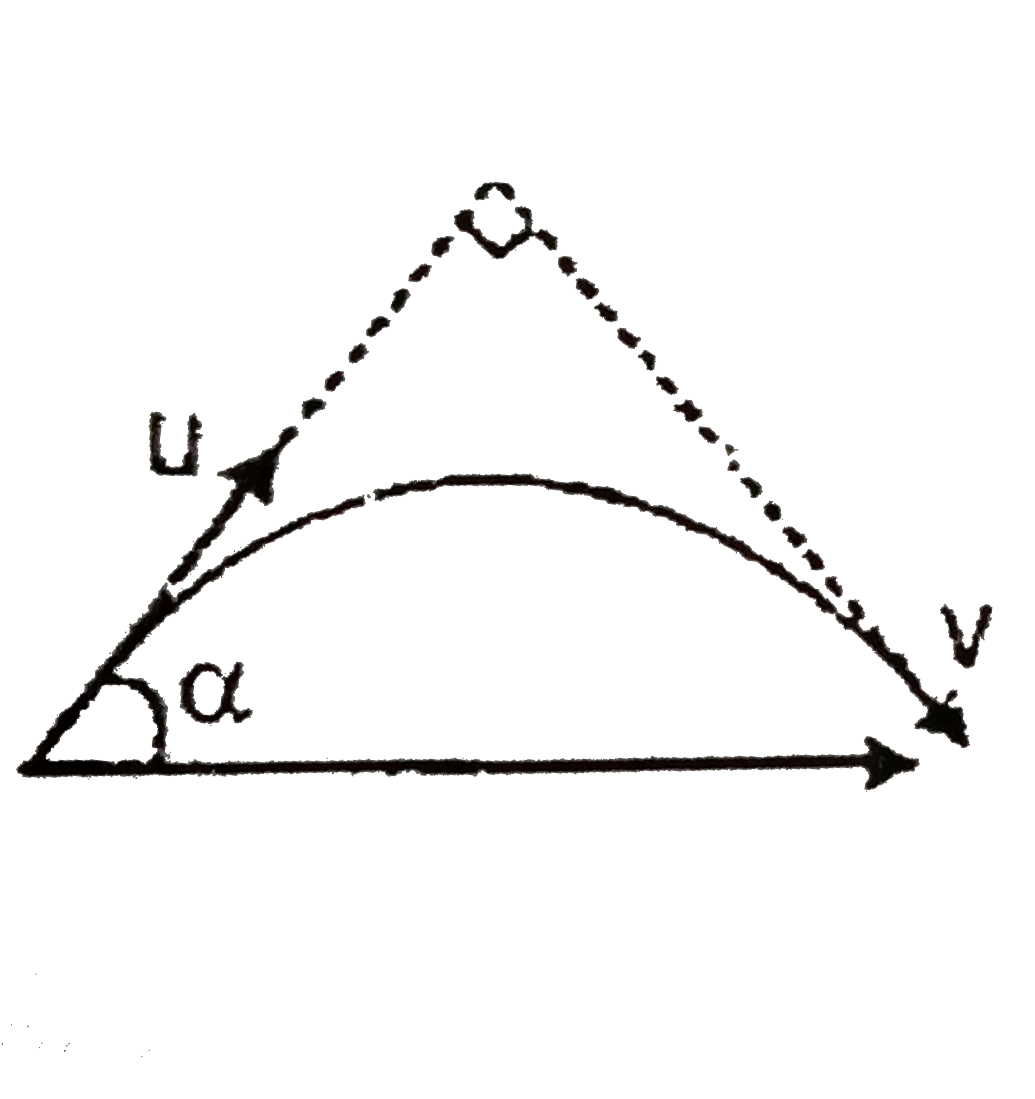
OP = √x2+y2=u22gsinα
tanϕ=yx=cosαxsinαsin2α=cotα
v=0+gcosa[ugsinα]=ucotα
Topper's Solved these Questions
Similar Questions
A particle is projected form ground with velocity u ar angle θ from horizontal. Match the following two columns.

View SolutionA ball is projected with initial speed u at an angle θ above the horizontal . Calculate the time after which ball will be moving at right angle to its initial velocity.
View Solution
Knowledge Check
A particle is projected from a point P with a velocity v at an angle θ with horizontal. At a certain point Q it moves at right angles to its initial direction. Then
AVelocity of particle at Q is vsinθBVelocity of particle at Q is vcotθCTime of flight from P to Q is (v/g)cosecθDTime of flight from P to Q is (v/g)secθA particle is project d from point A with velocity u at an angle α with horizontal. In its parabolic path, at point P the particle is moving at right angles to its initial direction of projection. Its velocity at P is:
AutanαBucotαCucosαDucosecαA particle is projected with initial velocity u making an angle α with the horizontal, its time of flight will be given by
A2usinαgB2u2sinαgCusinαgDu2sinαg
A particle is projected with a velocity of 39.2m/sec at an angle of 30∘ to the horizontal. It will move at right angles to the direction of projection after the time
View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. At any instant its velocity becomes v which is perpendicular to the initial velocity u. Then v is
View SolutionA particle is projected from a point A with velocity u√2 at an angle of 45∘ with horizontal as shown in the figure. It strikes the plane BC at right angles. The velocity of the particle at the time of collision is
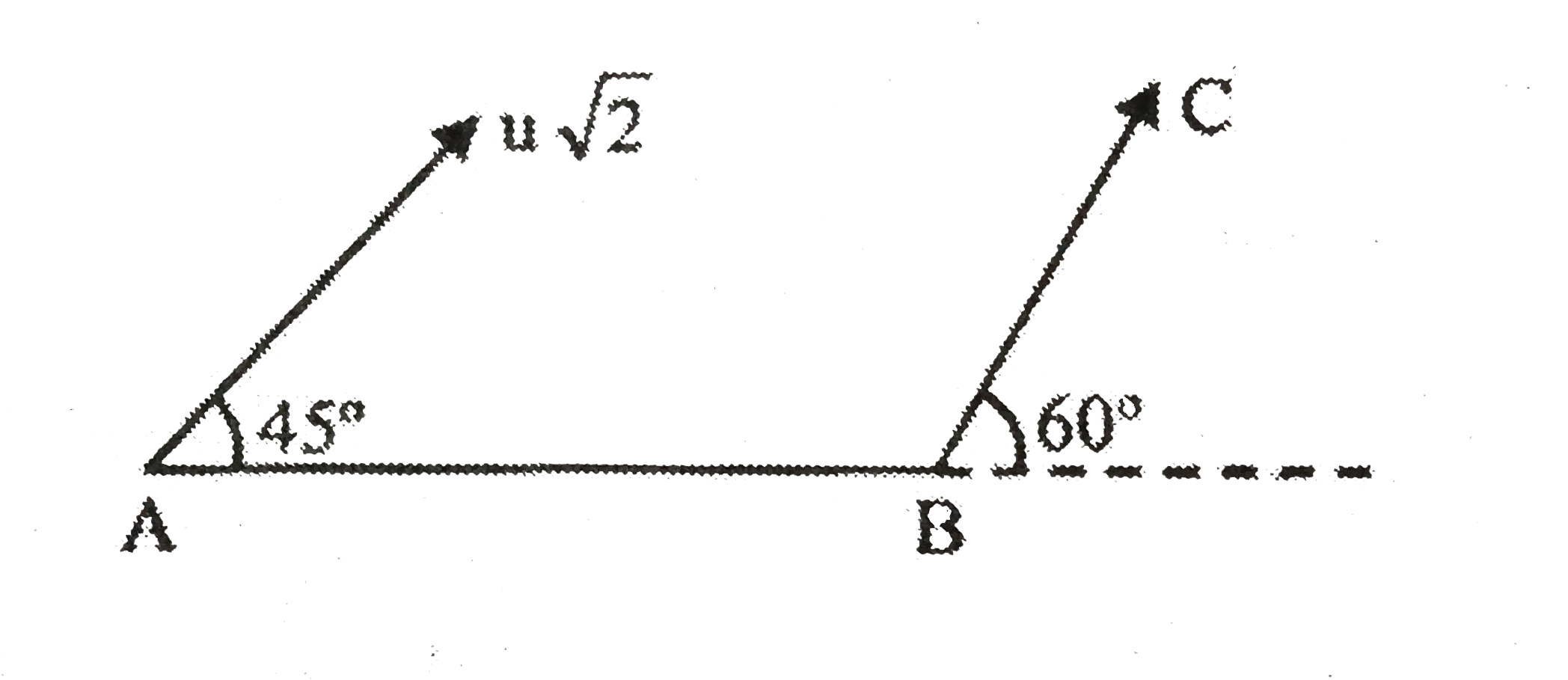
View SolutionTwo particle are projected with same initial velocities at an angle 30∘ and 60∘ with the horizontal .Then
View SolutionA particle is projected from a point A with velocity √2u an angle of 45∘ with horizontal as shown in figure. It strikes the plane BC at right angles. The velocity of the particle at the time of collision is

View Solution
