Derive an experssion for the average power in LCR circuit connected to a.c. supply. Hence define power factor. Show that average power cousumed per cycle in an a.c. circuit conataining an ideal capacitor is zero.
Video Solution
Text Solution
Power in LCR circuit /AC circuit: the voltage V=Vmsinωt is applied to a series RLC circuit.Then the current in the circuit is given by I=Imsin(ωt+ϕ)
where Im=VmZ and ϕ=tan−1(XL−XCR)
The instaneous power P supplied to the source is:
P=VI
P=Vmsinωt.Imsin(ωt+ϕ)
=VmIm2.2sinωtsin(ωt+ϕ)
=VmIm2[cos(ωt−ωt−ϕ)]−cos(ωt+ωt+ϕ)
[∵2sinAsinB=cos(A−B)−cos(A+B)]
=VmIm2[cosϕ−cos(2ωt+ϕ)]...(i)
∵ The average power over a cycle is given by the average of the two terms in RHS of equation (i).The second terms in RHS is time dependent.Its average is zero
[∵ Positive half of the cosine cancles the negative half]
Therefore, average power
¯¯¯P=VmIm2cosϕ=Vm√2Im√2cosϕ
¯¯¯P=VrmsIrmscosϕ
Where cosϕ is called power factor.It depends on voltage, current and phase angle ϕ CaseI:For resistive circuit:If circuit contains only resistance R
∴ϕ=0, Therefore
P=VrmsIrmscos0=VrmsIrms
Therefore, power disispation is maximum
Case II: For pure inductive or capacitive circuit, the phase difference between voltage and current is π2
∴ϕ=π2⇒cosϕ=cosπ2=0
∴¯¯¯P=VrmsIrmscosϕ=0
Therefore, power dissipation is zero. hence, no power is dissipated even through a current is flowing in the circuit, the current is called wattless current.
|
Topper's Solved these Questions
- ALTERNATING CURRENTBook:RESONANCEChapter:ALTERNATING CURRENTExercise:A.L.P
Explore 10 Videos
- ALTERNATING CURRENTBook:RESONANCEChapter:ALTERNATING CURRENTExercise:SOLVED MISCELLANEOUS
Explore 14 Videos
- ALTERNATING CURRENTBook:RESONANCEChapter:ALTERNATING CURRENTExercise:Exercise -3 Part-2
Explore 3 Videos
- ATOMIC PHYSICSBook:RESONANCEChapter:ATOMIC PHYSICSExercise:Advanved level problems
Explore 17 Videos
Similar Questions
Knowledge Check
The average power in LCR series circuit is
AermsIrmscosϕBermsIrmssinϕCermsIrmsDermsIrmstanϕThe average power dissipated in an AC circuit containing a resistance along is
AermsIrmsBermsIrmscosϕC0Dnone of theseAverage power lost per cycle of A.C. is given by
A12e0I0sinθB12e0I0cosθC12e0I0tanθD12e0I0×θ
The average power in LCR series circuit is
View SolutionHow much average power, over a complete cycle, does an a.c. source supply to a capacitor ?
View SolutionIn the given A.C. circuit the average power consumed in the circuit is
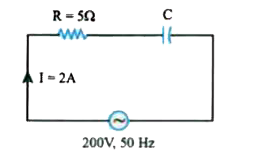
View SolutionPower consumed in an AC circuit becomes zero if
View SolutionThe average power dissipated in A.C. circuit containing resistance, inductance and capacitance depends upon
View Solution