A coil ABD of N turns & radius R carries a current of 1Amp & is placed on a horizontal tabel.K is a very small horizontal conducting ring of radius r placed at a distance Y0 from the centre of the coil vertically above the coil ACD.Find an expression for the EMF established when the ring K is allowed to fall freely.Express the EMF in terms of instanteous speed v& height Y.
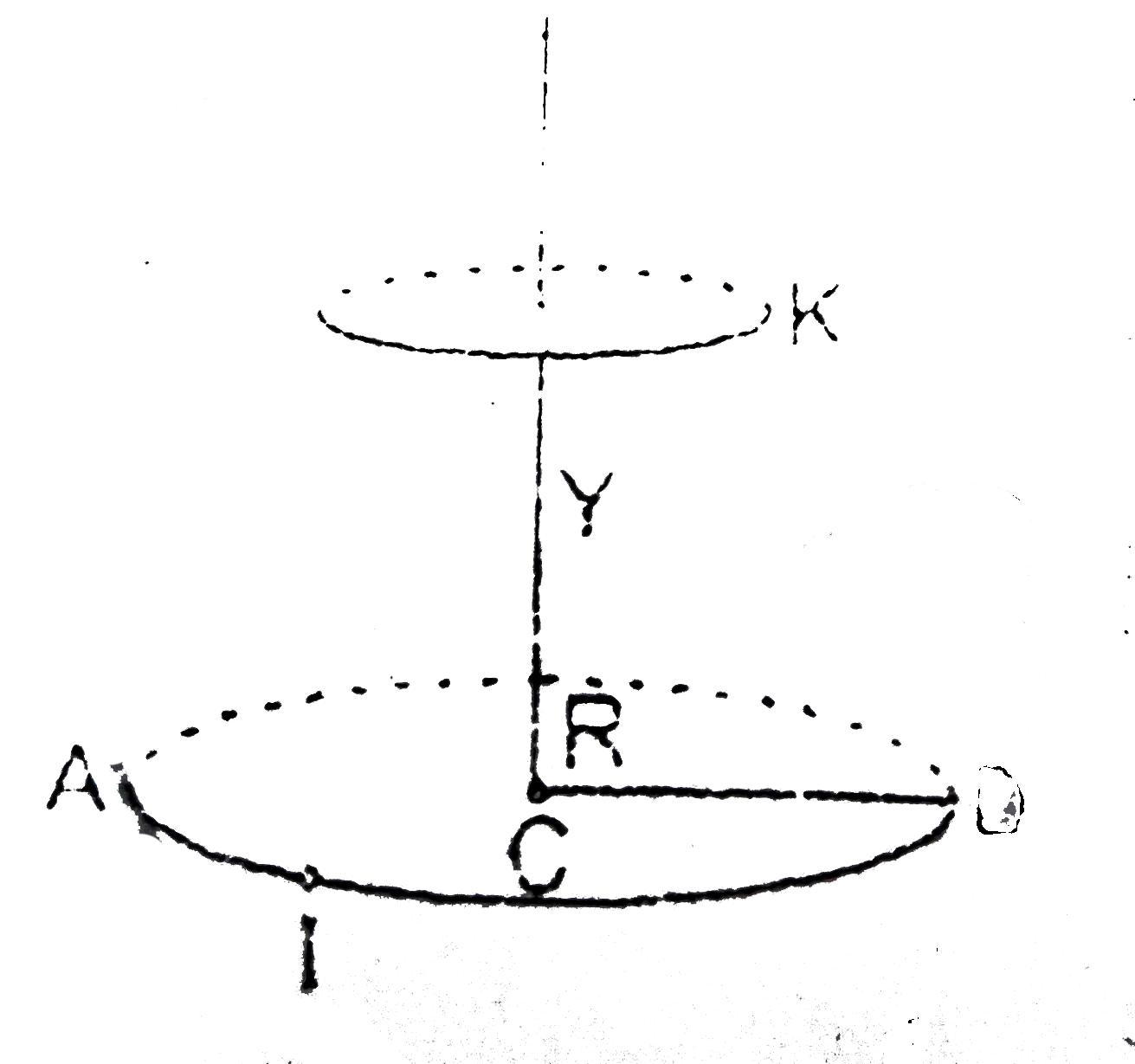
The correct Answer is:B, C
B=μ0IR2N2[R2+y2]3/2
ε=−dϕdt=−AdBdt=−πr2dBdt...(1)
here dBdt=−μ0IR2N2×321[R2+y2]5/2×2y×dydt
=−3μ0IR2N2yv[R2+y2]5/2 put this value in eq. (i) and get Ans.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-1 PART 2
51 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 1
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problem
12 Videos
- ELECTRODYNAMICSBOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP
39 Videos
Similar Questions
A coil A of radius R and number of turns n carries a current i and it is placed in a horizontal plane. A small conducting ring P of radius r(r<<R) is placed at a height y0 above the centre of the coil A as shown in figure-5.38. Calculate the induced EMF in the ring when the ring in allowed to fall freely. Express the induced EMF as a function of instantaneous speed of the falling ring and its height above the center of the coil A.
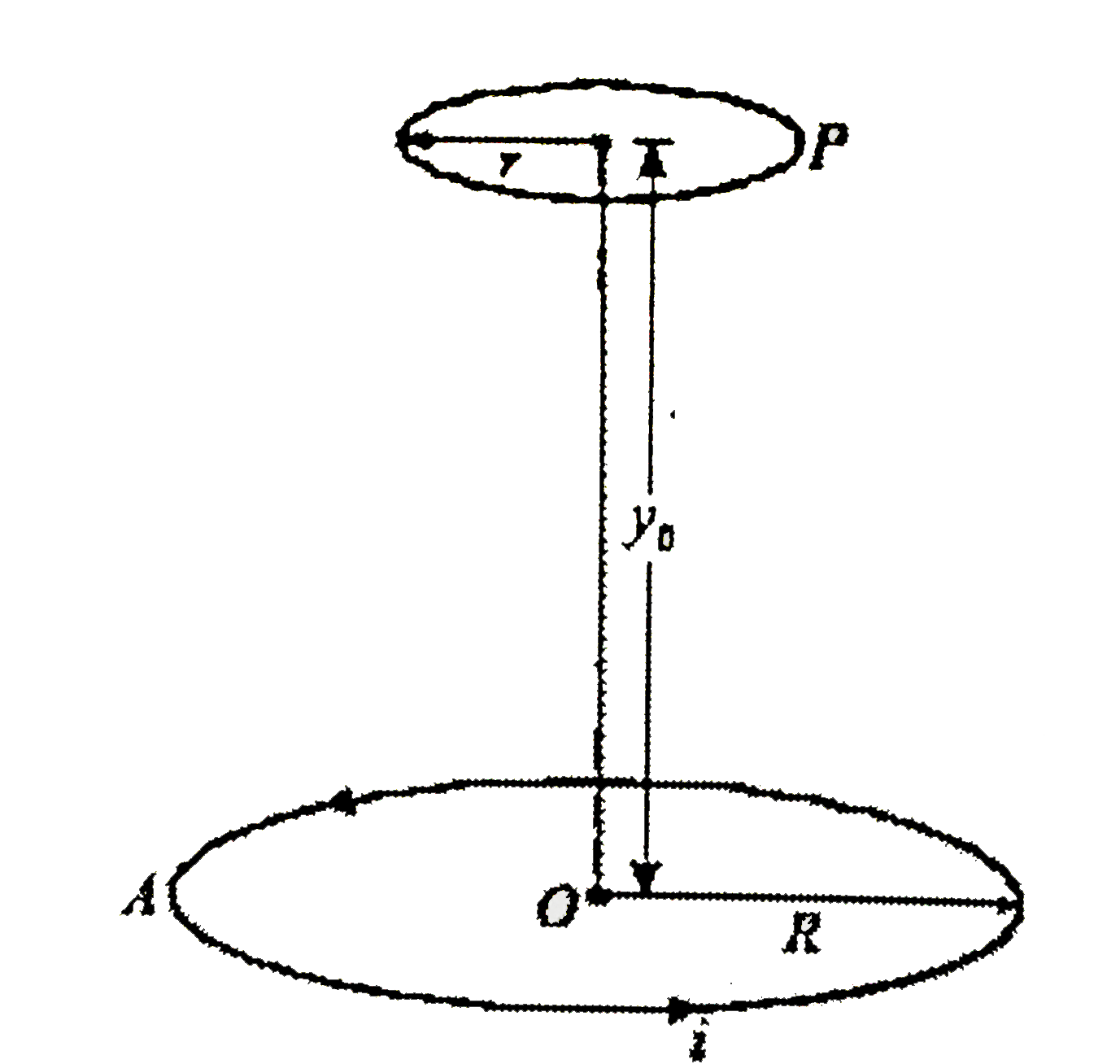
View SolutionA horizontal flat coil of radius a made of w turns of wire carrying a current sets up a magnetic field. A horizontal conducting ring of radius r is placed at a distance x0 from the centre of the coil (Fig. 30.6). The ring is dropped. What e.m.f. will be established in it? Express the e.m.f. in terms of the speed.
View Solution
Knowledge Check
A circular coil of n turns and radius r carries a current I. The magnetic field at the center is
Aμ0nIrBμ0nI2rC2μ0nIrDμ0nI4rThe ratio of the magnetic field at the centre of a current carrying coil of the radius a and at distance 'a' from centre of the coil and perpendicular to the axis of coil is
A1√2B√2C12√2D2√2A circular coil of radius R carries an electric current. The magnetic field due to the coil at a point on the axis of the coil located at a distance r from the centre of the coil, such that r>>R, varies as
A1rB1r3/2C1r2D1r3
A circular coil of 100 turns has a radius of 10 cm and carries a current of 5A. Calculate the magnetic field (a) at the centre of the coil (b) at a point on the axis of the coil at a distanceof 5cm from the centre of the coil.
View SolutionA circular coil of 120 turns has a radius of 18cm and carries a current of 3⋅0A. What is the magnitude of the magnetic field (i) at the centre of the coil (ii) at a point on the axis of the coil at a distance from the centre equal to the radius of the coil?
View SolutionShow that the magnetic field along the axis of a current carrying coil of radius r at a distance x from the centre of the coil is smaller by the fraction 3x2/2r2 than the field at the centre of the coil carrying current.
View SolutionA circular coil of radius R carries an electric current. The magnetic field due to the coil at a point on the axis of the coil located at a distance r from the centre of the coil, such that r>>R, varies as
View SolutionA circular coil of radius R carriers an electric current. The magnetic field due to the coil at a point on the axis of the coil located at a distance r from the centre of the coil, such that r>>R, varries as
View Solution
