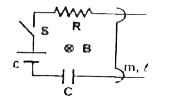
Figure showns a smooth pair of thick metallic rails connected across a battery of having a negligible internal resistance.A wire of length and resistance can slide smoothly on the rails.The entire system lies in a horizontal plane and is immersed in a uniform vertical magnetic field .At an instant the wire at this instant. (b)What is the force acting on the wire at this instant. (c)Show that after some time the wire will slide with a constant velocity.Find this velocity.
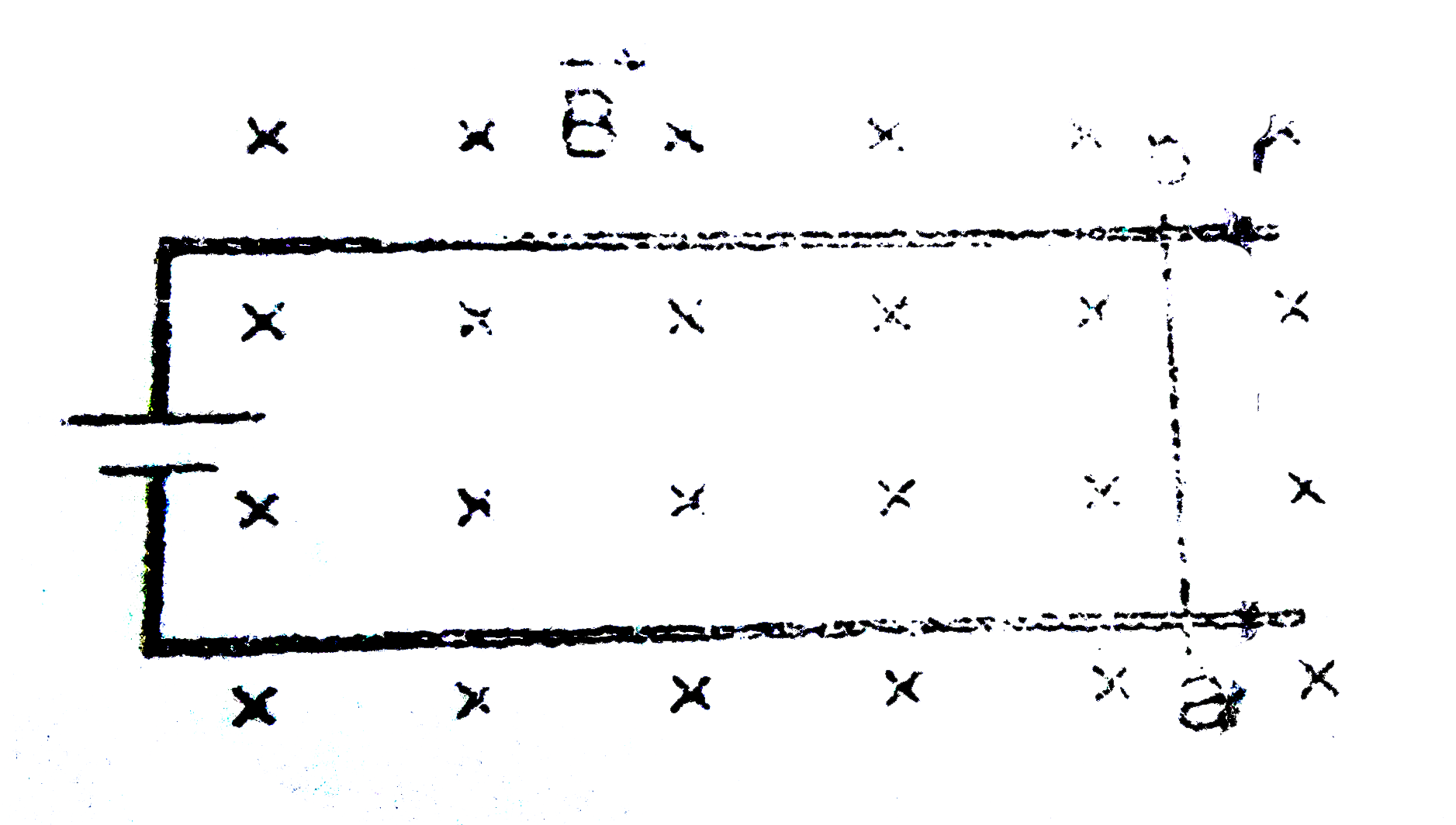
The correct Answer is:A, B, C, D
(a) clockwise
(b)
( c) is towards right so will keep on increasing after some time current will not flow in circuit then it will move with constant velocity . will be maximum (or constant) when .
So

Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-1 PART 2
51 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 1
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problem
12 Videos
- ELECTRODYNAMICSBOOK - RESONANCECHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems
31 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP
39 Videos
Similar Questions
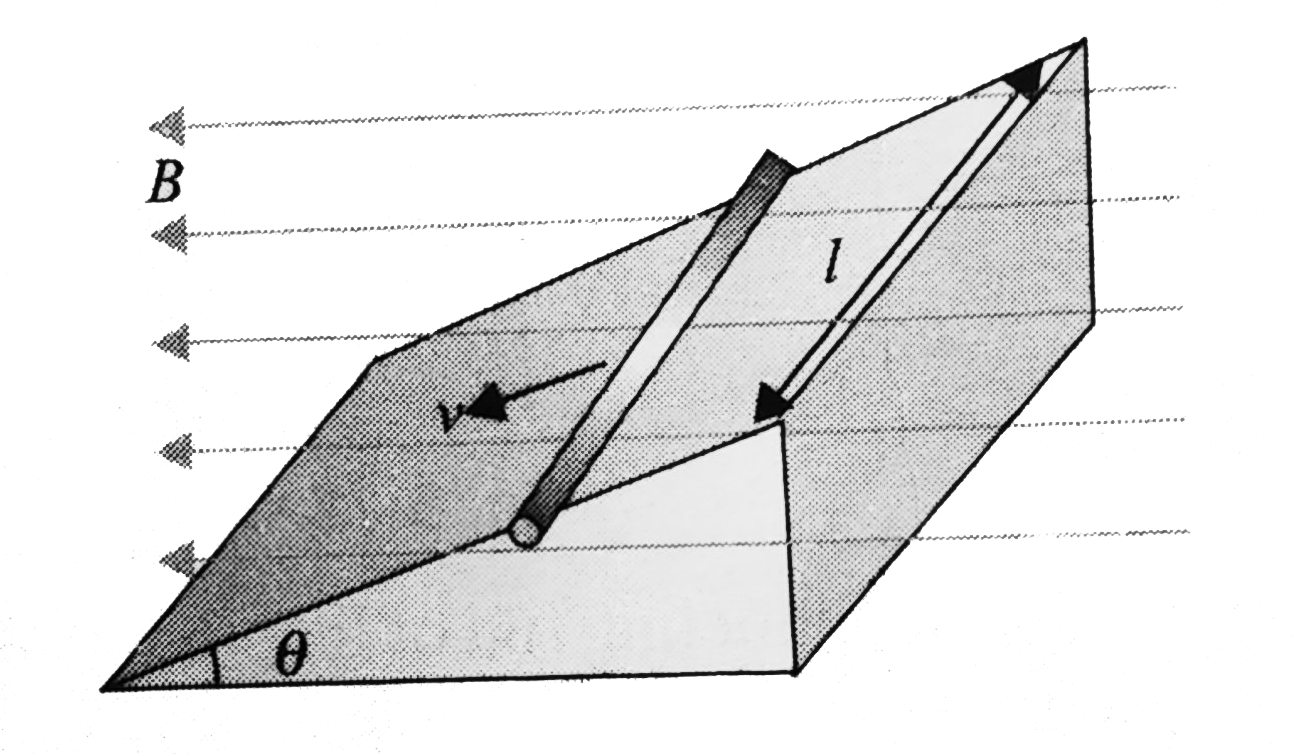
A rod of mass m, length l and resistance R is sliding down on a smooth inclined parallel rails with a cinstant velocity v. If a uniform horizontal magnetic field B exists, then find thevalue of B.
View SolutionA wire of resistance 4Ω is connected to a battery having an internal resistance of 1Ω For a current of 6 A to flow through the circuit what should be the emf of the battery ?
View Solution
Knowledge Check
A wire ab of length l, mass m and resistance R slides on a smooth thick pair of metallic rails joined at the bottom as shown in fig. The plane of the rails makes an angle θ with the horizontal. A vertical magnetic field B exist in the region. If the wire slides on the rails at a constant speed v, then the value of B is -
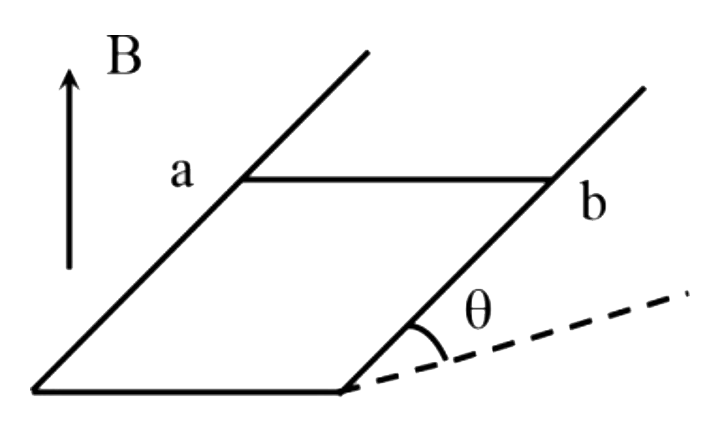 A√mgRvl2cos2θB√mgRcosθvl2sin2θC√mgRv2l2sin2θD√mgRsinθvl2cos2θ
A√mgRvl2cos2θB√mgRcosθvl2sin2θC√mgRv2l2sin2θD√mgRsinθvl2cos2θA conducting wire of length l and mass m can slide without friction on two parallel rails and is connected to capacitance C. Whole system lies in a magnetic field B and a constant force F is applied to the rod. Then
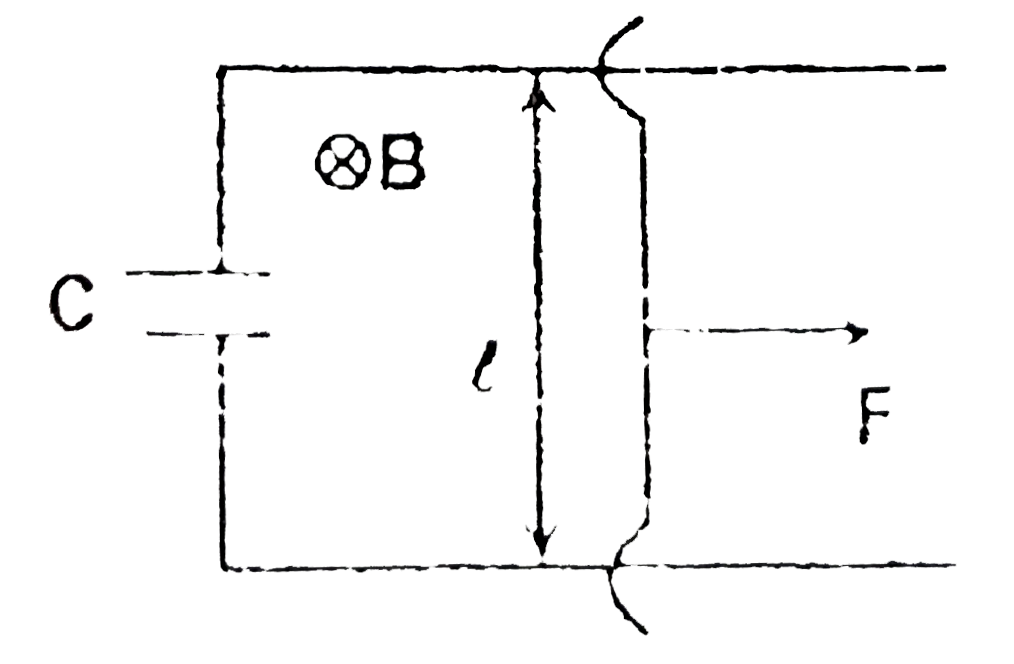 Athe rod moves with constant velocityBthe rod moves with an acceleration of Fm+B2l2cCthere is constant charge on the capacitor.Dcharge on the capacitor increases with time
Athe rod moves with constant velocityBthe rod moves with an acceleration of Fm+B2l2cCthere is constant charge on the capacitor.Dcharge on the capacitor increases with timeIn the network shown, the connector of mass m, length l and negligible resistance can smoothly side along two fixed parallel rails. The capacitor is initially uncharged and ET a uniform magnetic field B exists in the region directed into the plane of paper. If the switch S is closed at t=0 then the charge on the capacitor after long time is
 Amcε2(B2l2c+m)Bmcε(B2l2c+m)C2mcε(B2l2c+m)D3mcε2(B2l2c+m)
Amcε2(B2l2c+m)Bmcε(B2l2c+m)C2mcε(B2l2c+m)D3mcε2(B2l2c+m)
A uniform magnetic field →B=B0ˆk exists in a region. A current carrying wire is placed in x-y plane as show. Find the force acting on the wire AB, if each section of the wire is of length 'a'.
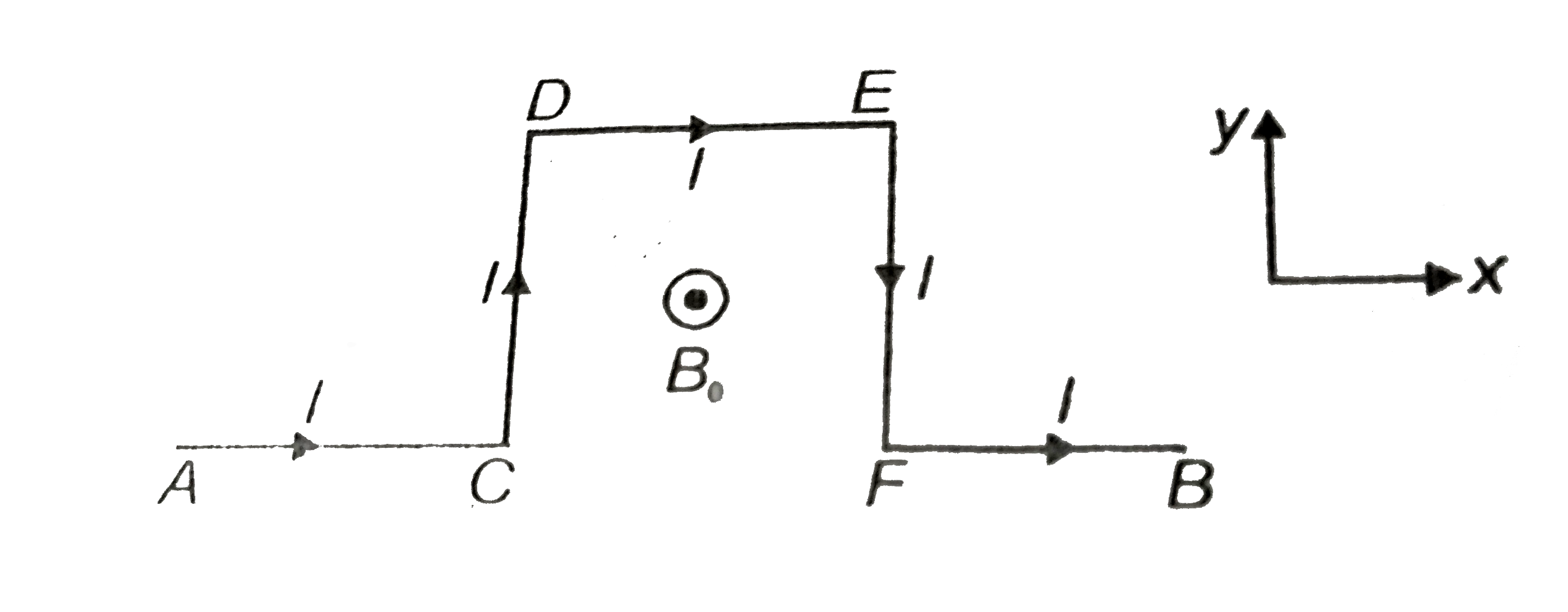
View SolutionIn Fig. shown, the rod has a resistance R, the horizontal rails have negligible friction. A battery of emf E and negligible internal resistance is connected between points aandb. The rod is released from rest.

The current when the rod attains its terminal speed is
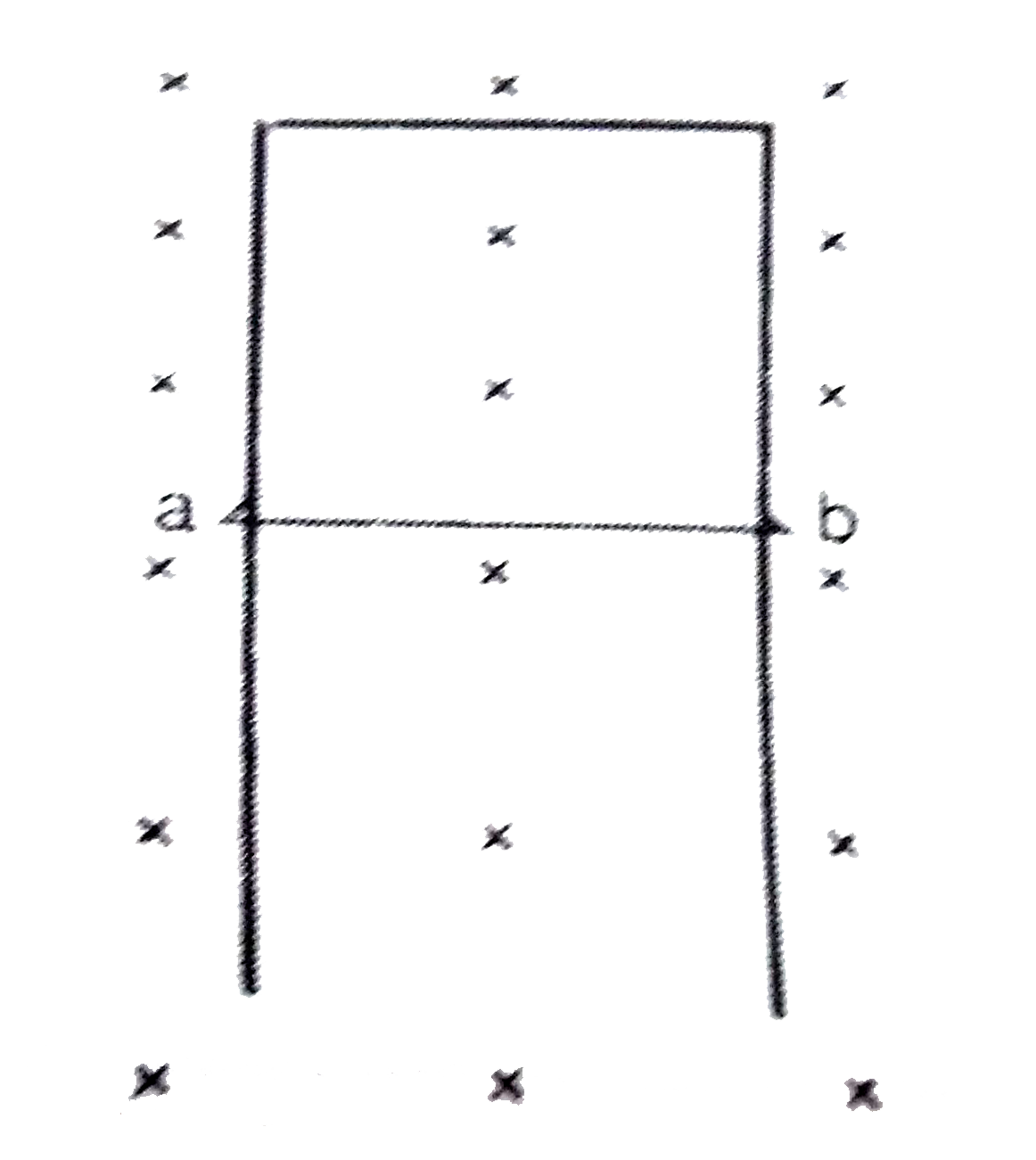
View SolutionA copper wire ab of length l, resistance r and mass m starts sliding at t=0 down a smooth, vertical, thick pair of connected condcuting rails as shown in figure.A uniform magnetic field B exists in the space in a direction perpendicular to the plane of the rails which options are correct.
View SolutionA copper wire ab of length l, resistance r and mass m start sliding at t=0 down a smooth, vertical, thick pair of connected conducting rails as shown in figure. A uniform magnetic field B exists in the space in a direction perpendicular to the plane of the rails which options are correct .
View SolutionA conducting wire of length l and mass m can slide without friction on two parallel rails and is connected to capacitance C. The whole system lies in a magnetic field B and a constant force F is applied to the rod. Then
View Solution
